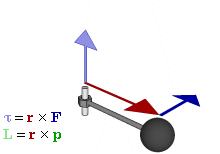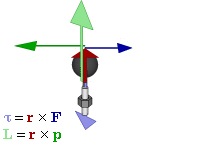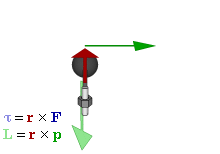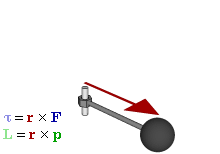
L, angular momentum, is not like linear momentum ($p$) that means that the object is actually moving in that direction. Angular momentum and torques are defined via the "cross-product", which means mathematically that angular momentum and torque vectors point 90$^\circ$ from the radius and force that produce the torque and angular momentum. The radius is the red arrow in the animation while the force is the dark green arrow in the diagram. That's why the angular momentum vector is pointing up and down in the animation; however, that doesn't translate to actual linear motion in that direction. It instead represents a direction that the object is spinning, using the right hand rule. If you take your right hand and point your thumb in the direction of the angular momentum vector and curl your fingers, the direction that your fingers point is the direction in which the object will be spinning. To repeat, mathematically the angular momentum vector represents the direction and speed with which an object is spinning (which direction can be found via the right-hand rule).
For a less mathematical explanation, if what you claim is true (that rotating things counter-clockwise will cause them to rise) then I think it would be difficult to keep tops on the table, since they are able to spin pretty fast and would be able to levitate. I have never seen this happen, nor have I seen a situation where spinning a top increased or decreased it's weight (based on the direction it's spinning). However, spinning the top (while not allowing it to fly) does increase its stability by making it precess under the force of gravity rather than topple (if it is spinning fast enough). This is angular momentum and torque at work in a real situation.