Topple a mass standing still

Question

Consider the following situation:

This is taken from an example from my book with a solution.

The box weight is $W$ and the coefficient of friction between the floor and the object is $\mu$.

What is the minimal magnitude of the horizontal force $F$ so that the box would topple?

The answer in the book states this:

We get the 2 following: $$F_{friction}=F \\N=W$$ Now find $x$ by moments: $$ \text{(1) } x\cdot N=x\cdot W=\frac{b}{2}\cdot W-h\cdot F$$ so that $$x=\frac{b}{2}-h\cdot \frac{h}{W}$$ and now equate $x$ to zero and find $F$: $$F_{min}=\frac{b}{2h}\cdot W .\text { Answer.}$$ I didn't understand the whole process written in $\text{(1)}$ at which most of the question is solved.

EDIT: This $F_{min}$ is correct for most geometric bodies i.e cone, cylinder, prism and so.

Answer 1

I start from this Free Body Diagram, with the block contacting the ground on the edges. The normal forces at the contacts are $N_1$ and $N_2$.

And state that forces obey $W = N_1 + N_2$ and moments about the contact point on the right are $$ \left. \frac{b}{2} W -h F - N_2 b = 0 \right\} N_2 (F) = \frac{W}{2} - \frac{h}{b} F $$

The block will tip if $N_2(F) \le 0$ or

$$ F \ge \frac{b}{2 h} W $$

Answer 2

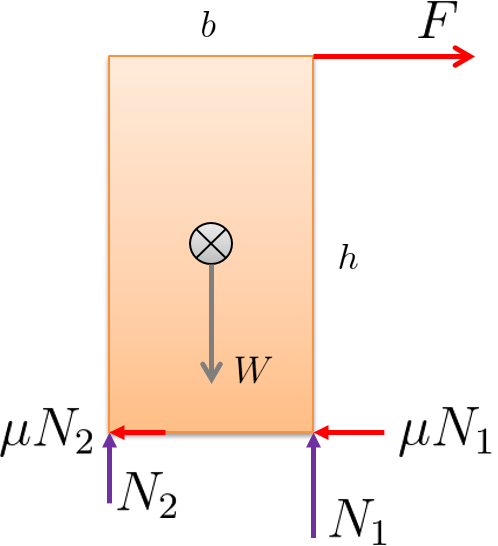
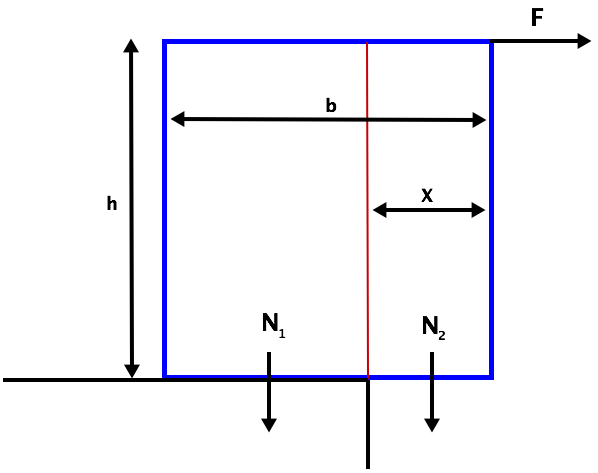
I feel a need to add a "simple" answer, because the book is making this needlessly confusing for you.

Given an object with width b and height h, with weight W, we make the following assumptions:

1. Center of mass is at the center of the object
2. Coefficient of friction $\mu$ is sufficient to prevent sliding

Now we can draw the diagram at the moment that the object topples (that is, when the object is supported entirely at the corner, and the net torque is zero):

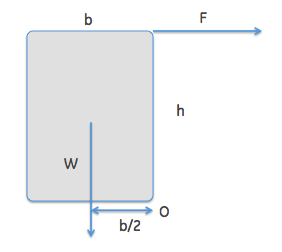
I did not show the reaction forces at $O$ which are the force of friction (horizontal, magnitude $F$) and the normal force (vertical, magnitude $W$): they are of course necessary to talk of "torque" but they make the diagram more complicated. I hope you can forgive me...

Torques about point $O$ are zero when

$$F\cdot h - W\frac{b}{2}=0\\ F = \frac{Wb}{2h}$$

Note that we have to assume that $\mu W > F$ in order for this to occur at all.

The thing that makes the question more complicated than necessary (and why you are struggling to understand it) is the addition of a distance $x$ (which I deliberately did not show in my diagram). This is useful when you want to figure out the apparent point of equilibrium of the object - basically, if you start pushing on the object, there will be a shift in the apparent location where the normal force acts on the interface between the object and the support below it. Solving for this apparent location (setting it to zero - i.e. acting on the corner) is what the book's solution did; I consider that a confusing way to introduce a simple subject.

One interesting aside: if the surface properties were not constant (for example, if the right hand side was ice while the left is concrete) then this concept of "apparent location of normal force" starts to make sense, and you could see that the object starts to slide when a certain lateral force is applied (the normal force on the concrete would become less, and if we assume the ice is frictionless the additional normal force on that side would not prevent sliding). So I'm not saying that the approach taken here is completely useless - just that I would prefer to start simpler...

Answer 3

The necessary requirement for the box to topple would be for the drag torque to exceed the torque provided by the normal force at the corner of the box.

Since the drag torque at the corner is given by $hF$ and the drag torque is $\frac{b}{2}N$ then the requirement is $hF > \frac{b}{2}N$. The exact point of the transition is when $\frac{b}{2}N - hF_{min} = 0$.

The introduction of the parameter $x$ is unnecessary to solve the problem as it is stated.

Generalizing the problem somewhat to allow the box to stand at the edge of a table so that it can topple around another point than the corner makes it relevant. Consider the situation sketched here:

The introduction of the edge of the table makes it necessary to consider two forces, each at the center of their respective part of the block. These can be expressed as $N_1 = \frac{b-x}{b}N$ and $N_2 = \frac{x}{b}N$ such that $N = N_1+N_2$.

The box topples when $\frac{b-x}{2}N_1 - \frac{x}{2}N_2 - hF < 0$ and, plugging in the expressions for $N_1$ and $N_2$, you arrive at the general condition $\frac{b}{2}N - hF < xN$ where the transitioning point is at $\frac{b}{2}N - hF_{min} = xN$. This is your expression (1).