I start from this Free Body Diagram, with the block contacting the ground on the edges. The normal forces at the contacts are $N_1$ and $N_2$.
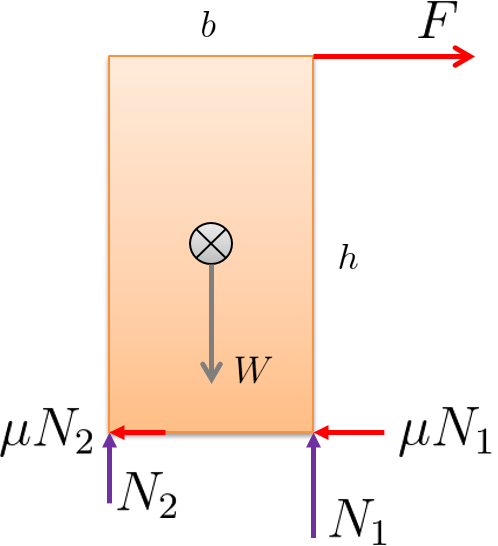
And state that forces obey $W = N_1 + N_2$ and moments about the contact point on the right are $$ \frac{b}{2} W -h F - N_2 b = 0 $$$$ \left. \frac{b}{2} W -h F - N_2 b = 0 \right\} N_2 (F) = \frac{W}{2} - \frac{h}{b} F $$
The block will tip if $N_2 \le 0$$N_2(F) \le 0$ or
$$ F \le \frac{b}{2 h} W $$$$ F \ge \frac{b}{2 h} W $$
