The title is the question. Here's why it seems like local kinetic energy should increase:
Numerous questions and answers here and elsewhere suggest that the reason the metric expansion of space is not observable locally (even on a galactic scale) is that local forces maintain the metric distance between near objects, with "near" meaning anything from two subatomic particles to two celestial bodies.
My mental picture is of ants on an expanding balloon. The ants get farther apart, but the expansion of the balloon beneath their feet is not going to tear the individual ants apart. They do however have to do a little dance to keep from doing the splits.
It seems to me that this should imply a continuously increasing kinetic energy, presumably generalized as random motion -- i.e. things get hotter.
Here is a simple illustration. A and B could be particles attracted by the electromagnetic force, or whole planets attracted by gravity:
A begin state with an arbitrary distance and energy level:
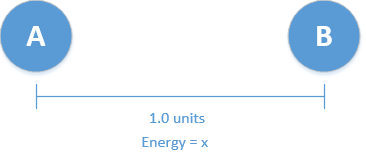
A little bit later, the two objects are farther apart, implying an increase in potential energy:
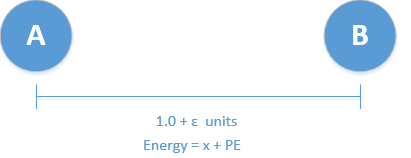
But the force at work brings the objects back to their original
positionsdistance, implying the potential energy was converted to (kinetic?) energy through work W:
Obviously A and B don't actually wiggle apart and then back together, rather the force between them acts constantly as the metric expands constantly, implying a smooth increase in kinetic (or some other type) energy while the measurable distance between the objects stays the same.
Scenarios
- Gravity at planetary distances: Jerry Schirmer's answer suggests the effect would be too small to measure
- What about at subatomic scales? The color force between quarks in a nucleon is a distance-dependent force whose potential energy is orders of magnitude greater than gravity. Should metric expansion not cause an increase in energy of a individual nucleon, detectable as a few extra photons emitted over the course of some time?
