3
$\begingroup$

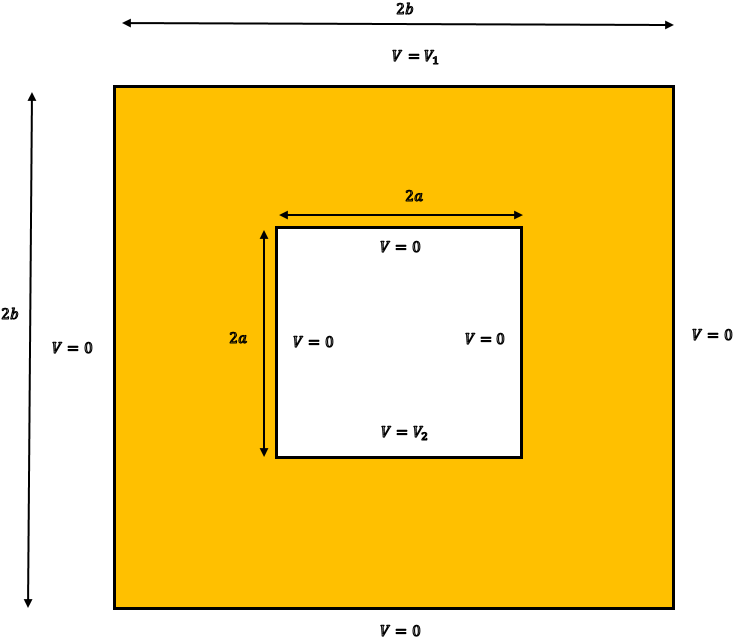
Suppose the values of $a$, $b$, $V_1$ and $V_2$ is given. I want to find the solution of the Laplace equation, $$\frac{\partial^2 \phi}{\partial x^2}+\frac{\partial^2 \phi}{\partial y^2}=0$$ in the orange region of the figure (with the boundary conditions given in the figure).

Can we use the separation of variable method for solving this problem? I have tried to find the solution by separation of variables, but it seems the the boundary conditions can not be satisfied. Do you have any idea for solving this problem, theoretically?

figure

Improve this question
$\endgroup$
4
  • $\begingroup$ An naive (but useful) advise is: Use superposition principle! $\endgroup$
    – Dox
    Commented Jul 18, 2014 at 16:15
  • $\begingroup$ For using superposition principle, we must choose complete eigen functions. Which eigenfunctions can I use? $\endgroup$
    – Mojtaba Golshani
    Commented Jul 18, 2014 at 17:06
  • $\begingroup$ If you want analytic solution, perhaps the method of complex functions will help you: feynmanlectures.caltech.edu/II_07.html $\endgroup$
    – Ján Lalinský
    Commented Jul 18, 2014 at 17:49
  • $\begingroup$ Well, you could try solving it numerically. The interior is obviously going to be a pain, but it should be doable. $\endgroup$
    – Kyle Kanos
    Commented Jul 18, 2014 at 19:55

2 Answers 2

Reset to default
1
$\begingroup$

If we ignore the inner section, we have a box with 3 sides held at V = 0 and the top edge at V = V1. I'm pretty sure this is easily solvable by separation of variables using an oscillatory solution in x with a decaying solution along y. Using superposition we can then treat the inner box as a separate problem of similar geometry/boundary conditions. The exact solution would be a bit tedious with the origin being held at a corner of the larger box but I don't see how it's not solvable in principle.

Improve this answer
$\endgroup$
1
$\begingroup$

OK, I will try to say something a bit more useful than my other response. I am still on the skeptical side of getting a closed-form, simple solution to this problem, specially using separation of variables. I think that the problem is that the potential outside of a square with that border conditions cannot be attacked using separation of variables. My reasoning is that, whatever the solution, very far from the square it should behave as $$\ln \frac{1}{\sqrt{x^2+y^2}},$$ and it is not hard to prove that this is inconsistent with a potential expressible as the product of a function depending on $x$ and a function depending on $y$. Since the potential inside a square can be solved by separation of variables, if the problem of this question could be solved by separation, then by superposition we could solve the problem of the external field to a square by separation of variables. Therefore, the original problem cannot be solve by separation of variables.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.