I have a particle with total energy $E$ confined in a potential $$U(x) = -\frac{\cos^4x}{2} - m \cos x - f \sin x. $$ The constants $f$ and $m$ are both in the range (-2,2). The energy is such that the particle is in a bound state, with turning points $x_1$ and $x_2$ on either side of the origin.
I want to make the following statement:
If and only if $f = 0$, $$\int_{x_1}^{x_2} \frac{\sin x dx}{\sqrt{E - U(x)}} = 0.$$
One direction of the implication is simple. If $f=0$, the potential is even, and $x_1 = -x_2$. Since the integrand is odd, this integral obviously vanishes. But I'm struggling with showing that the integral is zero only if $f = 0$. Is this true? My numerical solutions to the equations of motion seem to indicate so.
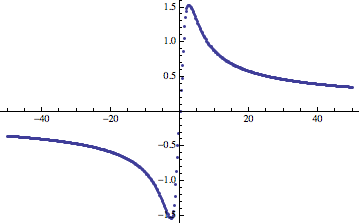
EDIT: I evaluated the integral numerically in Mathematica with $E = -2,~m = 2$, and for a wide range of values of $f$. The plot below shows the value of the integral on the vertical axis, and the input $f$ value on the horizontal. It looks like any proof relying on monotonicity will not work.