1
$\begingroup$

What is the difference between single mode and multi mode optical fibres? First off, I guess that by modes we mean the spatial modes of the electric (or magnetic?) field right?

Now: what makes a fibre able to support more than a single mode? I mean, what aspect of its structure corresponds to which mode(s) can be transmitted?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
1
$\begingroup$

It's simply the diameter of the fiber core. In a single-mode fiber, only the lowest-order mode fits physically into the fiber.

Improve this answer
$\endgroup$
0
$\begingroup$

You can assume either electric or magnetic field for simplicity, they both are present in light as it's electromagnetic wave.

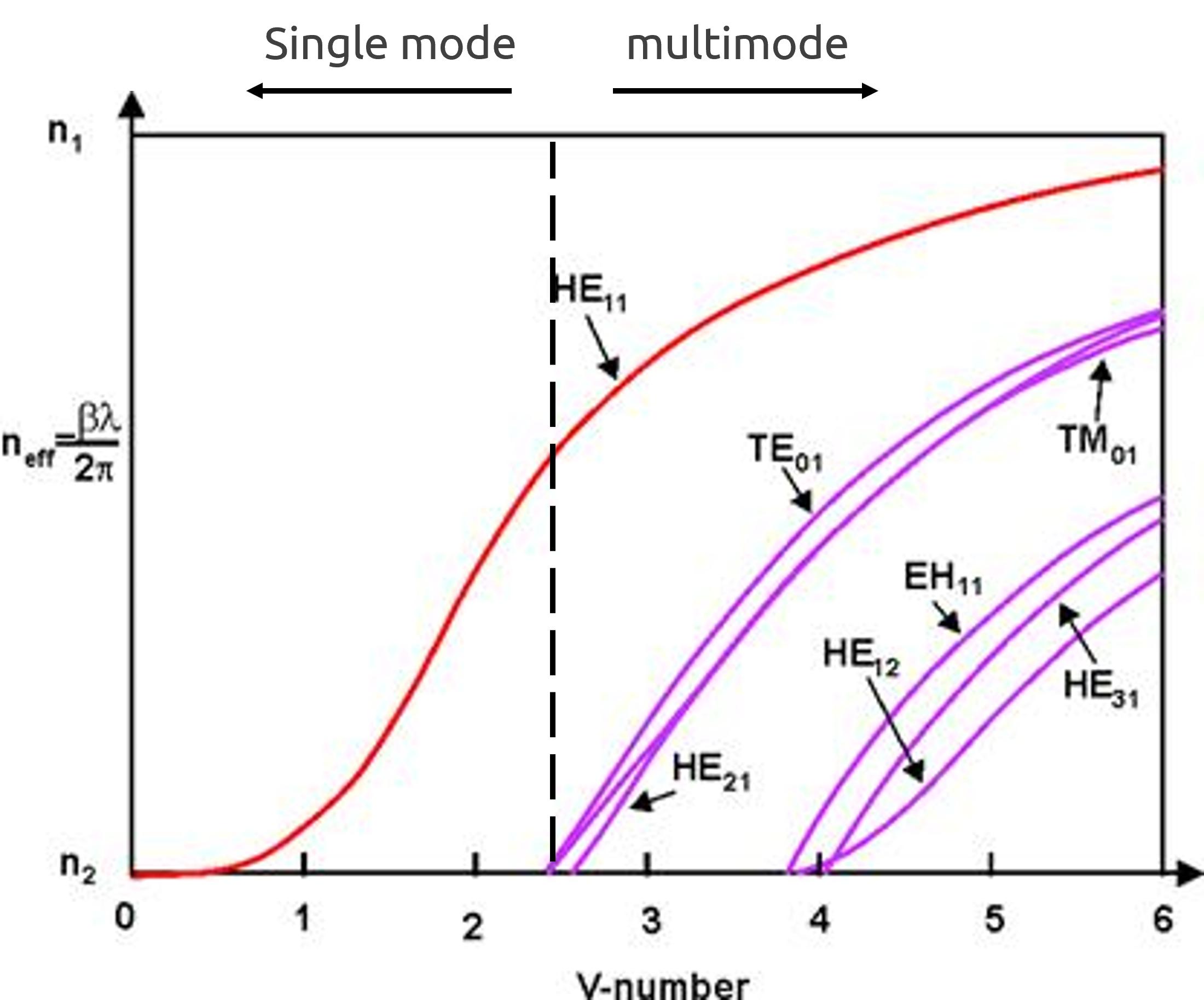
Now, mode is a sustainable pattern in the fiber optic cable. Imagine waves on a string, only the integral multiples of half wavelengths effectively form a mode. Let there be a complex output pattern at the output of a string. It turns out if we aren't playing with it continuously, it will be essentially periodic, maybe long period. And we can always reduce it via fourier transforms to a sum of some basic modes. Imagine the output at the end of a MMF(multi mode fiber) as waves on a string with intensity output as the wave amplitude on the string. It may be complex. But you can simplify the output by considering it as a sum of several modes. You can play with waves on a string here. It won't do a fourier transform though. http://phet.colorado.edu/sims/wave-on-a-string/wave-on-a-string_en.html

The concept is a little different for SMF and MMF. In case of SMF, Ray theory fails and light propagates as a wavefront as explained here: Single-mode fibers and ray-theory of light

Improve this answer
$\endgroup$
0
$\begingroup$

The V-number defines the mode of any fiber. It depends on wavelength, diameter of fiber and refractive index.

enter image description here

The v number is

\begin{align} \ V^2 &= \frac{2𝜋𝑑}{𝜆} (𝑛_1^2−𝑛_2^2)\\\\ \end{align}

Each mode has an effective index that can be defined by: \begin{align} \ 𝑘_𝑧 = 𝑛_𝑒𝑓𝑓 \frac{2𝜋}{𝜆}\\\\ \end{align}

The effective index tells you how tightly the mode is confined to the waveguide core

  • Guided mode 𝑛_𝑐𝑙𝑎𝑑 < 𝑛_𝑒𝑓𝑓 < 𝑛_𝑐𝑜𝑟𝑒

  • Tightly confined to the core 𝑛_𝑒𝑓𝑓 ~ 𝑛_𝑐𝑜𝑟𝑒

  • Weakly confined to the core 𝑛_𝑒𝑓𝑓 ~ 𝑛_𝑐𝑙𝑎𝑑

  • Unguided or radiating modes 𝑛_𝑒𝑓𝑓<𝑛_𝑐𝑙𝑎𝑑

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.