The Earth turns with a very high velocity, around its own axis and around the Sun. So why can't we feel that it's turning, but we can still feel earthquake.
-
5$\begingroup$ I guess it's the same way you can't "feel" that you are driving 100KM/h in a car, you only "feel" acceleration or deceleration. $\endgroup$– David d C e FreitasCommented Jul 20, 2011 at 10:37
-
20$\begingroup$ @David Freitas: That's a pseudo-explanation and a terrible analogy. $\endgroup$– Qmechanic ♦Commented Jul 20, 2011 at 18:39
-
3$\begingroup$ @Qmechanic Agree. $\endgroup$– lamwaiman1988Commented Jul 21, 2011 at 1:08
-
10$\begingroup$ @David Freitas: You are under acceleration when the earth is turning, though... $\endgroup$– Christian MannCommented Jul 21, 2011 at 19:16
-
5$\begingroup$ @Pieter Müller: i) When one drives on a road there are all kinds of vibrations; ii) if the car is on the rotating Earth, one would have to assume that the road is an initial frame, which is the very assumption that OP is questioning in the first place; iii) or if we imagine that the "car" is really a spaceship in empty space, then David Freitas is comparing the fact one cannot feel the velocity of the spaceship, due to Galilean invariance of inertial frames, with the unrelated fact that one cannot feel the centrifugal acceleration on the surface of Earth, which is in an accelerated frame. $\endgroup$– Qmechanic ♦Commented Nov 5, 2012 at 16:31
5 Answers
Because the rotation of the earth is very smooth and doesn't change, the centripetal acceleration we feel is very nearly constant. This means that the (small) centrifugal force from the rotation gets added to gravity to make up the "background force" we don't notice.
Earthquakes are not at all smooth and the accelerations involved are large and change direction a lot. This makes it easy to feel them.
-
2$\begingroup$ The rate of change in acceleration is sometimes called “jerk”. It can be used to quantify how much passengers on a vehicle are shaken. $\endgroup$ Commented Jul 20, 2011 at 20:05
-
5$\begingroup$ There's always this standard reply to your objection. $\endgroup$– DanCommented Feb 2, 2012 at 4:17
-
1$\begingroup$ @ldog: Furthermore, in general relativity, gravity is just a kinematic effect caused by the observer not being in an inertial reference frame. $\endgroup$– DanCommented Feb 10, 2012 at 20:11
-
1$\begingroup$ @Dan: That's the definition of a fictious force. And in GR, an inertial reference frame means a free-ly falling reference frame. $\endgroup$ Commented Jul 17, 2013 at 10:20
-
1$\begingroup$ @Dimension10: My issue is with the word "fictitious". A lot of people seem to think it means that they don't really exist, or are purely imaginary. I would argue that they are forces in the same sense that phonons are particles. I tend to prefer the terms "virtual" and "kinematic", but I don't know how widely used they are. $\endgroup$– DanCommented Jul 17, 2013 at 16:22
Dan's answer is essentially good, but miss one effect : the Coriolis effect. You can imagine a planet spinning much more rapidly than the earth, but at a constant angular speed. On that quickly rotating planet, the explanation of Dan would still stand, but as soon as on moves, we would feel a lateral Coriolis force.
The Coriolis acceleration is $2\vec{\Omega}\times\vec v$, where $\vec{\Omega}$ is the (vectorial) angular frequency of the planet's rotation and $\vec v$ the speed of the object moving. For an object moving at the speed of sound (340 m/s) near the Earth's pole, where the effect is maximum, the Coriolis acceleration is $$2\frac{2\pi}{24\times60\times60}\times 340 \simeq \frac{12\times 340}{24\times 3600}\sim \frac1{20} = 5\times10^{-2} \mathrm{m}\cdot\mathrm{s}^{-2}.$$ This corresponds to an acceleration which is half a percent of the gravity acceleration, for a situation which is already quite far from everyday life.
This small effect can accumulate over long distance and can have visible effects, notably at meteorological scales. In some sense, we feel the Earth rotation when we feel the dominant wind direction in our region. The parameter characterizing the intensity of the Coriolis effect for a phenomenon is the Rossby number, which is big if the Coriolis effect is negligible. If the phenomenon you analyse has a typical speed $v$, occur over a distance $L$, the Rosby number is essentialy proportional to the ration of the rotation period (24 h in our case) over the time $v/L$ it takes to go over the typical distance.
For meteorological depressions, the wind take several days to go over the thousands of kilometres they span, and the Coriolis effect has an important effect. To really feel the effect in everyday's life, one would need to be on a planet with a day of a few seconds, like the Little Prince's lamplighter's planet ! Of course, if you don't live on a rapidly rotating asteroid, you can see the effect on a carousel.
-
8$\begingroup$ Another way to feel (err... actually to see) the rotation of the Earth is with a Foucault pendulum. It's behavior can also be explained in terms of Coriolis forces. $\endgroup$ Commented Jul 20, 2011 at 19:57
I know it's very late in the game for this question, but this is partly a biology question. We don't feel the rotation of the earth because our brains are biased, they evolved that way. It's not useful to experience/be aware of this rotation day by day, in the same way it isn't useful to be aware of gravity. This is also why this optical illusion works:
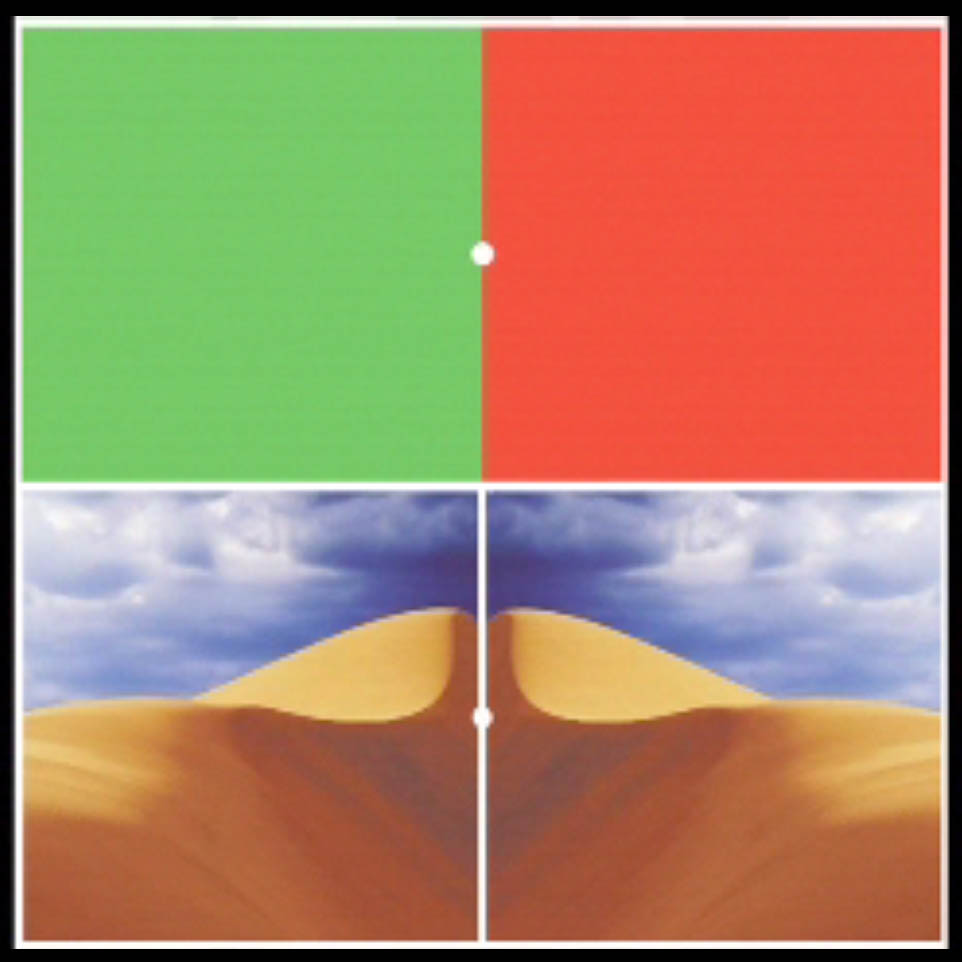 Stare at the white dot between the green and red for about 30 seconds, then look at the white dot between the identical desert pictures.
Stare at the white dot between the green and red for about 30 seconds, then look at the white dot between the identical desert pictures.
Our brains constantly adjust to what is "normal". In the above illusion, your brain "learns" that the right side of its field of vision is under red illumination, while the left side is under green illumination. Looking at the desert scenes below then reflects this new bias your brain have adopted.
Dan also touched on this in his answer, talking about the "background force" we don't notice. It is vital that the rotation is fairly constant, because our brains need time to adjust. But if somehow the earth suddenly started rotating at a higher but equally constant angular velocity, we all might be struggling a bit for a while.
In the case of adjusting the colour bias for your eyes, the adjustment is made within a few tens of seconds, but other changes are harder to adjust to. An example are upside-down goggles. Doing some googling brought me to the wikipedia page for perceptual adaptation. Although this focuses on the adjustment of the visual sense, this adaptation is a general function of the brain. In the case where the background force changes, I suspect the adaptation would take about the same amount of time as in the case of the upside-down goggles, maybe a little less - though this is only a guess.
Why we do feel earthquakes is then easy to understand. The bias allows our brains to effectively block out a background force, but the forces of an earthquake are not part of this background and are therefore felt. It's like receiving an audio signal with a constant background noise. Because it is unhelpful (and annoying) to hear this background noise all the time, you adjust your bias. But irregular noise or a signal will still be heard. This is an earthquake in the analogy.
-
2$\begingroup$ While I understand how perceptual adaptation plays a role in us “not feeling” earth’s gravity, I don’t get how it can play a role in feeling forces linked with earth rotation, which are basically too weak to be felt. $\endgroup$ Commented Aug 13, 2015 at 19:45
-
$\begingroup$ The biological answer is surely the most relevant one. With regard to your last paragraph, when I was in Tokyo for the first time, I noticed that most of the locals were barely aware of earthquakes that to me seemed quite strong. When I pointed that one was underway out to a lady in a shop (who was visibly swaying her body to compensate as she stacked books on shelves) she looked a bit confused at first and then, after a few moments said, "oh yes, so there is!" and then went cheerfully back to her book stacking. $\endgroup$ Commented Oct 28, 2015 at 0:36
-
$\begingroup$ "it isn't useful to be aware of gravity" Human literally has a sensing organ just to detect gravity. It's called semicircular canals. $\endgroup$– XwtekCommented Aug 17, 2022 at 10:17
-
$\begingroup$ @Xwtek Those do not detect gravity. You're thinking of changes in gravity (or any other acceleration on top of the baseline vertically down $\vec{g}$), deviations from the background force that we don't feel. This is my whole point. Granted, the semicircular canals probably should be mentioned in this context but as a clarification/explanation of the argument, not a negation of it. $\endgroup$– WouterCommented Aug 21, 2022 at 7:54
Can we keep this simple ?
The answer is that the acceleration associated with the rotation is very small and it is accounted for in the definition of the vertical.
The acceleration is small: $\omega^2 R \cos \theta = 0.032 m/s^2$ or 3 milli g at the equator. $\theta$ is the latitude.
The acceleration makes an angle of $\theta$ with the direction to the Earth's centre. The total force is the vectorial sum of gravity and centripetal force and this merely redefines the vertical direction by a tiny amount. If your floor was made plumb level this tiny deviation is built into your house and city.
we don’t feel the Earth spin because we, the atmosphere, skyscrapers, and everything else are spinning along with the Earth at the same constant speed.
-
2$\begingroup$ That's too simplistic. If you are in a car that is turning, you can still feel it, even with your eyes shut and even though everything around you that you can feel is turning with you. The real answer is that we can feel turning, just that the earth is turning so slowly that it is below our human perception limit. $\endgroup$ Commented Jan 7, 2014 at 17:01
-
$\begingroup$ This isn't a simplification: it's just completely wrong. The fact that everything we see is moving with the same acceleration and speed as us doesn't make us not feel the force in any way. $\endgroup$– user191954Commented Jun 24, 2018 at 11:31
