Suppose a light ray is emitted by a light source very close to the black hole's singularity away from the black hole's gravitational center. Why won't the light escape? Shouldn't the massive gravity of the black hole just cause massive redshift in the light ray? How does it pull it back? I know there might be many questions similar to this but I just need a basic answer for the redshift part. I understand that if a light ray is passing nearby the black hole, then it bends and gets pulled inside. But what if the light is going perpendicularly away from the black hole? The source is inside the event horizon of course. Otherwise this is just a normal case.
$\begingroup$
$\endgroup$
6
asked Jun 17, 2014 at 18:10
-
1$\begingroup$ Is the source inside the event horizon, or outside? (There is now some doubt about whether event horizons actually exist, but bear with me.) $\endgroup$– BetaCommented Jun 17, 2014 at 18:17
-
1$\begingroup$ possible duplicate of If you shoot a light beam behind the event horizon of a black hole, what happens to the light? $\endgroup$– John RennieCommented Jun 17, 2014 at 19:23
-
$\begingroup$ I've linked a question that seems to me to answer what you're asking, i.e. what happens to a light beam directed outwards from inside the event horizon. If the maths in that question is a bit too heavy let me know and I'll attempt a simpler explanation here. $\endgroup$– John RennieCommented Jun 17, 2014 at 19:26
-
$\begingroup$ The edited question is clearer, but you still seem to be confusing two different questions, 1) will the ray escape? (No, it won't), and 2) will it be red-shifted? (Yes, for some observers.) $\endgroup$– BetaCommented Jun 17, 2014 at 19:46
-
$\begingroup$ Well beta you said it will be redshifted for some observers. If it cannot escape how will it be observed? And why will it not escape? The answer below says >If the light will go away from the black hole, it will redshift, but will be able to go away. $\endgroup$– rahulgarg12342Commented Jun 17, 2014 at 19:54
|
Show 1 more comment
2 Answers
$\begingroup$
$\endgroup$
2
It is not so simple. On your question I think you try to estimate its behavior based on the Newtonian Mechanics. But this goes based on integrated curves based on the Einstein Field Equations and its mathematics is totally different. For example, the orbits aren't are generally not closed and not stable.
If the light will go away from the black hole, it will redshift, but will be able to go away. Light passing the event horizon, will go into the hole and will never get out.
Light going initially on a circular orbit will also end in the black hole if it started below $3/2R_g$.
You can read more about the stable/metastable and unstable orbits around the BH here.
-
$\begingroup$ Do in short light will be able to escape? $\endgroup$ Commented Jun 17, 2014 at 18:55
-
$\begingroup$ No, if it starts in the horizon, then it won't. But don't imagine it as if some like a "force" acted upon the light and dragged it back. In general relativity, there is no such thing. In GR you should think on gravity, as if the directions of the space(time) changed. In the case of an EH, they are changed so, that there is no direction leading out of the BH, just as there is no direction going back to the past in our world. $\endgroup$– peterhCommented Jun 17, 2014 at 21:34
$\begingroup$
$\endgroup$
0
The radial coordinate inside the black hole is time-like, so when you are into the black hole (according to standard General Relativity and considering a Schwarzschild black hole) you are forced to go to the singularity. It's like if the radial coordinate is our usual time coordinate, in which you can't travel to the past.
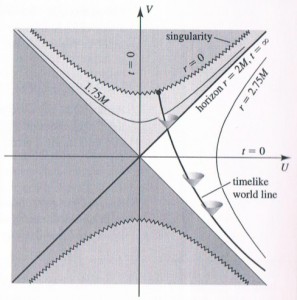
See for example this Kruskal diagram of the black hole. The maximum speed you can reach is $ c $, that means going on a $ 45° $ line into the diagram. Massive particles are forced to stay into the light cone and they follow $ 0-45° $ trajectories. You can't do better than $ 45° $ and you can't go away from singularity. Moreover, the horizon is the infinite redshift surface (more precisely, the effective infinite redshift surface is slightly outside the horizon, because you don't have an experimental device with infinite sensibility).
