Does the opening angle of the cone matter?

Question

When discussing orbital mechanics, you learn that all orbits roughly follow an ellipse which is obtained as the intersection of a cone with an inclined plane, creating conic sections.

Below is a plot of different mathematical variables of a cone. I am trying to figure out if the ellipses that orbits follow are a special rule where the opening angle (at the top of the diagram) of the cone is 90 degrees, or if it is irrelevant what that angle is.

Answer 1

The angle is not important when mentioning elliptical orbits. Let's consider two cones with $\theta_{1}$ and $\theta_{2}$ respective; with $\theta_{1} < \theta_{2}$ (not shown, but take my word).

Both cones have an ellipse, who's center is $y$ from the top of the cone. The solution to the orbit equation in it's most general form is:

$r(\phi) = \frac{\ell^2}{m^2\gamma}\frac{1}{1+e\cos\phi}$

and is $y$ independent.

The cone with the the larger angle ($\theta_{2}$) has an ellipse that is larger by a certain factor $ A = \frac{tan\theta_{2}}{tan\theta_{1}}$. That is if $r_{1}(\phi)$ is associated with $\theta_{1} $ and $r_{2}(\phi)$ is associated with $\theta_{2}$ then,

$$ \theta_{1}\rightarrow \theta_{2} \Rightarrow r_{1}(\phi) \rightarrow Ar_{1}(\phi)=r_{2}(\phi). $$

It also follows that if cone 2 has a different angle than cone 1, there exists a y for cone - 1 such that $r_{1}(\phi)=r_{2}(\phi)$. But since the solution is independent of y, then it does not matter what $\theta$ you choose.

Answer 2

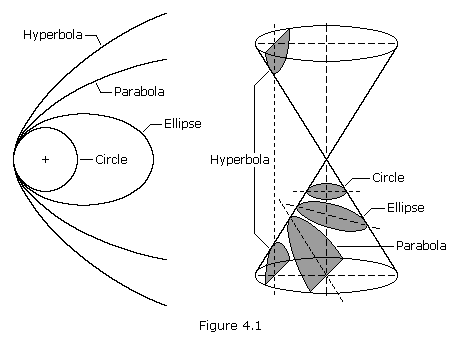
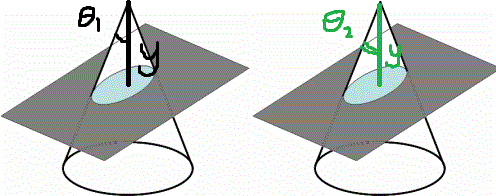
You can get all three classes of conic sections as intersections of planes which do not include the vertex with a cone of any nonzero vertex angle. You get a circle as the intersection between a plane which does not pass through the vertex and whose unit normal is in the same direction as the cone's axis of rotational symmetry. An ellipse is the intersection of a plane not through the cone's vertex and whose unit normal makes an angle with the cone's axis of rotational symmetry which is less than the half vertex angle. When the plane's unit normal makes an angle with the symmetry axis that is equal to the half vertex angle, its intersection with the cone is a parabola. If the angle between the plane's unit normal and the symmetry axis is greater than half the vertex angle, you get an hyperbola.

You can experiment with these ideas as follows. Let $V$ be the position vector of the cone's vertex, with its axis of symmetry defined by the ray through $V$ with direction defined by the unit normal $N$. Work out its intersection with the x-y plane. Let $X$ be a vector in the x-y plane on the cone; then:

$$(\left<X-V,\,N\right>)^2 = const^2 \times \left<X-V,\,X-V\right> \Leftrightarrow \cos\theta = const = \frac{\left<X-V,\,N\right>}{\sqrt{\left<X-V,\,X-V\right>}}$$

which you can see is a general quadratic locus. The above equation says that the cosine of the angle $\cos\theta$ between the cone's symmetry axis $N$ and the line $X-V$ joining the point in question and the vertex is constant. Write the equation out in full and see what it defines in the different cases:

1. $N=(0,0,1)$ (circle)
2. $N$ lies in $x-y$ plane (hyperbola)
3. Cosine of angle between $N$ and the $x-y$ plane equal to $\cos\theta$ (parabola)
4. Cosine of angle between $N$ and the $x-y$ plane less than $\cos\theta$ (ellipse)

You'll find you can get an ellipse of any eccentricity by changing $V$. Condition 2 represents an hyperbola in the $x-y$ plane with an angle $\theta$ between its asymptotes. Here we see that the vertex angle does limit the class of hyperbolas one can get as sections of the cone in question.