The angle is not important when mentioning elliptical orbits. Let's consider two cones with $\theta_{1}$ and $\theta_{2}$ respective; with $\theta_{1} < \theta_{2}$ (not shown, but take my word).
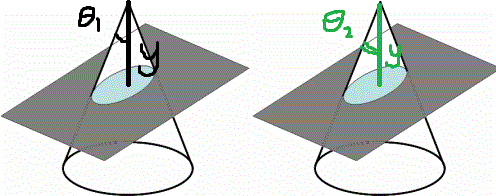
Both cones have an ellipse, who's center is $y$ from the top of the cone. The solution to the orbit equation in it's most general form is:
$r(\phi) = \frac{\ell^2}{m^2\gamma}\frac{1}{1+e\cos\phi}$
and is $y$ independent.
The cone with the the larger angle ($\theta_{2}$) has an ellipse that is larger by a certain factor $ A = \frac{tan\theta_{2}}{tan\theta_{1}}$. That is if $r_{1}(\phi)$ is associated with $\theta_{1} $ and $r_{2}(\phi)$ is associated with $\theta_{2}$ then,
$$ \theta_{1}\rightarrow \theta_{2} \Rightarrow r_{1}(\phi) \rightarrow Ar_{1}(\phi)=r_{2}(\phi). $$
It also follows that if cone 2 has a different angle than cone 1, there exists a y for cone - 1 such that $r_{1}(\phi)=r_{2}(\phi)$. Since yBut since the solution is independent of both general solutionsy, then it does not matter what $\theta$ you choose.
