Thermo-Emf variation with temperature

Question

In the following experiment for seebeck effect

After a certain temperature, the thermo-emf begins to fall. Why does this happen? What is happening microscopically at this level to cause such an effect ?

Answer 1

The calibration curve you show isn't typical. In fact there is no typical calibration curve because different types of thermocouples can have very different voltage:temperature dependences. There are lots and lots of articles giving different calibration curves - I selected this PDF as being fairly representative, or try this image search for lots of other calibration curves.

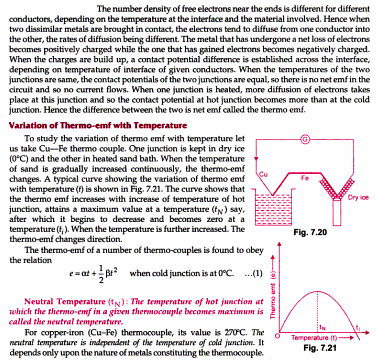
But we can interpret your question more broadly as asking about the physics underlying thermocouples. There is a nice paper summarising the physics downloadable as a PDF here. To give a rather basic summary, the distribution of electron energies in a metal follow a Fermi-Dirac distribution looking something like this:

You might also be interested to read the article this image came from as it covers related material.

When you heat a metal you produce a population of electrons with energies above the Fermi energy, and that population is temperature dependant. The Seebeck effect arises because if two ends of a metal wire are at different temperatures the electrons at hot end will have higher energies than the ones at the bottom end and they can lower their energy by moving from the hot end to the cold end. This movement of charge creates a potential difference between the ends of the wire.

This sounds simple enough but there are lots of complications. Firstly the size of the voltage difference depends on the shape of the energy distribution, but this depends sensitively on the shape of the Fermi surface. The paper by Fujita and Suzuki suggests a way to calculate the Seebeck coefficient, but they gloss over complications like the density of states near the Fermi surface.

While we're on the subject, both electrons and holes contribute to the Seebeck effect, and their contributions are opposite. Which domnates depends on the shape of the Fermi surface, and again this is a complication that is mentioned by Fujita and Suzuki but glossed over.

Finally, it's exceedingly difficult to measure the Seebeck effect in a single wire. What a thermocouple does is use two wires of different metals, and the voltage it generates is the difference between the potentials generated in the two wires. So the temperature dependence of a thermocouple voltage is the difference between the temperature dependences of the Seebeck coefficients in the two different wires. As discussed above, the temperature dependence of the Seebeck coefficient is hard enough to calculate in one material, but now you have to calculate it twice for two different materials and take the difference. Since it's a difference between two fairly similar quantities the thermocouple voltage can go up, down, up then down as you show, or even up then down then up again.

So at the end of the day I can't really answer your question, excpet to rather lamely say it's complicated. Still, I hope this has been useful and there are a few references for you to follow if you really want to pursue this in detail.

Answer 2

$v(drift)=\dfrac{A}{B}$

where A=(Mass*Voltage)

and B = (density*Avogadro's number*Length*fundamental charge*free electron #resistivity at 0°C(1+temperature coefficient of resistivity at 0°C*temperature

$v=\dfrac{(MV)}{(dNLefp(1+aT))}$, so with this equation we know the drift velocity of the electrons.

From this equation we will do some calculus.

$v=\dfrac{(MV)}{(dNLefp(1+aT))}$ can be rewritten as $dv=\dfrac{(MdV)}{(dNLefp(1+aT))}$

${\dfrac{dv}{dV}}={\dfrac{M}{(dNLefp(1+aT))}}$ is the change in drift velocity with respect to voltage, as temperature increases the drift velocity with respect to voltage decreases.

${\dfrac{dV}{dv}}={\dfrac{(dNLefp(1+aT))}{M}}$ this is the change in voltage with respect to drift velocity, as temperature is increased, the rate at which voltage changes increases.

Now the question will $\dfrac{dv}{dV}$ overpower $\dfrac{dV}{dv}$? lets take the derivative.

${(\dfrac{dV}{dv})}{\dfrac{1}{dT}}={(\dfrac{1}{dT})(\dfrac{(dNLefp(1+aT))}{M})}={a*{\dfrac{dNLefp}{M}}}$ (rate of change is constant)

${(\dfrac{dv}{dV})}{\dfrac{1}{dT}}=(\dfrac{1}{dT})(\dfrac{M}{(dNLefp(1+aT))})={-\dfrac{Ma}{dNLefp(1+aT)^2}}$ (this will quench the system forcing the drift velocity to go to zero).

drift velocity is equivalent to electron motion down a wire.