How might eddy currents and terminal velocity be broken down?

Question

This is a branch from this question.

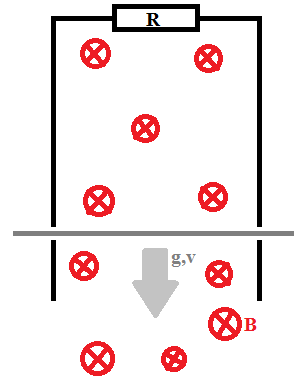
With regards eddy currents and terminal velocity I have located a homework question but would appreciate more detail (and translation of the symbols described therein) about the forces at play.

Eddy currents are generated though the resistance of electrons interacting with magnetic fields as they cut across coils and force them downwards.

Since there is resistance it would stand to reason that this would reduce the speed at which a magnet falls - but that there would be an equilibrium whereby further slowing a magnet would reduce the flux generation required to sustain that reduced descent.

So the forces at play include:

• gravity
• mass of the magnet
• coils of the coil (solenoid?)
• air resistance (may be discounted?)

May these be further broken down? Are other variables at play?

Answer 1

Yes, faraday law and eddy current will cause terminal velocities to exist for falling objects outside of any other friction consideration. The most widely used example to show it is this setup:

These are two connected rails of width $l$ with a third rail freely gliding along the two main rails. The whole setup can be reduced down to a single winding of varying (increasing) size (varying length $L$), and of ~fixed resistance R. The winding is placed in a perpendicular uniform constant magnetic field $\vec{B}(\vec{r})=B_0 \vec{1_z}$. An analysis of this simplified problem quickly gives: $$ \text{When terminal velocity is reached, the whole gravitic power is dissipated}\\ \text{through the resistance, and none contributes to an actual increase in kinetic energy, hence:}\\ P_{gravity}=P_{dissipated}\\ \text{an expression of mechanical power, and an expression of electrical power:}\\ \Leftrightarrow m\vec{g}\cdot\vec{v}=mgv=\frac{U^2}{R}\\ \text{Faraday Law:}\\ \Leftrightarrow mgv=\frac{\left(\frac{d\Phi}{dt}\right)^2}{R}\\ \text{Expression of the magnetic Flux. The only non time-constant variable is L:}\\ \Leftrightarrow mvg=\frac{\left(\frac{d(B\cdot L\cdot l)}{dt}\right)^2}{R} =\frac{\left(B\cdot l \cdot \frac{dL}{dt}\right)^2}{R}\\ \Leftrightarrow mvg=\frac{(Blv)^2}{R}\Leftrightarrow v=\frac{mgR}{(Bl)^2}$$

Once you have understood this, you will be able to physically grasp why a terminal velocity would exist both in this situation and when the magnet is falling through the coil, and to apply the exact same method to your precise situation if you want to further analyse your experiment.