I was wondering whether there is a simple formula in order to calculate the ground velocity (the velocity with which the satellite is moving along the planet's surface) on basis of the orbital velocity.
So for instance, a GEO satellite at Earth has an orbital velocity of around 3.07 km/s, while its ground velocity is 0.
The reason I would like to calculate the ground velocity, is to find the time to traverse one pixel on the planet's surface.
You must assume circular orbits, as stated in another answer. The ground speed will also be variable due to inclination, unless it's equatorial. An extreme example is an east-west orbit versus a west-east orbit. In one case, the ground velocity is added, in the other case, it's subtracted.
But with the query limited to equatorial orbits, we can continue. Find the orbital velocity from the radius. Convert the orbital velocity to angular velocity, and then multiply by the Earth's radius. Last, subtract the Earth's movement from this.
$$ v_{gnd} = \sqrt{ \frac{ GM }{ r} } \frac{ 1 }{ r } R_e - 0.465 \frac{ km }{s} \\ = \left( 7.9 \frac{km}{s} \right) \left( \frac{ R_e }{ r } \right)^{3/2} - 0.465 \frac{ km }{s} $$
You can simply plot this. For length units, I'm using multiples of Earth's radius. So r=1 here is the surface.
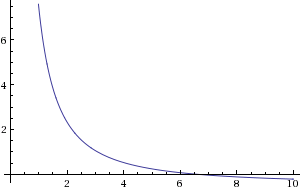
For inclined orbits, the above relationship will be combined with some trigonometry, and change over the period of the orbit.
Geostationary satellites travel in circular orbits about the equator. If you express the velocity as angular velocity, it will equal the angular velocity of Earth's rotation, about $7.3 \times 10^{-5} radians/second$.
If you had another satellite in circular orbit about the equator, but not geostationary, you could subtract the angular velocity of the Earth from the angular velocity of the satellite to get the angular velocity relative to the ground.
However, if the orbit of the satellite is elliptical or not along the equator, there will not be a constant velocity relative to the ground.
