Electric field in a sphere with a cylindrical hole drilled through it

Question

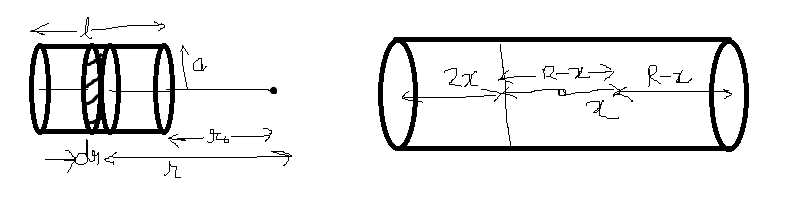
Suppose that you have a sphere of radius $R$ and uniform charge density $\rho$; a cylindrical hole with radius $a$ ($a\ll R$) is drilled through the center of the sphere, leaving it like a "necklace bead".

I would like to find a function for the electric field (1) very far away from the sphere ($r\gg R$) and (2) inside the hole, near the center of the bead $r\ll R$.

In case (1), I simply treat it as a point charge and calculating the electric field is trivial.

However, I am uncertain how to approach part (2) and would appreciate any assistance. The combination of spherical and cylindrical geometries seems to make this quite tricky. I am unsure what approximation or simplification to make from the knowledge that $r\ll R$.

Would it perhaps be correct to find the electric field from (1) a complete, uniformly charged sphere and (2) a cylinder of charge density $-\rho$? Summed together, the charge densities would result in our original "bead" system, so then I can just add together the expressions for the electric field. Doing case (1) is quite easy, but (2) is nontrivial for positions that are not along the axis of the cylinder, but perhaps due to our condition that $r\ll R$ and $a\ll R$, we can assume that the field from the cylinder along the $z$-axis is a good enough approximation.

Answer 1

For a cylinder:

---

$$dV=\pi a^2dr\\dq=\rho dV=\rho\pi a^2dr\\dE=Kdq/r^2=K\rho\pi a^2dr/r^2\\E=\int dE=K\rho\pi a^2\int_{r_0}^{r_0+l} dr/r^2=\frac{K\rho\pi a^2l}{r_0(r_0+l)}$$

---

In case inside of it as in the figure the field due to $R-x$ length cylinder is cancelled by a similiar one in the opposite side thus the resultant field is:

---

$$E=\frac{K\rho\pi a^2l}{r_0(r_0+l)} =\frac{K\rho\pi a^2(2x)}{(R-x)({R-x}+2x)}\\ =\frac{2K\rho\pi a^2x}{R^2-x^2} =\frac{2K\rho\pi a^2x}{R^2\left(1-\frac{x^2}{R^2}\right)} \approx\frac{2K\rho\pi a^2x}{R^2} \text{ as } x\ll R\\ =\frac{\rho a^2x}{2\epsilon_0R^2}$$

---

And for sphere:

$$\large E=\begin{cases} \frac{\rho x}{3\epsilon_0}\;0\le x\le R \\\frac{\rho R^3}{3\epsilon_0 x^2}\;x\ge R \end{cases}$$

---

Now $E$ can be easily calculated

$$E_{out}= \frac{\rho R^3}{3\epsilon_0 r^2}-\frac{\rho a^2(2R)}{4\epsilon_0(r-R)(r-R+2R)}\\ =\frac{\rho R^3}{3\epsilon_0 r^2}-\frac{2\rho a^2R}{4\epsilon_0(r^2-R^2)}\\ \approx \frac{\rho R^3}{3\epsilon_0 r^2}-\frac{\rho a^2R}{2\epsilon_0r^2} \text{ as } r\gg R \\ =\frac{\rho R}{6\epsilon_0 r^2}\left[2R^2-3a^2\right] $$

Note that, $\large\lim_{a\to 0}E=\frac{\rho R^3}{3\epsilon_0 r^2}$

---

Similiarly

$$E_{in}=\frac{\rho x}{3\epsilon_0}-\frac{\rho a^2x}{2\epsilon_0R^2}\\ =\frac{\rho x}{6\epsilon_0}.\left[2-3\frac{a^2}{R^2}\right]$$

Here also, $\large\lim_{a\to 0}E=\frac{\rho x}{3\epsilon_0}$

---

Answer 2

I agree with the result, but I would like explain another more general and rapid approach. Because of the radius of the hole is negligible with respect to the radius of the sphere, and the only posible direction for E compatible with the symmetry is the z axis, and finally having in mind that the tangencial components of E are continuous, the solution is exactly the same we obtain when only considering the sphere with a uniforme charge distribution.