We started learning about electromagnetism in physics class, and the Right Hand Rule comes in handy as seems easy to use, but I'm curious as to how it actually works. I guess it's more of a math question since I think it just involves solving the cross product of the velocity of the charge and the magnetic field. I don't know anything about cross products, but I searched some things up and it seems that the matrix is has unit vectors in it which determine the directions, so would one have to solve the whole thing to determine the direction of the force on the charge? I know it has to be perpendicular to both of the vectors but that still leaves 2 directions.
$\begingroup$
$\endgroup$
3
-
3$\begingroup$ An interesting point is that we usually end up using RHRs in pairs to get observables. That is, to compute the force between two currents we use one RHR to find the field due to current $A$ and another RHR to find the force on current $B$ due to the field from current $A$. This procedure would have given the same final force using left hand rules (though the direction of the field you found in the middle would be reversed; but recall that the assignment of magnetic poles was arbitrary in the first place). $\endgroup$– dmckee --- ex-moderator kittenCommented Mar 23, 2014 at 22:17
-
$\begingroup$ Is there any reason to chose one of the orientation of B? $\endgroup$– athenaCommented Jun 29, 2015 at 18:52
-
$\begingroup$ Most people are right handed. $\endgroup$– Jahan ClaesCommented Jun 29, 2015 at 19:40
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
2
The formula for the force of a particle due to its magnetic field is $F = q \vec v \times \vec B$. The cross product has the property that its result is always perpendicular to both arguments.
Its direction is simply a result of how the cross product function is defined and the sign of electric charge (an electron is defined as negative).
It is important to note that the order of the arguments matters as the cross product function is not commutative; generally $ \vec A \times \vec B \neq \vec B \times \vec A$. For vectors the direction will be reversed; for matrices both sides can be wildly different.
-
2$\begingroup$ "an electron is defined as negative when it should really have been positive)" See physics.stackexchange.com/q/17109 and physics.stackexchange.com/q/68471. It might have been more convenient in your first circuits class to have electrons positive, but it is purely a convention and there is no "should" involved. $\endgroup$ Commented Mar 23, 2014 at 22:14
-
$\begingroup$ @dmckee Yeah, 'should' is probably too strong -I'll use 'would have made more sense' or 'have been more convenient' in the future :) $\endgroup$– KvotheCommented Mar 23, 2014 at 22:18
$\begingroup$
$\endgroup$
Look at the diagram below,
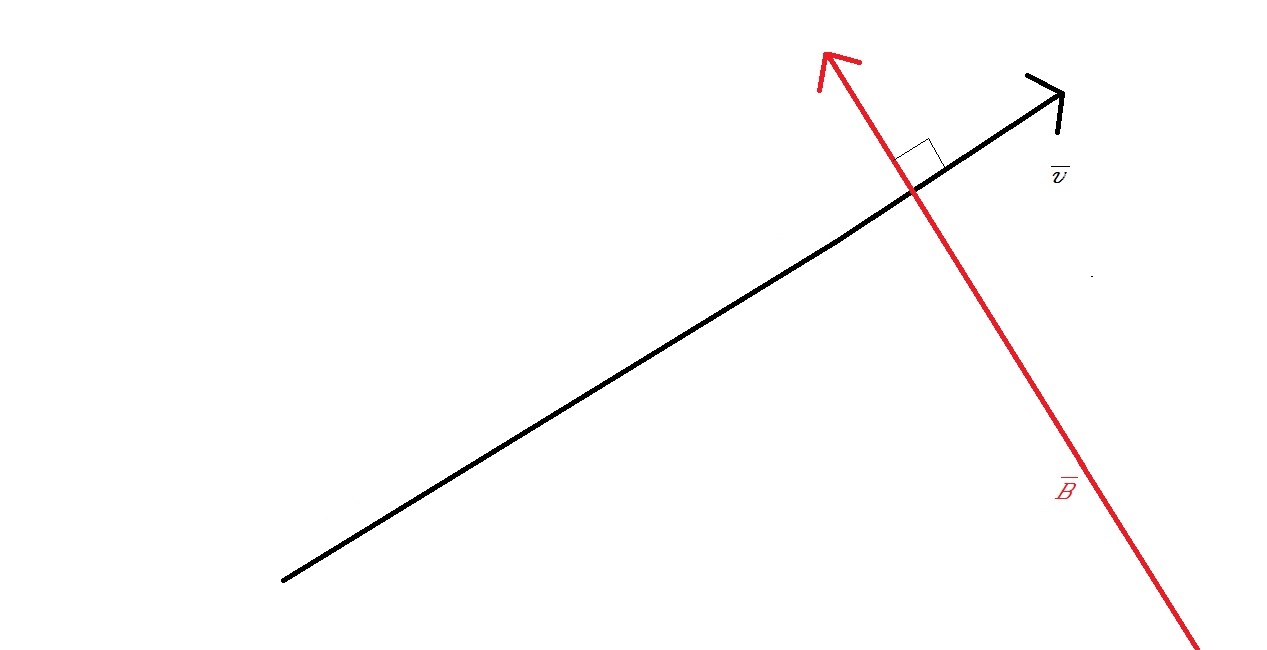
Two vectors $B$ (Magnetic field vector ) and $v$ ( velocity vector of a proton ) shown in the diagram are perpendicular to each other. Here, I have considered the simplest case where the two vectors are perpendicular to each other. The cross product of $B$ and $v$ gives us another vector $F$ ( Force vector ) which points in a direction perpendicular to both $B$ and $v$. This is the geometric meaning of the cross product. Say, $B$ and $v$ lie on the $x$-$y$ $plane$. The force vector obtained as a result of the cross product will point in a direction that is perpendicular to the $x$-$y$ $plane$. $F$ therefore points in a direction parallel to the $z$ $axis$. This leaves us with a question. In which direction does $F$ point? $-z$ $axis$ direction or $+z$ $axis$ direction. Using the $Right$ $Hand$ $Rule$, one can easily solve this issue.
Look at the diagram below,
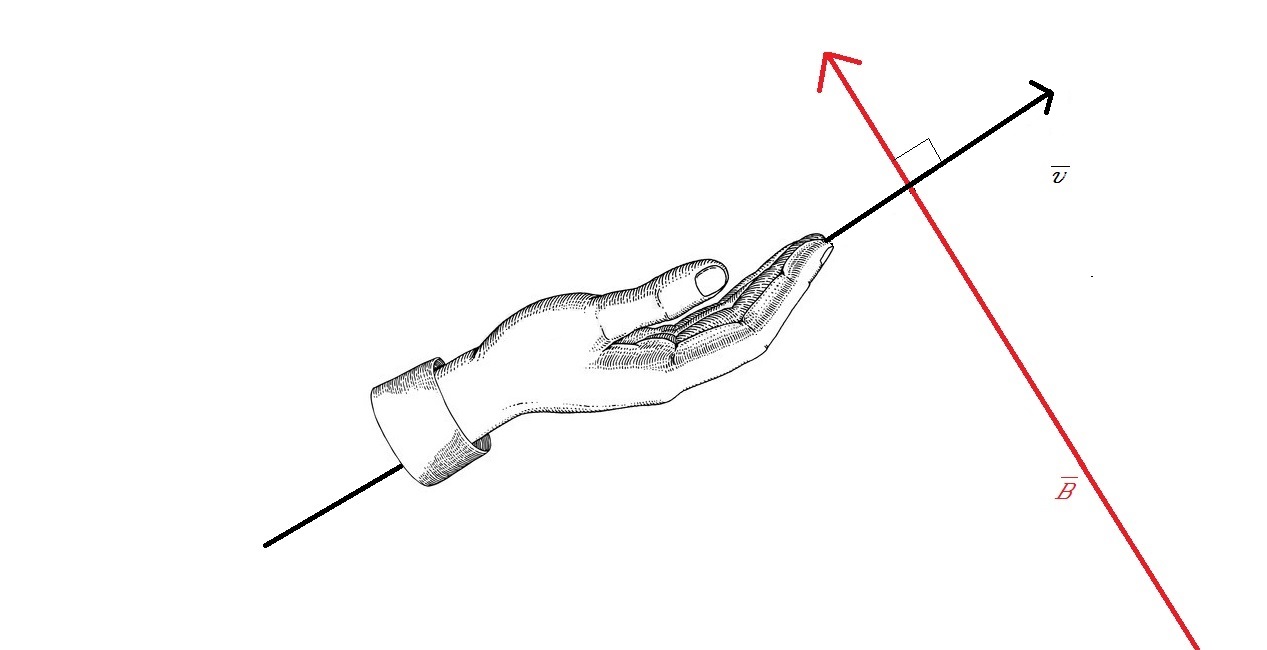
Place your right hand on $v$. Now, curl your fingers in the direction of $B$.
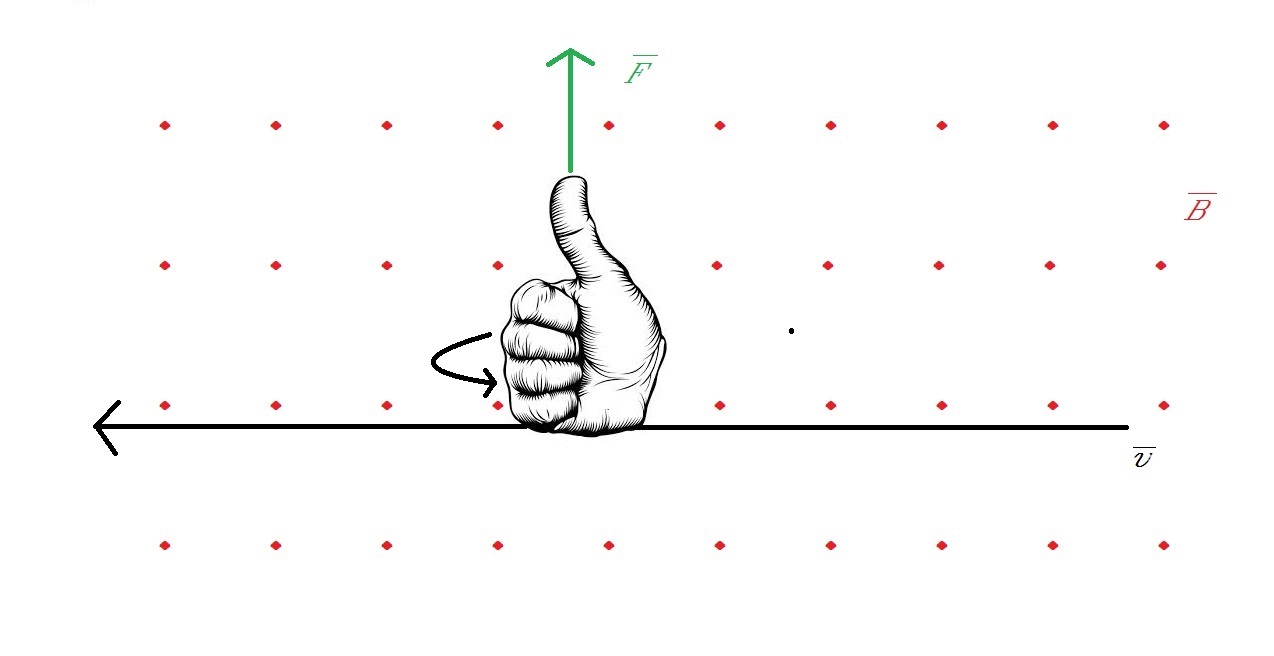
Here, $B$ is considered to be coming out of the screen. As you curl your fingers, you see that they are also coming out of the screen( in the direction of $B$ ). The thumb points in the direction of $F$. If $B$ were considered to be going into the page, $F$ would have pointed downwards.
Remember, I worked with a proton. If one works with an electron, one has to flip the direction of the force due to its opposite nature.
$\begingroup$
$\endgroup$
The cross product does not have a specific direction, but either the left hand rule or right hand rule works, but it has to be applied consistantly. That's the key. So you simply drum in one rule in everywhere.
It's kind of like driving to the left or to the right. Either works, but you have to be consistant about it.
$\begingroup$
$\endgroup$
1
The right hand rule is simply a mathematical convention. We use right handed coordinate systems. We could make the left hand rule true if we wanted to, but we would need to adapt our equations to the new convention.
-
$\begingroup$ This seems to be only part of an answer, the other part would be about how to use the RHR, as requested by the OP. $\endgroup$ Commented Nov 11, 2015 at 1:15