We know that energy of quarks inside the proton can not be exactly fixed because if it,the 'proton decay' must not be exist. My question is if the energy of quarks inside the proton is not exactly fixed than the mass of the proton must be fluctuate because 99% of the proton mass is due to the kinetic energy of the quarks and to the energy of the gluon fields that bind the quarks together. Is this fluctuation in mass really occur or I am missing something. please explain.
$\begingroup$
$\endgroup$
2
-
2$\begingroup$ It isn't clear what you are asking. The total energy of the proton is constant to very high accuracy, but how that energy is distributed between the particles making up the proton is variable. $\endgroup$– John RennieCommented Feb 28, 2014 at 6:41
-
$\begingroup$ What's your point? According to Wikipedia: Despite significant experimental effort, proton decay has never been observed. $\endgroup$– Thomas FritschCommented May 31, 2019 at 12:00
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
1
Yes it fluctuates but it is a very small fluctuation. Note that unstable particles have a decay rate or width $\Gamma$ that is related to its lifetime $\tau$ by $$ \Gamma=\frac{\hbar}{\tau} $$ when you measure the mass/energy of such particles in experiments you always get a Lorentzian or Breit-Wigner distribution like this
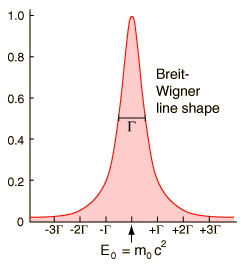
(source: gsu.edu)
from which you can measure the width and calculate both the mass (with an uncertainty) and the lifetime (from the width measurement). Note that this is for all unstable particles, even fundamental ones, then only stable particles as the electron have a perfectly defined mass.
The issue with the electron is that it's lifetime is very very long (otherwise aggregated matter wouldn't exist), in fact proton decay has never been observed, though theoretical decay modes exist in some models. So it is considered to be effectively stable and both experiments and theoretical calculation set limits for its lifetime. But it still has a lifetime in principle so its mass is not perfectly defined, the fluctuation is really small though.
-
$\begingroup$ Further comments: A quick numerical calculation shows the order of the value of the fluctuation. The mean value for the lifetime of the proton is of order $10^{39}$ years or $10^{46}$ seconds (note it is many orders of magnitude greater than the age of the universe). Now the Planck constant is of order $10^{-16}$ seconds eV. Then the width is of order $10^{-62}$ eV. The mass of the proton is of order $1000$ MeV/c^2 so $\Gamma/mc^2$ is of order $10^{-85}$. You can see that we can ignore this fluctuation. $\endgroup$– jpmCommented Feb 28, 2014 at 16:18
$\begingroup$
$\endgroup$
Take a simple quantum mechanical potential that describes an atom. The mass of the atom is fixed. Take hydrogen. The electron is in an orbital around the proton which is a probability distribution of its location in time and space: if you measure it, i.e. interact with it, where you may find it.
Correspondingly there exists an energy width to the energy lines of hydrogen which is very small , and is seen when electrons fall into the energy level and emitt slightly different frequency photons. This width is the equivalent measure of an orbital in momentum space.
When bound within the atom though what is measured externally , as the mass of the hydrogen atom, is constant because these probable values average out to zero when not interactively probed, so will not affect the mass measured collectively.
In a similar manner (and much more complicated, a many body quantum mechanical description) what the quarks do within the proton in their probabilistic evolution does not affect the external attributes of the proton, like the mass.
