the article in wikipedia says that in string theory the particles at lower level are broken down into one dimensional strings, but I understand that only a straight line can be one dimensional, how are these loop like strings still said to be one dimensional ?
Maybe this will help:
In mathematics, the dimension of an object is an intrinsic property independent of the space in which the object is embedded. For example, a point on the unit circle in the plane can be specified by two Cartesian coordinates, but one can make do with a single coordinate (the polar coordinate angle), so the circle is 1-dimensional even though it exists in the 2-dimensional plane.
Take a string and distort it, the position of the points on the string are into one to one correspondence with the stretched string, thus there is one string coordinate that describes the position of the points on the string, whatever its shape. Given the shape, one can find a mathematical function that will reduce the two or three dimensional description possible for a distorted string's points into one variable, as with the simple example of a circle above.



 (2)
(2)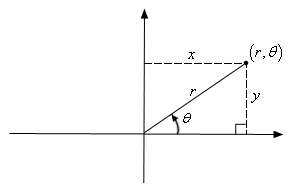
 Irrespective of the coordinate system the line will remain one-dimensional.
Irrespective of the coordinate system the line will remain one-dimensional.