0
$\begingroup$

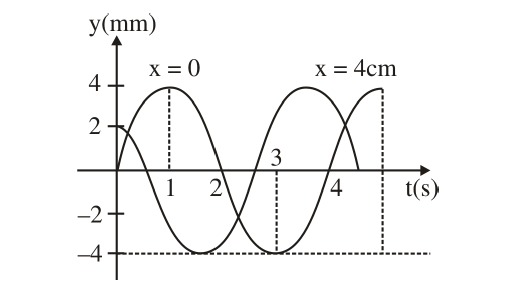
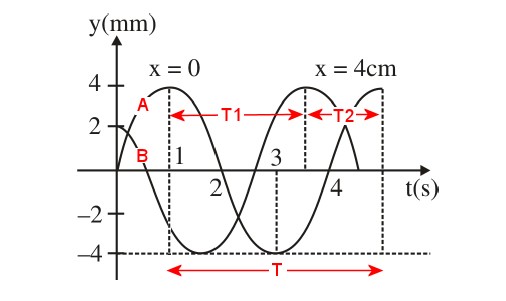
enter image description here

A sinusoidal wave is propagating along a stretched string that lies along x-axis. The wave is moving in +x-direction. Figure shows the graph of transverse displacement of particles of the string at x = 0 and x =4 cm as a function of time. The particles at x = 0 and x = 4 cm are within one wavelength of each other

I want to find the phase difference between particles at x=0 and x=4, and I think the phase difference should be either 7π/6 or 5π/6 ,how can we determine which of these is the correct phase difference and with what reasoning?

Improve this question
$\endgroup$
1
  • $\begingroup$ You should add why you think it is 5/6 or 7/6 pi. $\endgroup$
    – JEB
    Commented May 2 at 2:16

1 Answer 1

Reset to default
0
$\begingroup$

Looking at the graph below, the phase angle is defined as, $\phi_1 = \dfrac {T1}{T}\cdot 2\pi$, or $\phi_2 = \dfrac {T2}{T}\cdot 2\pi$, and note that $\phi_1+\phi_2 = 2\pi$.

enter image description here

The choice of which phase angle to use is often arbitrary.

So you could say that:

whatever happens at position $x=0$ (graph $A$) occurs later by a time $T1$ at position $x=4$ (graph $B$) or

whatever happens at position $x=0$ (graph $A$) occurs earlier by a time $T2$ at position $x=4$ (graph $B$).

Improve this answer
$\endgroup$
2
  • $\begingroup$ But different choices of Phase difference will then lead to different wavelengths , so how will we decide to which particular wavelength the graph refers to? $\endgroup$
    – Garv Chaudha
    Commented May 2 at 13:44
  • $\begingroup$ The assumption is that the speed, wavength and frequency of the wave on the string do not change. $\endgroup$
    – Farcher
    Commented May 2 at 14:34

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.