Question about boundary condition of electric field

Question

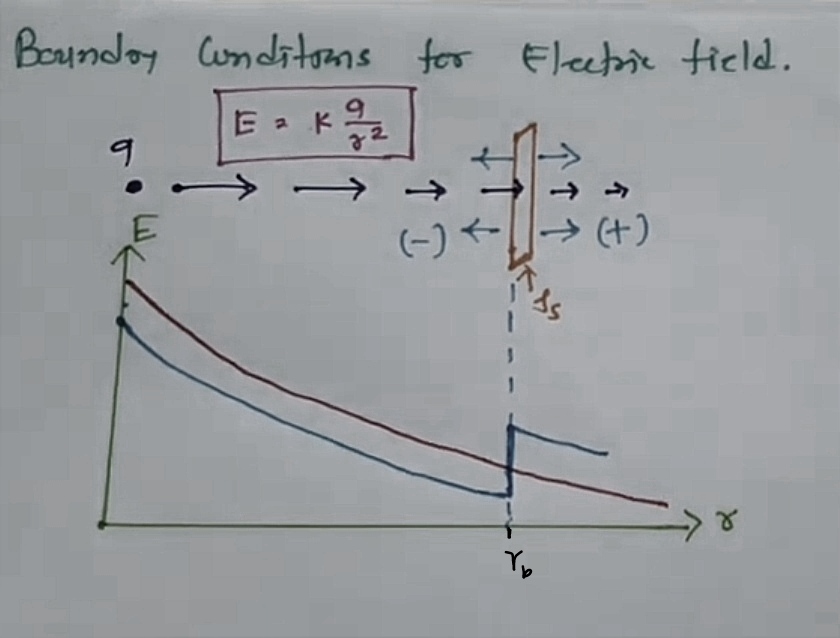
Let us consider a positive point charge placed has electric field $E$, that decreases with distance $r$. When surface charge of $+q$ is placed in field, the electric field will get increase and decrease on right and left side of surface. It is said that 'Electric field is discontinuous by charge density at boundary' called boundary conditions. This is shown in diagram below. My question is that, how the electric field lines of point charge had positive field across the surface charge (inspite of repulsion due to same charge) over this boundary?

Answer 1

I'd guess that the charged plane is supposed to extend indefinitely in all 'sideways' directions, so that the field it gives rise to is uniform and directed normally outwards from the plane. Gauss's law shows the magnitude of that field to be $$E_{\text{plane}}=\frac \sigma {2\epsilon_0}\ \ \ \ \ \ \ \ [\sigma = \text{charge per unit area of plane}]$$ The blue line on your graph shows the resultant field due to the point charge and to the charged plane (except that the field should get indefinitely large next to the point charge). The condition for the resultant field everywhere to the right of the point charge to be directed to the right is simply $$\frac {q_{\text{point}}}{4\pi{\epsilon}_0 r_1^2}>\frac {\sigma}{2\epsilon_0}$$ in which $r_1$ is the distance from the point charge to the plane.

Answer 2

My question is that, how the electric field lines of point charge had positive field across the surface charge (in spite of repulsion due to same charge) over this boundary?

There might be confusion between a dielectric charged plane vs. a charged metallic sheet. The difference between a dielectric and a metal is that in the latter the charges are mobile, so, indeed, the repulsion would make them move and compensate the electric field of the point charge nearby - the resulting electric field then can be calculated using the method of images. Note that the charge distribution in the metal sheet is no more uniform.

In case of a dielectric, with a given surface charge density, the charges do not move - so the repulsion is irrelevant. We simply calculate the field as a sum of the field created by the point charge and the uniform charge distribution on the surface (superposition principle.)

The two cases are easily confused in absence of a point charge or any other external field, since, for symmetry reasons, the charge on a metallic sheet spreads uniformly, and the two cases become indistinguishable.

Related:
Electric field for point charge in a smoothly-varying dielectric?
How can electric field be constant everywhere due to infinite plane sheet?

Answer 3

This is more or less a restatement of Philip’s answer. Hopefully, in a more accessible way for you.

First, the lines radiating outward from q aren't field lines per se. They are actually a graphical representation of E's vector field along the axis.

Second, the vector field drawn is for $E_q$ only. It's easy to see that the magnitude of vector field is dropping off with distance prior to passing through the plane. As you point out if the surface is a conductor, the magnitude of the E-field would be zero at the plane (and on the other side due to shielding the effect of conductors).