How can electric field be constant everywhere due to infinite plane sheet?

Question

Why electric field lines through infinite plane sheet straight and constant everywhere I am not getting it why don't it change with distance can someone explain it omitting Gauss's law proof, I will appreciate if someone can explain it intuitively.

Answer 1

Why the field is perpendicular to the sheet?
Symmetry says that the lines should be perpendicular to the sheet. Indeed, if, e.g., they were inclined at some angle, then one could argue that they could be inclined in the opposite direction - because all the orientations about an axis perpendicular to the sheet are equivalent.

Alternatively, one could think of a sheet cut into small pieces, the field due to each of which is like that of a point charge. The field at any point outside the sheet is coming from all the points, and for every field component not perpendicular to the sheet, there is one directly opposite in the perpendicular plane.

Cancellation of fields coming from opposite points (image source):

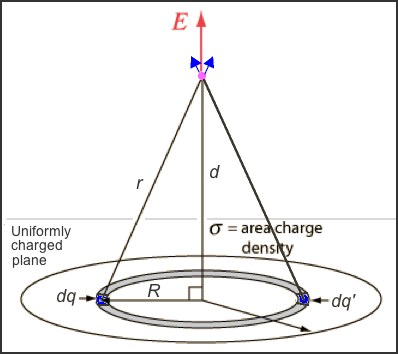
Why the field does not decrease with distance?
Finally, the intuition that the field should decay with distance comes from the experience with point charges or other charged objects that have finite amount of charge. An infinite sheet contains infinite charge density, and can sustain the field infinitely far from itself.

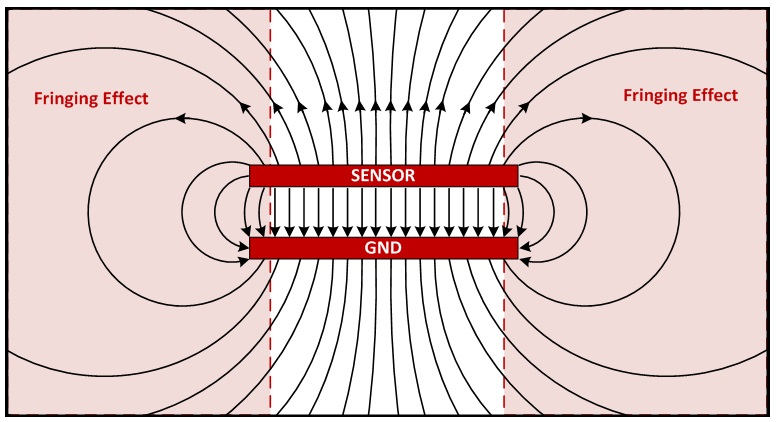
In practice, of course, no sheet is infinite, and the field is nearly uniform only very close to the sheet, where we can neglect effects due to its borders - the fringe effects.

Fringe effects in a parallel plates capacitor (image source): the field is uniform deep inside the capacitor, but is no more uniform towards its edges. Outside the capacitor it resembles the field of a dipole (since we have here two charged plates - positively and negatively charged.)

Answer 2

I will give you an intuitive explanation, that is similar to Huygens principle,

Consider a charge at a point, and for this example consider the Electric field as something flowing from the +ve charge and towards -ve charge, now consider the equi-electric-field surfaces, as you move from one surface to next the value of electric field changes,

Now instead of changing the surface consider the surface being evolved and and value being changed on it. This is now equivalent to a wave-front as in Huygens principle and the instesity of the wave decreases as the surface area of wave front increases,

Now consider an an infinite line charge by drawing the wave front of individual charges and adding it according to Huygens principle, you'll see it taking a shape of cylinder and the surface area of cylindrical wavefront is increasing, so electric field should decrease,

Now coming to your problem, consider and 2d lattice of charges at each point draw the surfaces for them and as per Huygens principle you'll see they form a large surface and as this surface moves away from the sheet, the surface area is unchanged giving you same electric field intensity.