Is there any meaning or statistical distribution associated with the Jacobi's $\theta$ functions?

Question

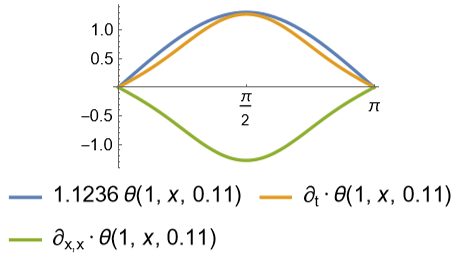
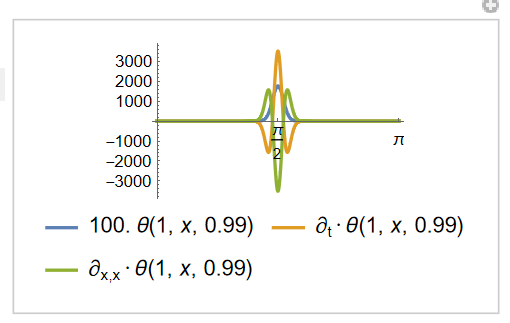
The Jacobi's theta functions

$$\theta_1(0,\tau )=0$$ $$\theta_2(\tau) =\sum_{n\in \mathbb{Z}} q^{(n+\frac{1}{2})^2 /2 }$$ $$\theta_3(\tau) =\sum_{n\in\mathbb{Z}} q^{n^2/2}$$ $$\theta_4(\tau) =\sum_{n\in\mathbb{Z}} (-1)^n q^{n^2/2}$$

and the $\eta(\tau)$ functions were used to build the partition functions in the conformal field theory, which, in the rational CFTs, often corresponding to the condensed matter systems.

However, the $\theta_i(\tau)$ functions were not as intuitive as the Fermi–Dirac distribution or the Bose–Einstein statistics, i.e. $1+\exp((\mu-\epsilon)/k_B T)$ and $\frac{1}{1-\exp((\mu-\epsilon)/k_B T)}$, built up from the typical terms such as $\exp((\mu-\epsilon)/k_B T)$.

Is there any meaning or statistical distribution associated with the Jacobi's theta functions?

Answer 1

It all comes from the (not so) mysterious fact, that the Jacobi theta functions are perodic functions that obey the diffusion PDE wrt to the parameter and the argument. So they are used to interpolate parametrically between a periodic $\delta$-distribution as start distribution and the constant function as the equilibrium limit.

For experiments in Mathematica

    Manipulate[
Plot[{EllipticTheta[n,x,t]/( 1-t),
      4 *t *Derivative[0,0,1][EllipticTheta][n,x,t],
      Derivative[0,2,0][EllipticTheta][n,x,t]},
    {x,0,\[Pi]}] ,
 {t,Range[0.11,0.99,0.11]},{n,Range[4]}]

By the fundamental fact, that the solution of the diffusion equation in a box with any start distribution leads to thermal equilibrium for time $t\to \infty$, while the same equation with imaginary time is the Schrödinger equation decribing time-oscillating eigen modes, makes the theta functions the ideal basis for studying quantum mechanical thermal distributions, according to the famous statement of statistical quantum field theory, that the inverse temperature is imaginary time and theta series on the cylinder are what are Gaussians with complex argument for quantum statistics of fields on $\mathbb R^2$ .

Answer 2

Massive non-relativistic particle
Not sure what the answer is exactly looking for and some notations are not explained. However, what readily pops up in my mind is a particle in a square well, which has energy $E=\alpha n^2$, so that, if coupled to a bath or if a part of a thermodynnamic ensemble, such particle would have partition function $$ Z = \sum_{n=0}^{+\infty}e^{-\beta\alpha n^2}. $$ This also applies to a more general (but somewhat artificial) case of plane waves with quadratic dispersion quantized by periodic boundary conditions.

Coulomb blockade
Another example could be Coulomb blockade, routinely realized in quantum dots, nanograins and other materials, where the energy of the charges stored is given by $$ E_Q = \frac{(Q-Q_0)^2}{2C}=\frac{e^2(n-n_0)^2}{2C}, $$ where $C$ is the capacitance, $n$ is the number of charges (electrons) in the grain/dot, and $n_0$ is a rational number representing the gate potential, typically taken between $-1/2$ and $1/2$.

Holes in a semiconductor
If one is interested specifically in having positive sign in the exponent, applying the periodic boundary conditions or quantization in a box to holes in semiconductor fits the bill, as the dispersion relation (in the effective mass approximation, near the top of the valence band) is $$ E(p)\approx E_v-\frac{p^2}{2m_h}. $$