Radiation through optical windows

Question

Edit: I have tried answering this a different way since posting this, see my newer answer below.

Problem I'm interested in solving: How much radiative heat load is entering the system through optical windows (in SI units - Watts)? (see the images below)

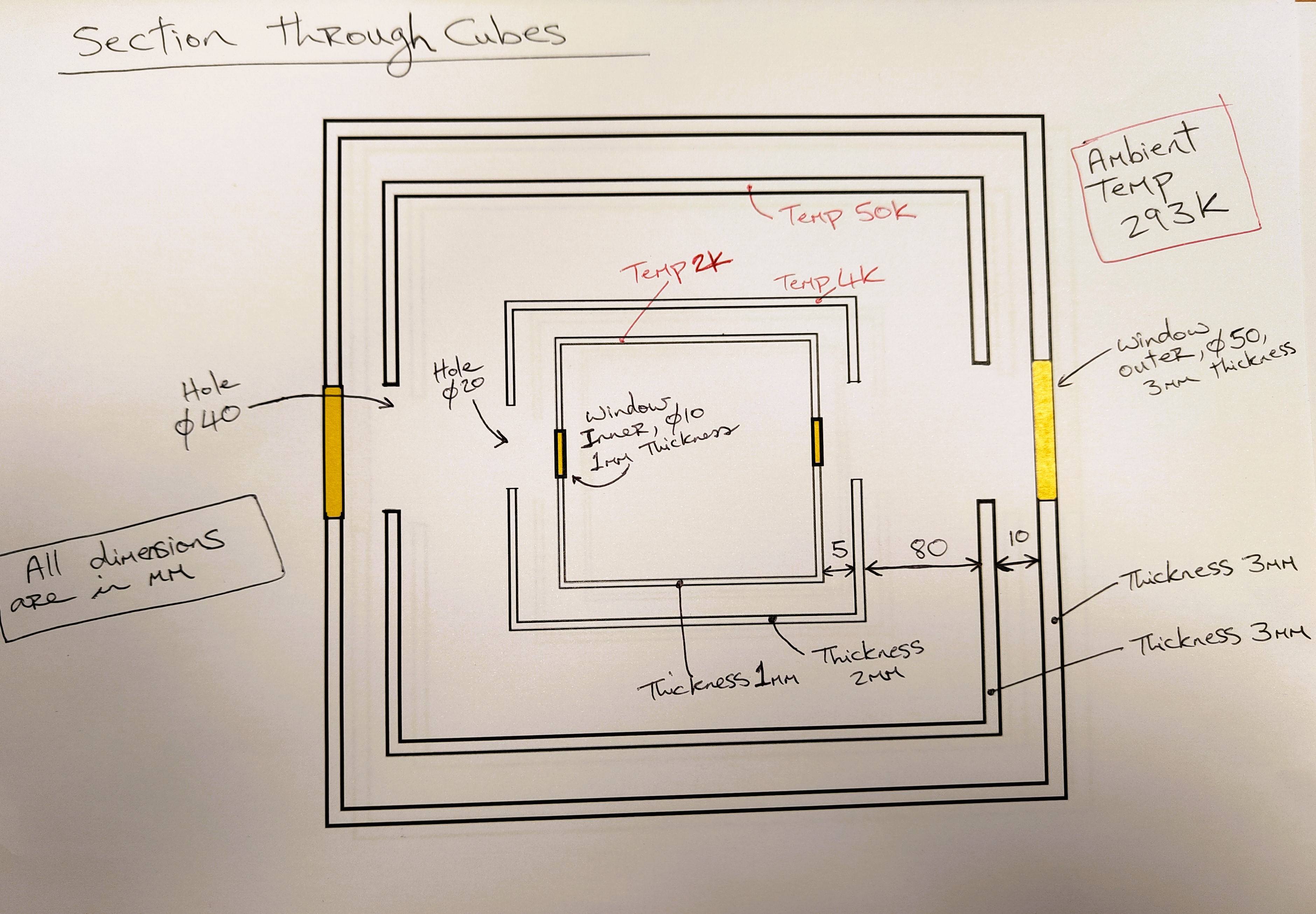
I have tried to break the problem into its most basic components. We have a series of 4 boxes, one inside the other.The boxes themselves are radiation shields and so should have very little radiation entering through them. Can consider it negligible as the windows are the focus of the question.

They are not in thermal contact with each other and there is a gap between each one (in reality the top of the boxes are connected to something else and not floating in air!).

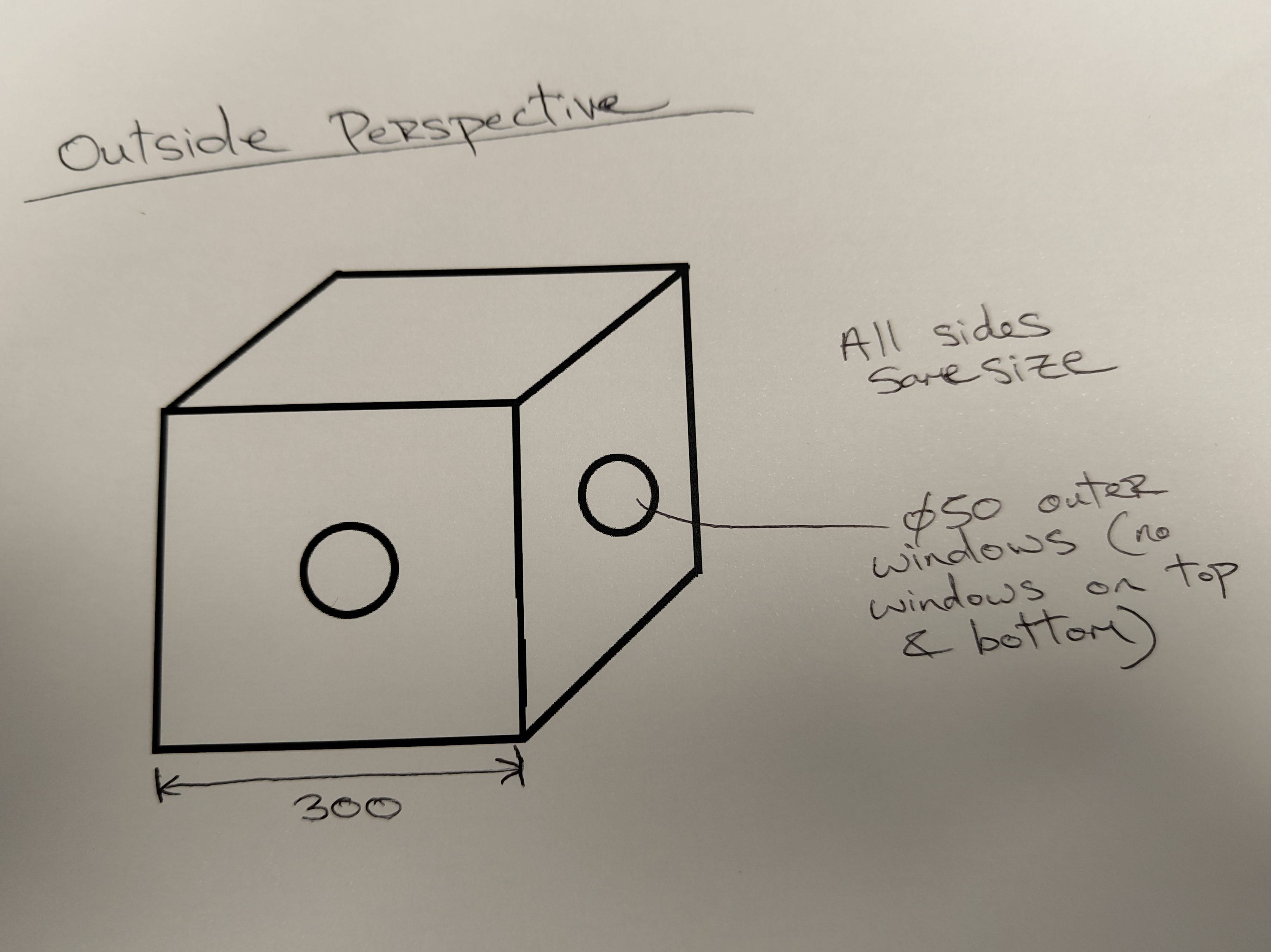
The outer box has a window on each side (except top and bottom). The diameter of the windows are 50mm and 3mm thick.

The 2nd and 3rd boxes have holes in each side to allow light to travel through (eg. if you wanted, you could shine a laser through and into the inner box).

The inner box also has windows on each side. The diameter is 10mm and the thickness is 1mm. Each box is at a different temperature.

The outer box is exposed to ambient temperature around it (293K is a fine approximation). The 2nd box is at 50K. The 3rd box is 4K. The inner box is 2K. There is a vacuum inside the boxes.

How many watts makes it through the 2nd window (where I might have a sample of some sort)?

Some other info: The windows are in thermal contact with the boxes and thus the same temperature. The boxes are aluminium and the windows are Zinc Selenide. However, any material could be used for the calculation. I want to know how it was done and what properties of the material were used. For Zinc Selenide, I was using an emissivity of $0.88$. It transmits $75$ percent of light (approximation) between $0.6\mu m$ and $21\mu m$ wavelength range.

To try solve this, I assumed (perhaps incorrectly) that only the windows need to be considered. I tried to use the Stefan-Boltzman law but the answer I get seems suspiciously low. Window 2 answer is tiny. I feel I'm missing some key consideration needed to solve the problem.I tried to put all info in the drawing below even if I'm not using everything in the calculation.

Stefan-Boltzman Law (with transmissivity term): \begin{equation} P = \tau \epsilon \sigma A (T_1^4 - T_2^4) \end{equation} Emissivity $\epsilon = 0.88$

Stefan-Boltzman Constant $\sigma = 5.67 \times 10^{-8}$

A = Area

Transmissivity $\tau = 0.75$

Window 1 (Outer)

Radius = $\phi / 2 = 0.05 / 2 = 0.025 m$

Area = $\pi (0.025)^2 = 0.00196 m^2$

\begin{equation} P_1 = \tau \epsilon \sigma A (T_1^4 - T_2^4) = (0.75) (0.88)(5.67 \times 10^{-8}) (0.00196) (293)^4 = 0.541 W \end{equation}

Window 2 (Inner)

Radius = $\phi / 2 = 0.01 / 2 = 0.005 m$

Area = $\pi (0.005)^2 = 0.000079 m^2$

\begin{equation} P_2 = \tau \epsilon \sigma A (T_1^4 - T_2^4) = (0.75) (0.88)(5.67 \times 10^{-8}) (0.000079) (2)^4 = 4.73 \times 10^{-11} W \end{equation}

At this point I'm stuck as it seems something is wrong. Can anyone point me in the right direction?

Answer 1

Your second approach is much better. If you have enough light power to make negligible the thermal radiation of the inner shield (including the inner window), then the light power transmitted to the sample is all you care about. Of course, to calculate the effect of that light power, i.e. how much it heats up the sample, you'd need to know how much the sample absorbs, how much it re-radiates, and the quality of thermal contact to the sample mount. In these situations where you have heat coming in from one side (optical) and flowing out the other (to the cold finger), there will be a thermal gradient across the sample, and it behooves you to measure temperature as close to the sample as possible (if not directly on the sample and/or directly via some property of the sample).

If the solid angle subsumed by the inner window, $\Omega$, is equal to or smaller than the solid angle of the outer window, then you can just multiply the incident power, $P_0$, by the transmittances and (twice, for two windows) the solid angle ($2 T^2 P_0 \Omega$). This would be appropriate for thermal radiation which is coming from all angles outside.

But what purpose are windows except to illuminate the sample? If you are providing some illumination $P_0$, then that may be both larger in power than the thermal background and smaller in solid angle than the windows. Then it's just $T^2 P_0$, where T is the transmittance of your illumination spectrum through one window (which may differ from the blackbody spectrum).

Answer 2

I believe I was approaching this in the wrong way and the Stefan-Boltzman equation is not what is needed here. I decided that I should have used the incoming light intensity along with the known transmission rate. For example, looking at the first window and choosing 1000W/m^2 as the incoming light intensity (purely as an example).

Window 1 (Outer):

Radius: $r_1 = \phi_1 / 2 = 0.05 / 2 = 0.01 m$

Area: $ A_1 = \pi (0.01)^2 = 0.000314 m^2$

Assuming $1000 W/m^2$ light intensity incoming to the outer window and a tranmittance factor of $T=0.75$:

Light intensity through window 1: \begin{equation} I_1 = T \times I_{0_1} = 0.75 \times 1000 = 750 W/m^2 \end{equation}

Radiative power through window 1: \begin{equation} P_1 = I_1 \times A_1 = 750 \times 0.000314 = 0.2356 W \end{equation}

The light intensity emitted from the first window should equal to the light incident on the second window ($I_1 = I_{0_2}$). I'm assuming there wouldn't be much loss (I would appreciate any information on the validity of this assumption).

Window 2 (Inner):

Radius: $r_2 = \phi / 2 = 0.01 / 2 = 0.005 m$

Area: $ A_2 = \pi (0.005)^2 = 0.000079 m^2$

Light intensity through window 2: \begin{equation} I_2 = T \times I_{0_2} = 0.75 \times 750 = 562.5 W/m^2 \end{equation}

Radiative power through window 2: \begin{equation} P_2 = I_2 \times A_2 = 562.5 \times 0.000079 = 0.0444 W \end{equation}

If anyone has any options on the validity of this approach, I'd be interested to know.