One-dimensional tight-binding model with two different hoppings in the limit the hoppings are equal

Question

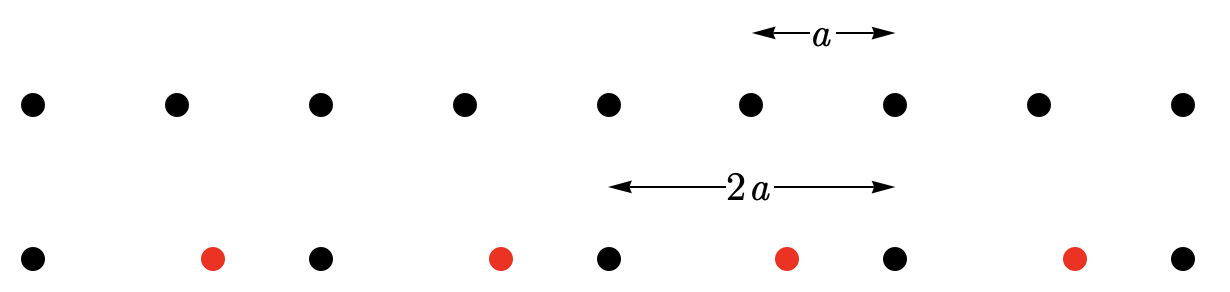
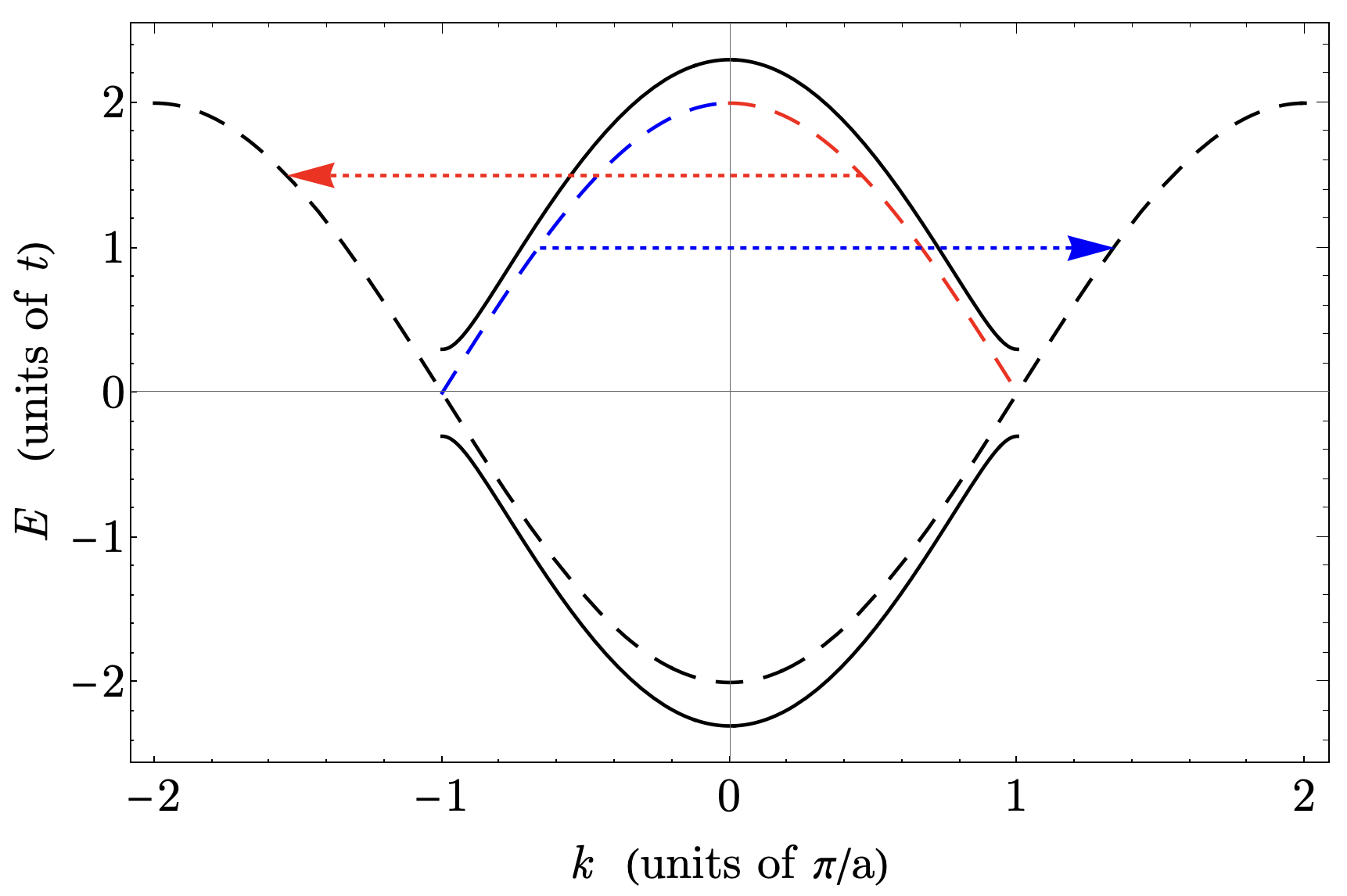
I've recently been watching this lecture series on Condensed Matter. The part I'm currently on covers band theory for the tight-binding model in a few different scenarios. We covered two different models in one-dimension. The first had a Hamiltonian given by $$H = -t \sum_i c_i^\dagger c_{i+1} + c_{i+1}^\dagger c_i,$$ where the sum runs over the $N$ sites in the one-dimensional chain with periodic boundary conditions and $c_i$ is a creation operator for an orbital at site $i$. We solved this model and got to the single-particle eigenenergies $$E(k) = - 2 t \cos(k a),$$ where $a$ is the lattice spacing and $k = \frac{2\pi n}{N a}$, with $n = 0, 1, \ldots, N-1$.

The second model had two sites per unit cell. We have $N$ unit cells and have the Hamiltonian $$H = - \sum_i t_1(c_{i,A}^\dagger c_{i,B} + c_{i,B}^\dagger c_{i,A}) + t_2(c_{i-1,B}^\dagger c_{i,A} + c_{i+1,A}^\dagger c_{i,B}),$$ where $A$ and $B$ label the two different sites per unit cell. The single-particle eigenenergies we found this time were $$E(k) = \pm \sqrt{t_1^2 + t_2^2 + 2 t_1 t_2 \cos(ka)},$$ where $a$ is the size of one unit cell and $k = \frac{2\pi n}{N a}$, $n = 0,1, \ldots, N_1$ (to the best of my knowledge).

What I find weird is that I would expect that the limit $t_1 \to t_2$ in the second model should reduce it to the first model with $t = t_1 = t_2$, albeit with $2N$ sites instead of $N$. However, that is not what happens, since the second model always has two energy bands, while the first has only one. My question is: why doesn't the second model reduce to the first one in the limit $t_1 \to t_2$?

Answer 1

Here's a way in which we can make sense of the limits in a physical situation in which the limit $t_1\to t_2$ should yield the first Hamiltonian. We could think of the atomic species as being the same, but there being two different crystal structures. The first is one in which every atom is a distance $a$ apart. In the second, we shift every other atom some small distance $\alpha a$ to the right, with $\alpha < 1$. This would then introduce alternating hopping strengths due to the differing distances between adjacent atoms, but in the limit as we take $\alpha \to 0$, this becomes the original lattice. The lattices are illustrated below.

Crucially, we have doubled the size of the unit cell from $a$ to $2a$. This makes it so that in the second dispersion relation, we should take $a\to 2a$, yielding $$ E(k) = \pm \sqrt{t^2 + t_2^2 + 2 t t_2 \cos(2ka)}\,, $$ where I have set $t_1=t$. In the limit as $t_2\to t$, the above becomes $$ E(k) \to \pm 2t \cos(ka)\,. $$ It seems like this is a problem, because we get two branches (the plus and the minus). However, we haven't taken into account the fact that the first Brillouin zone must double in size in this degenerate limit, because the size of the unit cell gets cut in half ($2a\to a$) in this limit. Thus, we need to "unfold" the dispersion relation out of the reduced zone scheme picture we needed in going from a unit cell of size $a$ to a unit cell of size $2a$.

This is illustrated below. The black dashed curve is the dispersion relation for the simple case of the crystal structure with equal inter-atomic spacings $a$. The solid black curve is the dispersion relation for the diatomic lattice with $t_2/t = 1.3$. The dashed black curve is the dispersion relation for the monoatomic lattice, and we plot it over a larger region because the Brillouin zone is twice as large! Then, we can see that the positive branch of the limit dispersion relation (i.e., $+2t\cos(ka)$) seems as if it's a second branch of the dispersion relation, but really we need to shift those over by reciprocal lattice vectors (of the diatomic lattice) to where they should be in the first Brillouin zone of the monatomic lattice.

Thus, we can take the limit and get the same system, but you need to make sure that the unit cell sizes are consistent and that you are thinking carefully about the actual periodicity of your system and hence the correct size of the first Brillouin zone.

Answer 2

Taking your second equation an setting $t_1,t_2\to t$, we get $$E(k)=\pm t \sqrt2 \sqrt{1+\cos(ka)}=\pm 2 t \cos\left(\frac{ka}2\right)$$

where I used $1+\cos x = 2 \cos x/2$. The $k/2$ comes because $N$ is different by a factor of 2.

However notice that there is still a $\pm$. This happens because the equation used is not right, it was diagonalized as if I was handling a two-species unit cell, so we get a duplication of the bands.