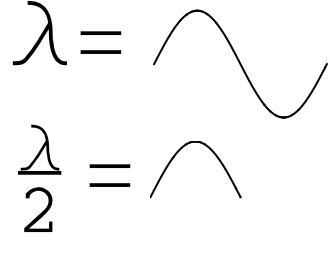I'm reading my textbook and it says the distance between two successive nodes is equal to $\frac{1}{2} \lambda$ in standing wave.
If $\lambda$ here means the wavelength of the standing wave shouldn't it be:
when there are two nodes: $L=\lambda$
when there are three nodes: $L=\frac{1}{2} \lambda + \frac{1}{2} \lambda$
when there are three nodes: $L=\frac{1}{3}\lambda + \frac{1}{3}\lambda + \frac{1}{3}\lambda$
Does $\lambda$ here mean anything?
As Danu said, the wavelength $\lambda$ corresponds to the length where the wave starts reproducing itself. See the picture attached
Does λ here mean anything?
Yes, it means, in this context, precisely what it usually means: $\lambda$ is distance over which the wave completes one (spatial) cycle, i.e., the distance over which the argument changes by $2 \pi $
Look at the 2nd drawing from the top. It is clear that exactly one cycle completes in precisely a length of $\lambda$
Now, recall that the sine function is zero when the argument is zero and an integer multiple of $\pi$ so, the sine function passes through zero twice each cycle.
Because of the boundary conditions (zero displacement at the ends), integer multiples of one-half of a cycle can "fit" between the ends which leads to the condition that the spatial variation of the standing wave must be of the form:
$$f(x) = \sin(\frac{n \pi}{L}x) = \sin(\frac{2 \pi }{\lambda_n}x) $$
which immediately leads to:
$$\lambda_n = \dfrac{2L}{n} $$
In other words, for each value of $n$, there is an associated wavelength, $\lambda_n$. Granted, the drawing in the book doesn't make this particularly clear.
So, for $n = 1$, the wavelength $\lambda_1 = 2L$ or, as the 1st diagram shows $L = \dfrac{\lambda_1}{2}$
For $n = 2$, the wavelength $\lambda_2 = L$ as the 2nd diagram shows.
For $n = 3$, the wavelength $\lambda_3 = \dfrac{2L}{3}$ or, as the 3rd diagram shows, $L = \dfrac{3\lambda_3}{2}$
And so on...
These waves are described by sinusoidal functions. $\lambda$ is the wave length of the wave, so that means the phase of the sinusoidal function will increase by $2\pi$ when the distance increases by $\lambda$. If you know about sinusoidal functions, can now show that these have exactly two zeros (or nodes) per wave length. These are equally spaced, so there's always $\frac{1}{2}\lambda$ between the nodes. If you count both the starting and end point of the standing wave as nodes too, a standing wave of length $L=\frac{n\lambda}{2}$ (with $n$ an integer) will always have $n+1$ nodes.
The reason behind why it is $1/2\lambda$ instead of $\lambda$ is
a standing wave was created by the superposition of the wave moving to the left $y_1 = A sin(kx-\omega t)$ and the wave moving to the right $y_2= A sin(kx+\omega t)$, and that was also how the node(s) in between 2 nodes are formed.
This video here explains it the best.
