What is the precise definition of unification of fields (in classical and quantum mechanics)?
In general, does unification of a field mean that we can write both of them at both sides of an equation (like Maxwell's laws)? Or does it mean that one of them can produce the other (like $E$ and $B$)?
Is there any intuitive explanation of how electroweak unification works? Like an electric charge will feel a weak field or that a flavoured particle will produce a weak field?
-
5$\begingroup$ Electroweak interaction is not a unified interaction. You have $2$ different coupling constants, and $2$ different Lie groups $U(1), SU(2)$, plus a rotation and Higgs phenomenon. To have a unified theory, you need one Lie group, for instance $SO(10)$, and one coupling constant. $\endgroup$– TrimokCommented Sep 17, 2013 at 16:13
-
2$\begingroup$ @Trimok I thought electroweak was U(1)xSU(2). And how can electroweak not be a force? Wikipedia says: "on the order of 100 GeV, they would merge into a single electroweak force". $\endgroup$– jinaweeCommented Sep 17, 2013 at 16:30
-
1$\begingroup$ @jinawee : OK. I have not been precise enough. Before the electroweak symmetry breaking, you have not the rotation and Higgs phenomenon , you have a $U(1)*SU(2)$ symmetry, but you have still $2$ different coupling constants, one for each symmetry. You are not in a situation where you have only one coupling constant, like in GUT theory, above the GUT energy. $\endgroup$– TrimokCommented Sep 17, 2013 at 16:51
-
1$\begingroup$ @Trimok So your definition of unification is to have a single coupling constant. I suppose that this is only valid in QFT context. Or is it valid in EM if you consider $\epsilon_0$ and $\mu_0$? $\endgroup$– jinaweeCommented Sep 17, 2013 at 16:58
-
1$\begingroup$ $\epsilon_0, \mu_0, c$ may be set to $1$ in some appropriate unit system. There is only one dimensionless coupling constant $\alpha = \frac{e^2}{4 \pi \epsilon_0 \hbar c}$ $\endgroup$– TrimokCommented Sep 17, 2013 at 17:21
2 Answers
"Unification" refers to explaining two sets of phenomena (theories) which were previously urelated, and combining them into a single cohesive description.
Eg: electricy and magnetism unified into electromagnetism.
While those two sets of phenomena could be approximately treated (in one regime) by neglecting the other, it is important that the two phenomena are coupled to each other in some regime. Otherwise, any unification would be superficial since the two kinds of physics would be happening alongside each other, but separately.
If you do manage to unify two formerly separate physical theories, then you would see equations involving both of them, like Maxwell's equations involve both E and B. And since they are coupled, one can affect the other.
As for electroweak unification, the gauge group is non-abelian. So the notion of charge is "souped up" into representations of the Lie group (or Lie algebra). So the technically correct statement would be that there exist particles/fields which would be influenced by both electromagnetism and the weak force. One can work out the details of the Higgs mechanism of how the unified electroweak theory "breaks down" into electromagnetism and weak force, and everything fits well with observations.
Unification in physics is used differently in classical physics than in the quantum regimeof elementary particles.
Unifying electricity and magnetism became necessary when functional measured relations appeared which connected the motion of charges with the magnetic field and the magnetic field with the motion of charges. The Biot-Savart law and Ampere's law . Maxwell unified these observations into an electromagnetic theory with a lot of predictive power and which explained radiation elegantly.
Unification at the elementary particle theory level came after the accumulated observations of a plethora of resonances in scattering particles against each other. The masses and spin states of these resonances displayed a surprising symmetry and could be orgnised into SU(3) multiplets, octets and decuplets. The quark model for the nucleons and mesons arose from these experimental observations .
Finally these led to the standard model for particle physics: SU(3)xSU(2)xU(1). If you notice in the diagrams, for example
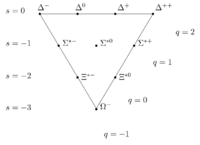
The S = 3⁄2 baryon decuplet
The symmetry unfolds with the highest masses at the lower level, it is the mass that is separating the various isospin multiplets:
The first Omega baryon discovered was the Ω−, made of three strange quarks, in 1964. The discovery was a great triumph in the study of quark processes, since it was found only after its existence, mass, and decay products had been predicted by American physicist Murray Gell-Mann in 1962 and independently by Israeli physicist Yuval Ne'eman
The group symmetries of a lagrangian, in the standard model SU(3)xSU(2)xU(1) which unifies all three interactions, weak electromagnetic and strong, they are called symmetries because in principle calculating the results by operating on the wave function of the model, these should be invariant to the symmetries of the group: measurements of crossections, lifetimes etc. This is the ideal case where each multiplet represents all the particles assigned to it and has a mass of zero. An approximation with zero mass would be true for the very high energies available in the beginning of the Big Bang, for example. the range of measured masses a hundred GeV or so would be approximately zero at those energies.
In the real world, the fact that the decuplets and octets etc are occupied by different masses tells us that the symmetry is broken at the energies we live in, and the particles get masses. In order to accommodate this experimental observation the Standard Model incorporates the mechanism of spontaneously broken symmetry with the Higgs field. This gives masses to the intermediate gauge bosons by breaking the symmetry and giving masses to all elementary particles too.
I hope I have replied to the firs two subquestions.
Is there any intuitive explanation of how electroweak unification works? Like an electric charge will feel a weak field or that a flavoured particle will produce a weak field?
The Z and W are the exchanged gauge bosons in weak interactions and the photon in electromagnetic ones. As the symmetry is broken at our energies it is only through higher order Feynman diagrams that there will be an effect, but the difference in strength of the two interactions is orders of magnitude; effectively if the electromagnetic can happen it will be the fastest and first and will overwhelm the weak. The weak can appear if the electromagnetic is forbidden, as in the different decays of the charged pions versus the neutral one.
