Different version of conformal Ward identity

Question

In the book by Di Franceso, Mathieu, Senechal, equation (5.46) shows that (assuming $\bar\epsilon = 0$) $$ (*) \qquad \langle \delta_{\epsilon, 0} \mathcal{O}\rangle = - \oint_\infty \frac{dz}{2\pi i z}\epsilon(z) \langle T(z)\mathcal{O}\rangle, $$ where on the left is the variation of $\mathcal{O}$ under an arbitrary local conformal transformation with param $\epsilon$. If I understand correctly, this equation starts from the three Ward identities eq (5.32) for the global conformal symmetry, then through some tricks around eq (5.42), an arbitrary conformal transformation parameter $\epsilon$ is brought in to arrive at the above equation, which seems to be much powerful than the original 3 identities.

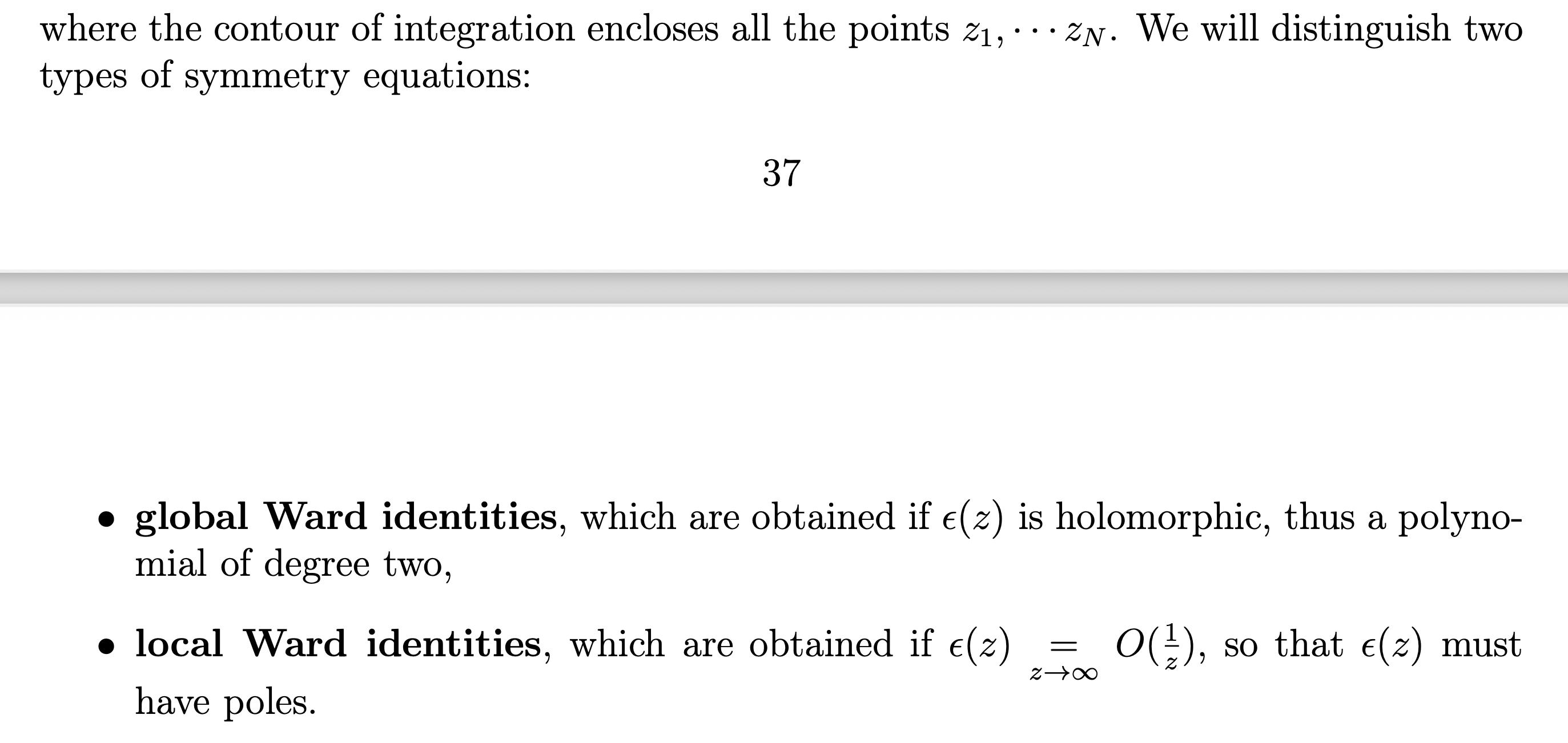
Now, in the note Conformal field theory on the plane by Ribault, equation (2.2.13) writes for any meromorphic $\epsilon(z)$ that has no poles outside $z_1, ..., z_N$, $$ (**) \qquad 0 = \oint_\infty dz \epsilon(z) \langle T(z) \mathcal{O}(z_1, ..., z_N)\rangle, \qquad \text{if } \epsilon(z \to \infty) = O(z^2) \ . $$

In the two Ward identities, the right hand side looks the same, but the left do not.

I wonder

(1) Is the $(*)$ equation more powerful than the original Ward identities eq (5.32) for global conformal symmetry?

(2) Are the two statements $(*), (**)$ the same, or complimentary, or one is the special case of another?

In other words, what is the most general $\epsilon$ such that $\langle\delta_\epsilon \mathcal{O}(z_1, ..., z_N) \rangle = 0$ and how to see that? To begin, $\epsilon(z) = a + bz + cz^2$ is a solution, since it corresponds to the global conformal transformations, the "true symmetry of the system". But from $(**)$ it seems to suggest that any things like $\epsilon(z) = \frac{1}{z - z_1} + \frac{3.14}{(z - z_N)^{2023}} + 1000 z^2$ will set $\delta_\epsilon \langle \mathcal{O}(z_1, ..., z_N)\rangle = 0$, but I don't quite understand why.

(3) In Ribault, also considering the two bullets point after $(**)$, it seems that only $\epsilon(z \to \infty) = O(z^2)$ and $\epsilon(z \to \infty) = O(1/z)$ are considered. What about $\epsilon(z \to \infty) = O(z^{1000})$? Is there any thing one can say about such $\epsilon$? (Below is a snapshot)

Answer 1

Your first equation is more general, and can be obtained from the infinitesimal change of variables in the path integral. The second equation is a special case where the integrand has no poles, hence the infinite contour integral is 0. Thus, in my opinion, both are consistent. The second equation simply tells you that $\delta_\epsilon \langle O \rangle = 0$ when $\epsilon \sim z^2$ at $z\to \infty$.

The two equations are complementary. The first one is the important property, and the second one tells you that in some special case, it vanishes. But this is not a simple application of the first equation, because one needs to understand the pole structure of the integrand to claim such a property.