Potential energy of an (electric) dipole is said to be zero when the angle it makes with the applied uniform field is zero yet it experiences torque

Question

My doubt is that I am unable to understand why a dipole would continue to experience a torque due to a field eventhough the potential energy is zero for angle 90⁰ it makes with the field.Like,there is no energy left to use but the dipole still rotates so I guess I might be missing on some physics fundamentals but so far I havent seen the reason mentioned in any books.

Answer 1

The choice of the position of zero potential energy is often chosen to correspond to the position when the force/torque on a body is zero but it does not have to be so.

For example, wherever a body is close to the surface of the Earth it will experience a force due to gravitational attraction of the Earth and yet any position could be chosen as the zero of potential energy of the body/Earth system.

Answer 2

The absolute value of electric potential energy doesn't tell much about the behavior of dipole under a uniform electric field. Only the change in potential in energy holds significance in this case. The external work done to attain the given configuration is not zero. This results in rotation of dipole under electric field. $$\Delta U=|\vec{p}||\vec{E}|cos{\theta_1}-|\vec{p}||\vec{E}|cos{\theta_2}$$ $$\Delta U=|\vec{p}||\vec{E}|cos{0°}$$ $$\Delta U=|\vec{p}||\vec{E}|\not=0$$ • $\Delta U$ = change in potential energy.

• $\theta_1 and \;\theta_2$ are initial and final angle between dipole and electric field. $$\theta_1=0°$$ $$\theta_2=90°$$ Even if the final value is zero but the change in potential energy is not zero. Therefore, if the external torque becomes zero at $\theta_2$, then dipole would still rotate since it has gained some potential energy.

Answer 3

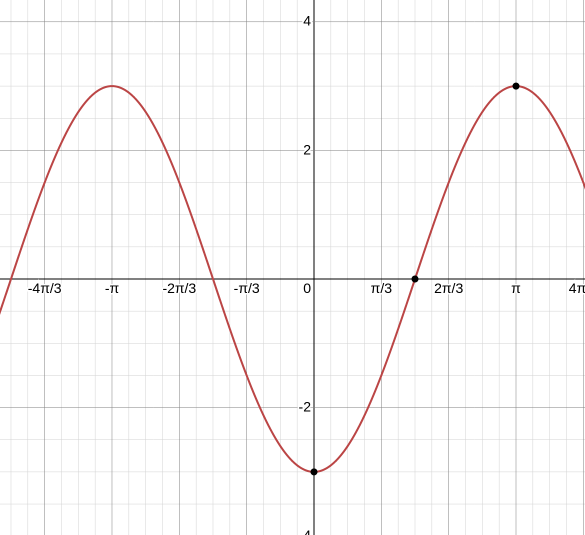
The potential energy of the dipole is given by $$U=-\vec{p}\cdot\vec{E}=-pE\cos(\theta)$$ where the $\theta$ is the angle between the dipole moment and the electric field. $$change\ in\ potential\ energy=-(internal\ work\ done)$$ so we can write this as follows $$dU=-\tau d\theta$$ $$\tau=-\frac{dU}{d\theta}$$ Here is a specimen graph for $U$ w.r.t $\theta$

From the expression of $\tau$ we can say that the torque will be zero only at angles where the slope of $U$ is zero.

What this means is the torque is zero only where the potential energy is either maximum or minimum.

At $\theta=\frac{\pi}{2}$ potential energy is zero but it's neither maximum nor minimum.

Potential energy is maximum at $\theta=\pi$ and $\theta=-\pi$ and minimum at $\theta=0$.

so the torque will be zero at those points and not at $\theta=\frac{\pi}{2}$.