Faraday's Law Induced Electric Field

Question

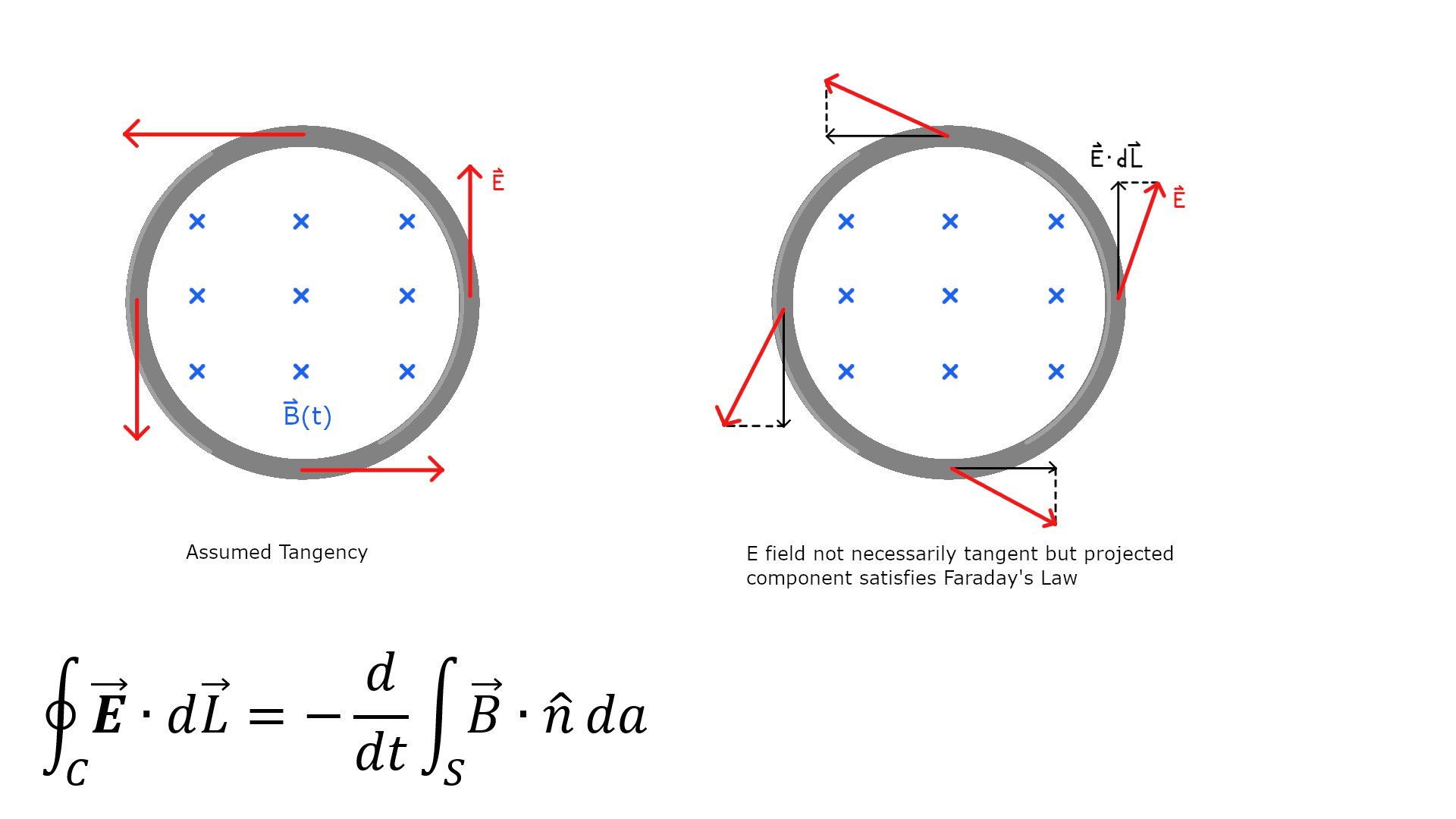
I have been going through A Student's Guide to Maxwell's Equations and am looking at Faraday's Law but am confused about why there always seems to be an assumption that the induced field is tangent to a given loop. I included a diagram to illustrate. The left hand side of the equation is the dot product of the induced electric field and the differential element dL but to me this only specifies that the parallel component is summed and that the actual direction of the field is free to point in any direction so long as there is some non zero component tangent to the ring.

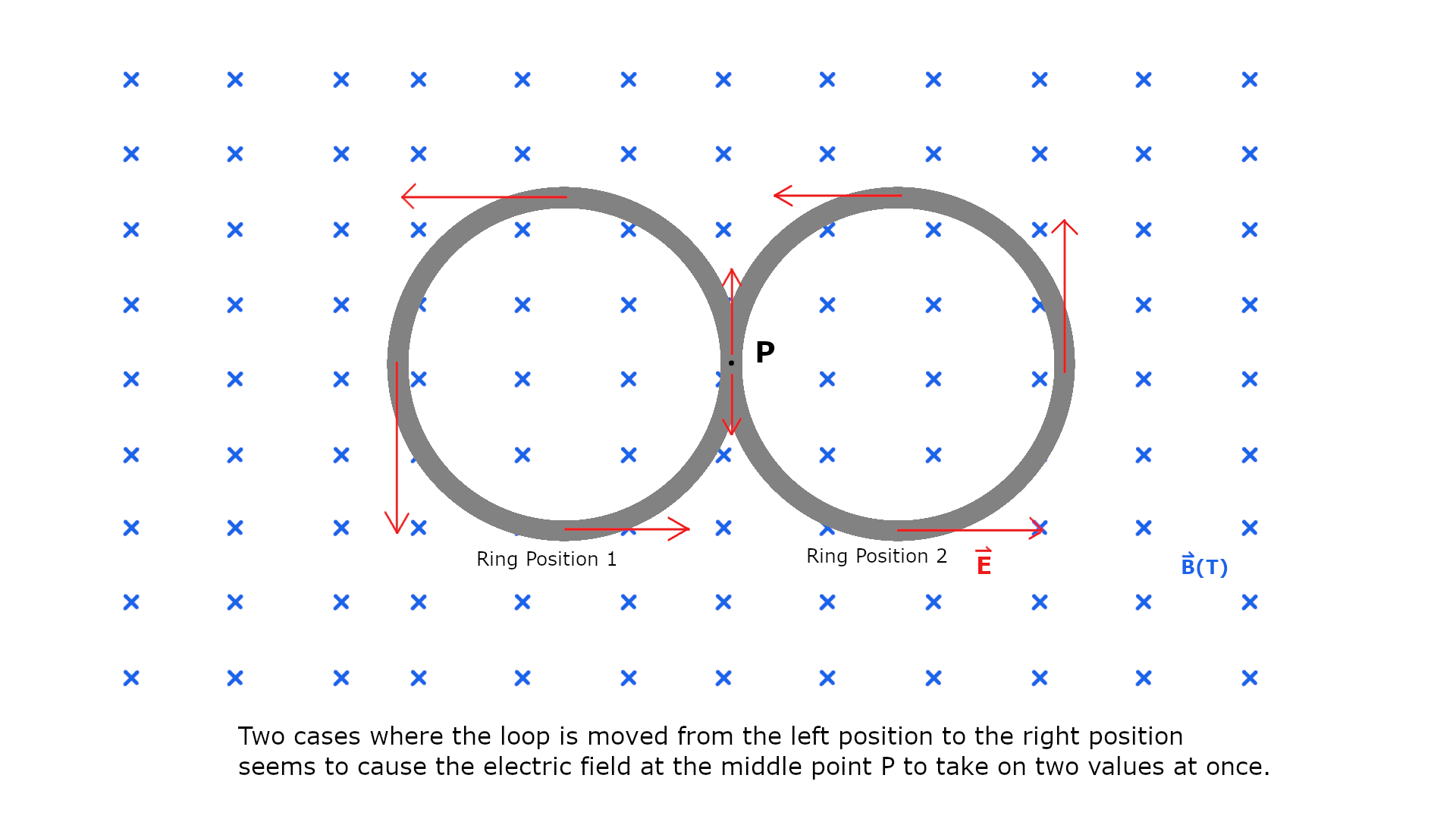
Also another issue I see is that if we consider this same ring in two different positions it seem like we can create contradictory fields where the electric field can take on more than one value at a point.

The overarching question here is how is the induced electric field actually calculated? Is it possible with just Faraday's law or is there additional information required such as the tangency assumption?

Answer 1

If you use Jefimenko's (https://en.wikipedia.org/wiki/Jefimenko%27s_equations), the only sources of electric and magnetic fields at a point are the charges, currents, and their time derivatives on the point's past light cone. The equations ensure Maxwell's Eq are satisfied.

In this viewpoint, a changing magnetic field doesn't induces and electric field (even though this is a handy viewpoint in designing electric motors, metal detectors, etc), rather the sources ensure that $\partial B/\partial t$ is proportional to $\nabla \times E$. The curl of $E$ is not E, of course.

In your example, all that is ensured is the integral of $E$ around the loop , not the value at any point.

Answer 2

In the formula $$\oint_{\mathcal L} \mathbf E \cdot d\mathbf{\ell} = - \frac{d\Phi}{dt} \tag{1}\label{1}$$ where the flux $\Phi$ is defined by $\int_{\mathcal A} \mathbf B\cdot \mathbf {\hat n} dA$ the contour taken in the integral in $\eqref{1}$ is a simple continuous and piecewise smooth rectifiable curve that is otherwise completely arbitrary. The actually induced electric field intensity can be in any relationship with that contour, the terms that are integrated are the projections of the field intensity vector to the local tangent of said curve,$\mathbf E \cdot d\mathbf{\ell}$, hence the requirement for smoothness of the curve everywhere except for a "few" singular points, i.e., corners. Similarly, the surface $\mathcal A$ through which the flux $\Phi$ is defined is to be simple piecewise smooth so that the surface integral exists and its boundary is the integration contour over which the induce emf $\oint_{\mathcal L} \mathbf E \cdot d\mathbf{\ell}$ is defined: $\partial \mathcal A = \mathcal L$.

In other words, you have two fields $\mathbf E$ and $\mathbf B$ that are connected through Faraday's law in such a way that taking any, yes any, simple surface $\mathcal A$ and its boundary $\partial \mathcal A = \mathcal L$ and integrating $\mathbf E$ over $\mathcal L$ you get the negative time rate of the $\mathbf B$ integrated over $\mathcal A$.