Induced current by a dipole?

Question

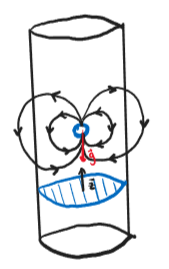
Imagine you have the following situation:

a magnet falling through a hollow metal tube. I want to calculate the equation of movement of this magnet falling. The resulting differential equation should be something like: $$ m y''(t) = -k y'(t) - mg $$

At first glance,you could calculate the induced current in the metal tube. For this, I will calculate the flux around a circular section inside the tube: $$ \phi_m = \iint_C {\mathbf B \cdot d \mathbf A} $$ Using cylindrical coordinates: $$ \phi_m =\frac{\mu_0}{4\pi}\iint_C {\frac{3 \mathbf{\hat{r}}(\mathbf r \cdot \mathbf m) - \mathbf m}{r^3} \cdot \mathbf{\hat{z}} \; rdr d\varphi} = -\frac{\mu_0}{4\pi}\iint_C {\frac{\mathbf m \cdot \mathbf{\hat{z}}}{r^2} \; dr d\varphi} \\ \; \\ \phi_m= -\frac{\mu_0}{4\pi}\int_0^{2\pi} \int_{0}^{R} {\frac{\mathbf m \cdot \mathbf{\hat{z}}}{r^2} \; dr d\varphi} \\ $$ The problem is, that this is the flux across a section on the $xy$ plane, and not all over the infinite planes that intersect the tube along the magnet trajectory.

After $\Delta t$ time, the magnet will cross another circular section with a different velocity, hence the flux has changed. By Lenz law, an electric field will be induced, creating a current, that will create a magnetic field inside the tube. I assume I have to add up all this fluxes contributions, but I don't know exactly how. $$ \phi = \sum_i \Delta \phi_i \rightarrow \phi = \int_\mathcal{V} d \phi $$ How I should find this $d \phi$? Is this line of reasoning correct?

Answer 1

At any given time, we can look at the magnetic flux through a "slice" of the tube (with width $dz$) a distance $z$ from the magnet. Each one of the "slices" has an induced current, meaning that each slice creates its own magnetic field $dB$, which pushes back on the magnet with an infinitesimal force $dF$. By integrating these infinitesimal forces over all possible $z$, you can in principle find the total force on the magnet.

Note: if all you're interested in is the terminal velocity, then it's easier (note: not easy, just easier) to find the velocity $v$ for which the power delivered by gravity to the magnet is equal to the power dissipated as resistive losses in the tube. It's still a pretty involved calculation, though.