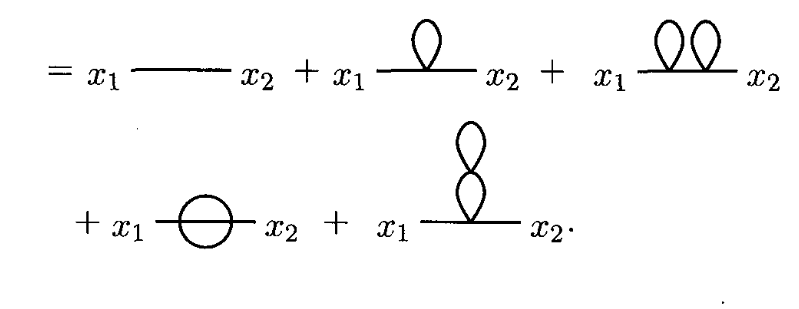0
$\begingroup$

What is the precise definition of a $n$-loop one-particle irreducible ($1$PI) diagram?

For example, consider the following diagrams.

  • Is the first diagram a $0$-loop $1$PI diagram?
  • Is the second diagram $1$-loop $1$PI ?
  • Is the third diagram $2$-loop $1$P (i.e. reducible)?
  • Are the fourth and fifth diagrams $2$-loop $1$PI?

enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ Can any close voter please explain their reason in a comment, thank you $\endgroup$
    – don't train ai on me
    Commented Dec 6, 2022 at 23:21
  • $\begingroup$ Does this help explain the definition: physics.stackexchange.com/q/342994 $\endgroup$
    – hft
    Commented Dec 7, 2022 at 1:36
  • $\begingroup$ Shouldn't this definition just be plainly stated in your textbook? What textbook are you using? $\endgroup$
    – hft
    Commented Dec 7, 2022 at 1:36
  • 1
    $\begingroup$ @doublefelix The reason is: A community-specific reason. The sub-reason is: This question doesn’t meet a Physics Stack Exchange guideline. The sub-sub reason is: Homework-like or check-my-work type question. $\endgroup$
    – hft
    Commented Dec 7, 2022 at 1:42
  • $\begingroup$ @doublefelix Can you explain whether or not that makes sense to you? $\endgroup$
    – hft
    Commented Dec 7, 2022 at 1:44

1 Answer 1

Reset to default
3
$\begingroup$

A 1PI diagram is a connected graph that cannot be disconnected into two pieces by cutting a single line, cf. Wikipedia. In particular its external legs must be amputated, i.e. not part of the diagram.

For the last reason, none of OP's examples are actually 1PI diagrams. [However, if we strip/amputate their external legs, then there is nothing left from the 1st diagram (the free propagator), and the 2nd diagram, the 4th diagram (the sunset diagram) and 5th diagram become 1PI.] Nevertheless they are all connected diagrams of loop-order 0,1,2,2,2, respectively.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.