Recall that for $t$ and $u$ channel the characteristic amplitudes are,
$$\begin{align}\mathcal{M}_t &\propto \frac{1}{t-m^2}\tag{1}\\\mathcal{M}_u &\propto \frac{1}{u-m^2}\tag{2}\end{align}$$
This expression is for a single virtual propagator at tree level. Proceeding with this tree level analysis, recall also that here,
$$\begin{align}s&=k^2\\t+u&=4m^2-s\end{align}$$
So if $s>4m^2$ the above sum is negative and off shell. While it doesn't imply that both $t$ and $u$ are off shell, at least one of them has to be. But note that the definition of $t$ and $u$ are interchangeable for same type of particles (Peskin page 156). So both of them have to be off shell, which implies that a branch cut (or point) cannot exist in their respective amplitudes.
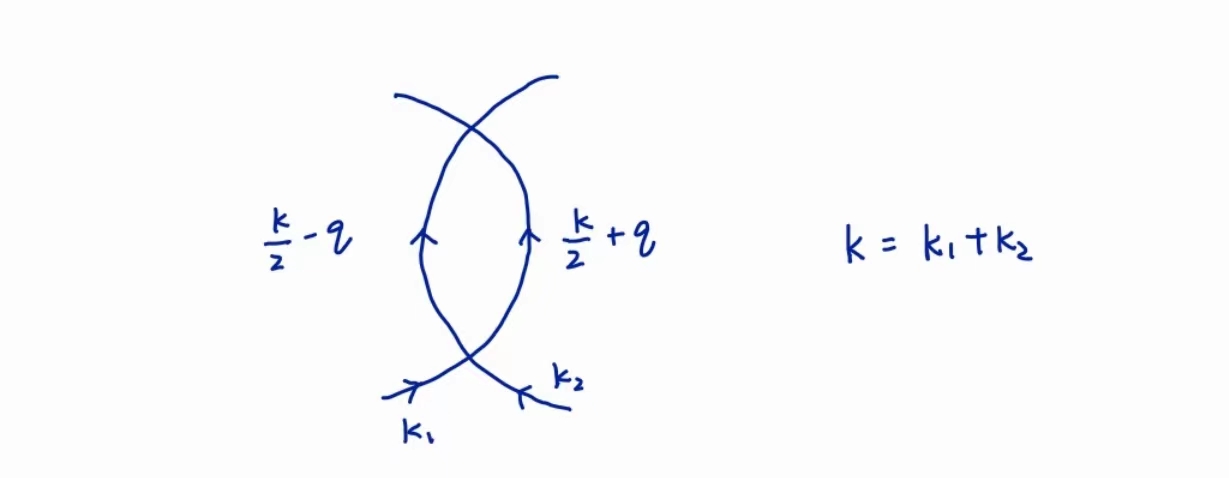
With the intuition worked out for the tree level, we should anticipate something on similar lines for the case of a loop diagram. Thus let us quickly derive the possible expression for the loop integral in the amplitude of $t$-channel. The expression won't be much different from Peskin's Eq. 7.52 where the loop integral for $s$-channel was given as,
$$i\delta\mathcal{M}=\frac{\lambda^2}{2}\int\frac{d^4q}{(2\pi)^4}\frac{1}{(k/2-q)^2-m^2-i\epsilon}\frac{1}{(k/2+q)^2-m^2-i\epsilon}\tag{3}$$
To get to the $t$-channel rotate this diagram clockwise 90 degree and change the $s$-channel ingoing momentum $k_1$ to $-k_1$. How exactly does this correspond to the $u$-channel? Well we know that $t$ expresses the square of the difference between the momentum of the incoming and outgoing legs of the same side. Say in the $s$-channel the outgoing momentum are $p_1$ and $p_2$ (note that we won't require this to be present in the integral as long as they satisfy momentum conservation at the vertex). Then the mentioned rotation and change in the momentum sign would correspond to changing $s\to t$ in all loop integral. The $t$-channel integral would be,
$$i\delta\mathcal{M}=\frac{\lambda^2}{2}\int\frac{d^4q}{(2\pi)^4}\frac{1}{k'/2-q)^2-m^2-i\epsilon}\frac{1}{(k'/2+q)^2-m^2-i\epsilon}\tag{4}$$
where $t=(k_1-k_2)^2=k'^2$. Will $t$ be negative? Surely if $s>4m^2$ which is our threshold, $t$ cannot be too large. In fact in the center of mass system $t$ it looks like $t$ will be zero, but lets take a more general setup taking $k_1^0,k_2^0>m$. In the $s$ channel we keep $k_2^i=-\alpha k_1^i$ where $\alpha$ is some proportionality which will be real and positive. Then consider the following,
$$\begin{align}
(k_1-k_2)^2&=(k_1^0-k_2^0)^2-(k_1^i-k_2^i)^2\\
&=(k_1^0-k_2^0)^2-|\mathbf{k_1}|^2(1+\alpha)^2\\
&=k_2^2+|\mathbf{k_2}|^2-2k_1^0k_2^0+k_1^2-|\mathbf{k_1}|^2\alpha(2+\alpha)\\
&=2m^2-2k_1^0k_2^0-2\alpha|\mathbf{k_1}|^2\\
&< 0
\end{align}$$
So indeed $t$ is off shell. We can then proceed along the same route the book took to calculate the imaginary part of the amplitude. For our purpose we don't need to full analysis, just showing the analytic structure would be enough. Note that one can use Feynman parameters to solve this integral but it might not give a satisfactory answer to this question so we proceed as the book did. Let us shift our analysis to the center of mass system. Here $k'=(0,\mathbf{k'})$ with $\mathbf{k'}=\mathbf{k}_1+\mathbf{k}_2$. The poles will look like,
$$q^0=\pm\left[\sqrt{E_{\mathbf{q}}^2+\mathbf{k'}\cdot\left(\frac{\mathbf{k'}}{4}-\mathbf{q}\right)}-i\epsilon\right],\quad q^0=\pm\left[\sqrt{E_{\mathbf{q}}^2+\mathbf{k'}\cdot\left(\frac{\mathbf{k'}}{4}+\mathbf{q}\right)}-i\epsilon\right]\tag{5}$$
These does not look good but anyways lets move ahead. Taking the poles that are in the lower hemisphere, we have,
$$q^0=\sqrt{E_{\mathbf{q}}^2+\mathbf{k'}\cdot\left(\frac{\mathbf{k'}}{4}-\mathbf{q}\right)},\quad q^0=\sqrt{E_{\mathbf{q}}^2+\mathbf{k'}\cdot\left(\frac{\mathbf{k'}}{4}+\mathbf{q}\right)}\tag{6}$$
Let's check both the poles to see if a branch cut can develop or not. The left pole gives us the integral after picking up the residue,
$$i\delta\mathcal{M}_1=i\frac{\pi}{2}\frac{\lambda^2}{2}\int_m^{\infty}\int_{-1}^{1}\frac{dE_{\mathbf{q}}\,d(\cos\theta)}{(2\pi)^3}\frac{E_{\mathbf{q}}}{|\mathbf{k'}|\cos\theta}\frac{1}{\sqrt{E_{\mathbf{q}}^2+\frac{|\mathbf{k}|'^2}{4}-|\mathbf{k'}||\mathbf{q}|\cos\theta}}\tag{7}$$
We can already see that the integrand does not become imaginary in the given domain of integration so there is no possibility of a branch cut although there definitely is a pole at $\cos\theta=0$, which can be handled by taking a principal value (there is a caveat which we will discuss at the end). Let us proceed, doing the $\cos\theta$ integration we are left with,
$$i\delta\mathcal{M}_1=-i\frac{\pi}{2}\frac{\lambda^2}{2}\int_m^{\infty}\frac{dE_{\mathbf{q}}}{(2\pi)^3}\frac{E_{\mathbf{q}}}{|\mathbf{k'}|}2\,\text{artanh}\left(\sqrt{1-\frac{|\mathbf{k'}||\mathbf{q}|\cos\theta}{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}}\right)\Bigg|_{-1}^1\frac{1}{\sqrt{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}}\tag{8}$$
And similarly for the other pole,
$$i\delta\mathcal{M}_2=i\frac{\pi}{2}\frac{\lambda^2}{2}\int_m^{\infty}\frac{dE_{\mathbf{q}}}{(2\pi)^3}\frac{E_{\mathbf{q}}}{|\mathbf{k'}|}2\,\text{artanh}\left(\sqrt{1+\frac{|\mathbf{k'}||\mathbf{q}|\cos\theta}{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}}\right)\Bigg|_{-1}^1\frac{1}{\sqrt{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}}\tag{8}$$
Both of these look horrendous but we don't need any further analysis to get to the required answer. There will be two terms one corresponding to $\cos\theta=1$ and another $\cos\theta=-1$. Notice that so far everything within the denominator square root is positive so we don't have to worry about poles coming from there. The inverse hyperbolic tangent can be written as,
$$\text{artanh}\,x=\frac{1}{2}\ln\left(\frac{1+x}{1-x}\right)\tag{9}$$
This should give us a hint of some sort of logarithmic divergence. However we expect that the amplitude should be real. Sadly plugging in the value for $x$ for any one pole gives us an imaginary components which is a result of the complex logarithm. To see this lets define the short hand,
$$x_1=\sqrt{1+\frac{|\mathbf{k'}||\mathbf{q}|}{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}},\quad x_2=\sqrt{1-\frac{|\mathbf{k'}||\mathbf{q}|}{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}}\tag{10}$$
Then from each pole with its $\cos\theta=1$ and $\cos\theta=-1$ components we get the term,
$$\ln\left[\frac{1+x_1}{1-x_1}\right]-\ln\left[\frac{1+x_2}{1-x_2}\right]\tag{11}$$
And this is not real because while $x_2<1$ for all possible values of $|\mathbf{k'}|$ and $E_{\mathbf{q}}$ within the integration domain, $x_1>1$ making the logarithm complex giving us an imaginary component of $\pi$.
Feels devastating right? After such a lengthy calculation spending hours verifying steps and we can't even get a real result! But wait wasn't the original integral real? Where is this complex contribution coming from? With this we come to our caveat. Of course Peskin and Schroeder talk about one root at a time, for the complex contribution came only from one root. But in our case there is a pole at $\cos\theta=0$. We swiped it under the rug and forgot about it using some undiscussed principal value. Now its time for a better analysis. We take both the $q^0$ poles together in our evaluation. First lets define another short hand,
$$x_1(\theta)=\sqrt{1+\frac{|\mathbf{k'}||\mathbf{q}|\cos\theta}{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}},\quad x_2(\theta)=\sqrt{1-\frac{|\mathbf{k'}||\mathbf{q}|\cos\theta}{\frac{|\mathbf{k'}|^2}{4}+E_{\mathbf{q}}^2}}\tag{12}$$
Adding the two hyperbolic inverse tangent terms together we get,
$$\begin{align}
[\text{artanh}\,x_2(\theta)-\text{artanh}\,x_1(\theta)]\bigg|_{-1}^{1}&=\,\text{artanh}\left[\frac{x_2(\theta)-x_1(\theta)}{1+x_1(\theta)x_2(\theta)}\right]\bigg|_{-1}^{1}\\
&=\,\text{artanh}\left[\frac{x_2-x_1}{1+x_1x_2}\right]\tag{13}
\end{align}$$
Given that for all configuration in the integration domain we have $x_2<1$ and $x_1>1$ which makes this expression real because the term inside the hyperbolic inverse tangent stays within $[-1,1]$ for all $E_{\mathbf{q}}\in[m,\infty)$. What a relief! So indeed the book was right in the $t$-channel we will not have a branch cut singularity. The calculation for $u$ is the same. Note that this does not imply the amplitude with the current integration domain will be finite. There will be divergences which needs to be regularized but that will fall outside the scope of the question asked.