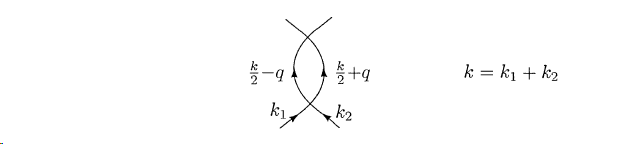
Section 7.3 ("The Optical Theorem") in Peskin and Schroeder's QFT text contains a leading order verification of the optical theorem in $\phi^4$ theory by calculating the (discontinuity across the branch cut in) the imaginary part of the Feynman amplitude $\mathcal{M}$ of the following diagram
$$ i\mathcal{M} = \frac{\lambda^2}{2} \int \frac{d^4q}{(2\pi)^4} \frac{1}{(k/2-q)^2 - m^2 + i\epsilon} \frac{1}{(k/2 + q)^2 - m^2 + i\epsilon} $$
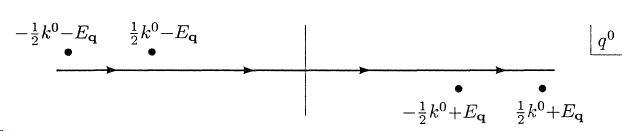
Working in the center-of-mass frame, where $k = (k^0, \vec 0)$, there are two poles below the real $q^0$ line and two above
The textbook here mentions that, if the contour is closed downward,
only the pole at $q^0 = -(1/2)k^0 + E_{\mathbf{q}}$ will contribute to the discontinuity.
I cannot come up with an argument as to why the other pole's residue does not contribute to the discontinuity in $\mathcal{M}$. In fact, from the symmetry between $q$ and $-q$ in the integral above, the two propagators should contribute equally. By factorizing the denominators and calculating residues one can see that indeed the two residues are equal.
What gives? Is P&S wrong here? Or am I making an elementary slip-up somewhere?