Recent interest in what is called "The Hubble Tension" brought to the fore potentially conflicting error estimates for $H_0$ as illustrated in be below diagram. I've been looking for any error estimates for the mass* of the observable universe $m_U$ but thus far I've not found a single such estimate let alone a diagram comparing various error estimates for $m_U$.
Is there such an estimate?
*Presumably it would have to be the sum of all forms of mass-energy, including dark matter. Correct?
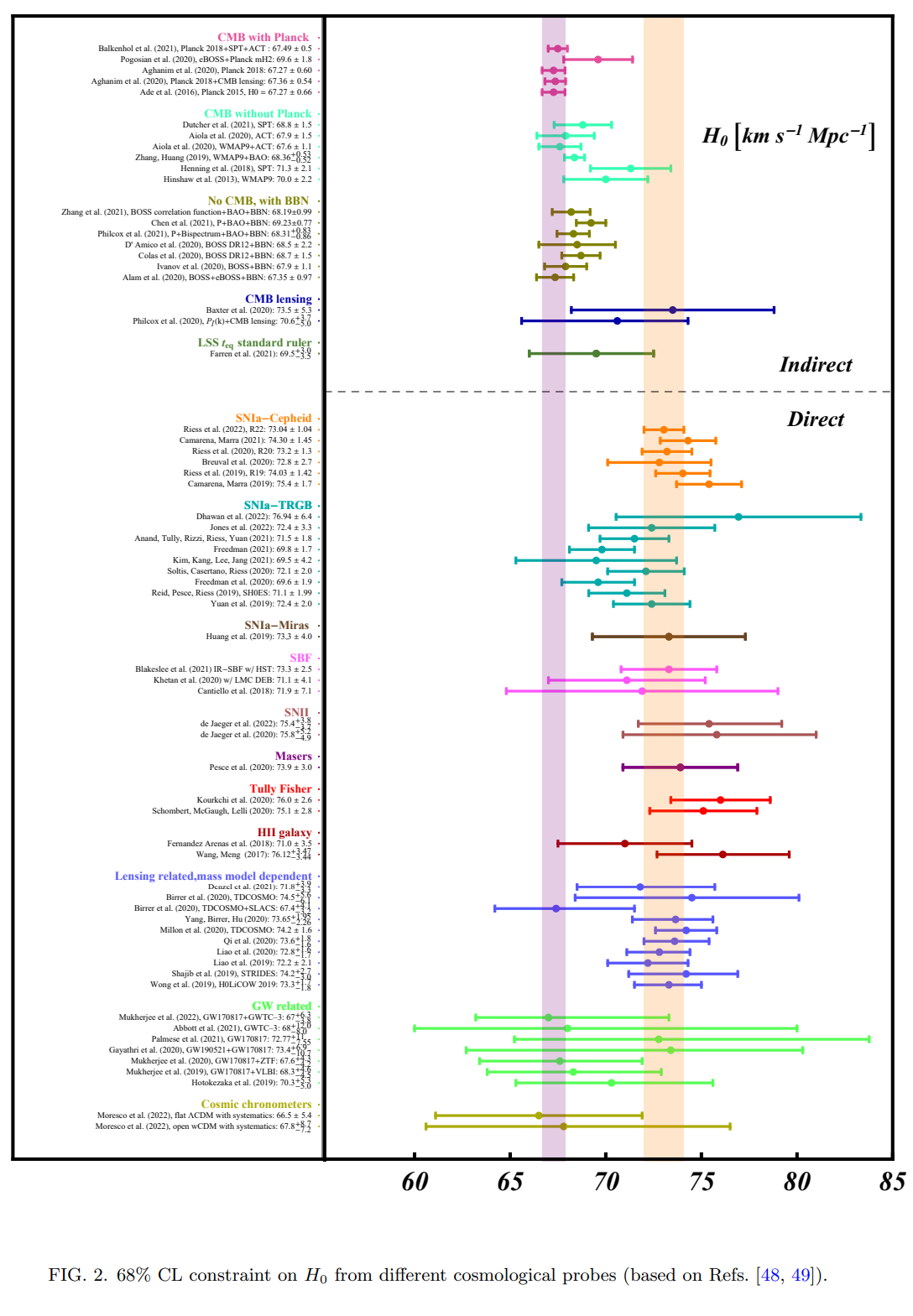
I have not seen it calculated, but I think the fractional error would be similar to the fractional error on the Hubble constant, since it depends on $\sim \Omega_m H_0^{-1}$. The value using the Cosmic Microwave Background (CMB) parameters is $8.82\times 10^{53}$ kg (see below) with a 2% error on $\Omega_m$ and whatever error you think appropriate for $H_0$ based on the picture above.
Details:
The calculated mass would be the current mass density of the universe multiplied by the volume within the particle horizon. This is a quantity that cannot be measured, only inferred indirectly within a model framework for the expansion history of the universe. i.e. It depends on your assumed cosmology and the parameters that describe it. In contrast $H_0$ is something that can be measured (locally).
In particular I don't think you can do a sensible comparison of the indirect (CMB-based) value with "direct" values, because "direct" measurements of the matter density would have very large uncertainties.
Assuming the concordance $\Lambda$CDM model, then the mass density is a multiple of the critical density, which itself is dependent on $H_0^2$. The multiple is known very precisely as $\Omega_m = 0.315\pm 0.007$ (Planck Collaboration 2018) and so the present mass density uncertainty is dominated by uncertainty in $H_0$ (if you assume the scatter of 2-3 km/s/Mpc in the plot in the question to represent a $\sim 5$% uncertainty in $H_0$).
The particle horizon, defining the edge of the (in principle) observable universe is given by $cH_0^{-1}$ multiplied by a complicated integral involving the cosmological parameters $\Omega_m$, $\Omega_\Lambda$, $\Omega_r$ and $\Omega_k$ (e.g. see here). All of these parameters are known to high precision and again, the dominant error would be in $H_0$.
For a very nearly flat universe, the observable volume is proportional to the cube of the particle horizon distance. Thus ultimately, the mass of the observable universe will be proportional to $H_0^{-1}$ and its uncertainty will have a similar fractional uncertainty to the present-day Hubble parameter (though some full Monte Carlo simulation would be needed to deal with the smaller contributions from the other parameters and their covariances).
In terms of a value, one can use the Planck2018 parameters, with $H_0 = 67.5$ km/s/Mpc to calculate a radius for the observable universe of 45.21 billion light years (using $z=1100$ as the limit to the observable universe). This $H_0$ then leads to a mass within the observable universe (dominated by dark matter) of $8.82\times 10^{53}$ kg, with about a 2% error due to the uncertainty in $\Omega_m$. Scale this with $H_0^{-1}$ as desired.
NB Increasing the redshift to infinity for the potentially observable universe (neutrino telescopes?) increases the enclosed mass by 5.3%.
