Schwarzschild singularities are described by the Kantowski-Sachs metric with a contracting S2. Of course, T-duality doesn't apply to S2. But what about a Kasner-type singularity with two contracting spatial dimensions compactified over a torus T2, and an expanding spatial dimension? The T-dual of the torus gives rise to a geometry which is expanding in all spatial directions.
1 Answer
The Kasner solutions were the starting point of the paper that studied whether S,T,U-dualities are enough to de-singularize every possible scaling limit of tori:
Dualities vs singularities
http://motls.blogspot.com/2009/02/dualities-vs-singularities.html
http://arxiv.org/abs/hep-th/9811194
The answer is that if there are at least 2+1 large dimensions, it's always possible to find a duality that makes all the dimensions larger than the fundamental (Planck/string) scale and that makes the coupling smaller than one, in the case of string theory descriptions.
The moduli space of M-theory on tori is completely covered by regions where M-theoretical, type IIA, or type IIB description is weakly coupled in this sense. One gets pretty beautiful tilings of the moduli space in this way - see the blog post with a Mathematica-generated one. The picture refers to the case of 1 large dimension where the moduli space has a Lorentzian-metric and only the future light-cone may be dualized to well-understood vacua.
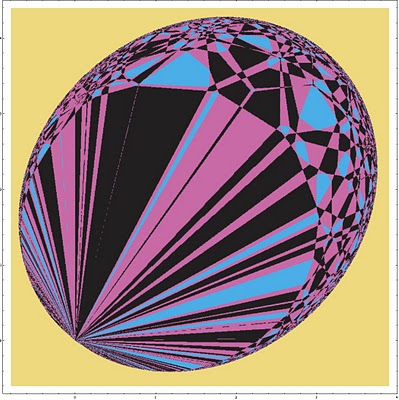
It just happens that I co-authored the paper above which is most relevant for your question but statistics happens. ;-) We also wrote a heterotic followup. The moduli space is more complicated over there and many more descriptions have to be included. There's no "canonical" subspace of the moduli space, analogous to the rectangular tori above, so the work remained incomplete over there.
The dualities were performed in the way that would apply to static configurations in general relativity. The time-dependence itself may change many things. For spacelike singularities, the time dependence becomes huge and essential which is why the fate of spacelike singularities remains poorly understood in string theory - very different from the time-like singularities whose physics has been largely cracked.
If the compact dimensions are spheres - which have no non-contractible loops - the required winding states etc. don't exist and you can't de-singularize the spacetime if it is singular "almost everywhere". However, you may de-singularize spacetimes where spheres etc. shrink locally - that's what mirror symmetry (a fiber-wise T-duality) often does.
-
2$\begingroup$ Excellent, including the part about not being able to use T-duality for circle that are rapidly time varying. $\endgroup$– user566Commented Mar 19, 2011 at 17:04
