5
$\begingroup$

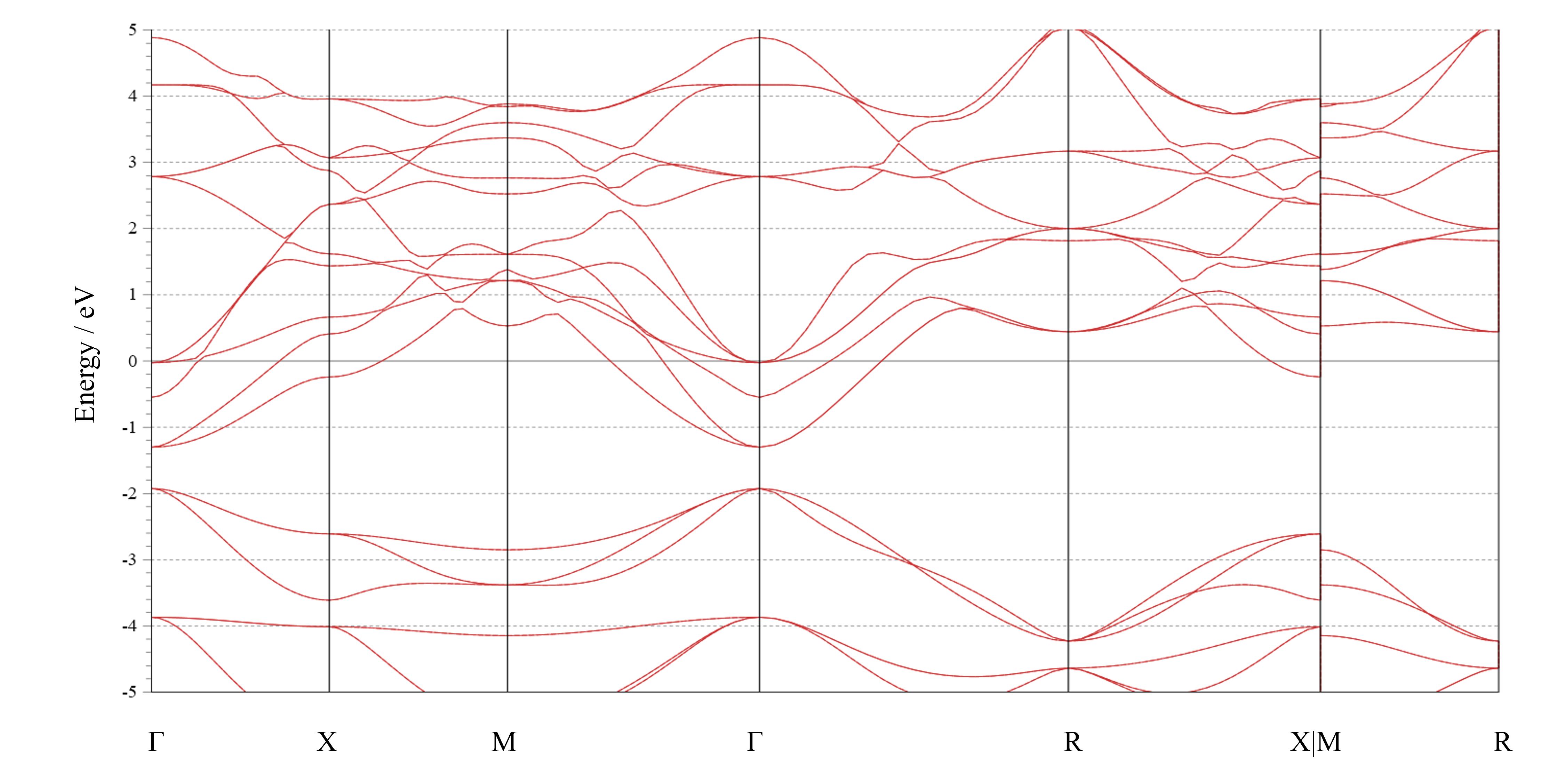
I've been working on this material to get more accustomed to Quantum Espresso, and I've gone on and performed calculations to get their band structures. Here are the band structures that I got for two of the variations of my material: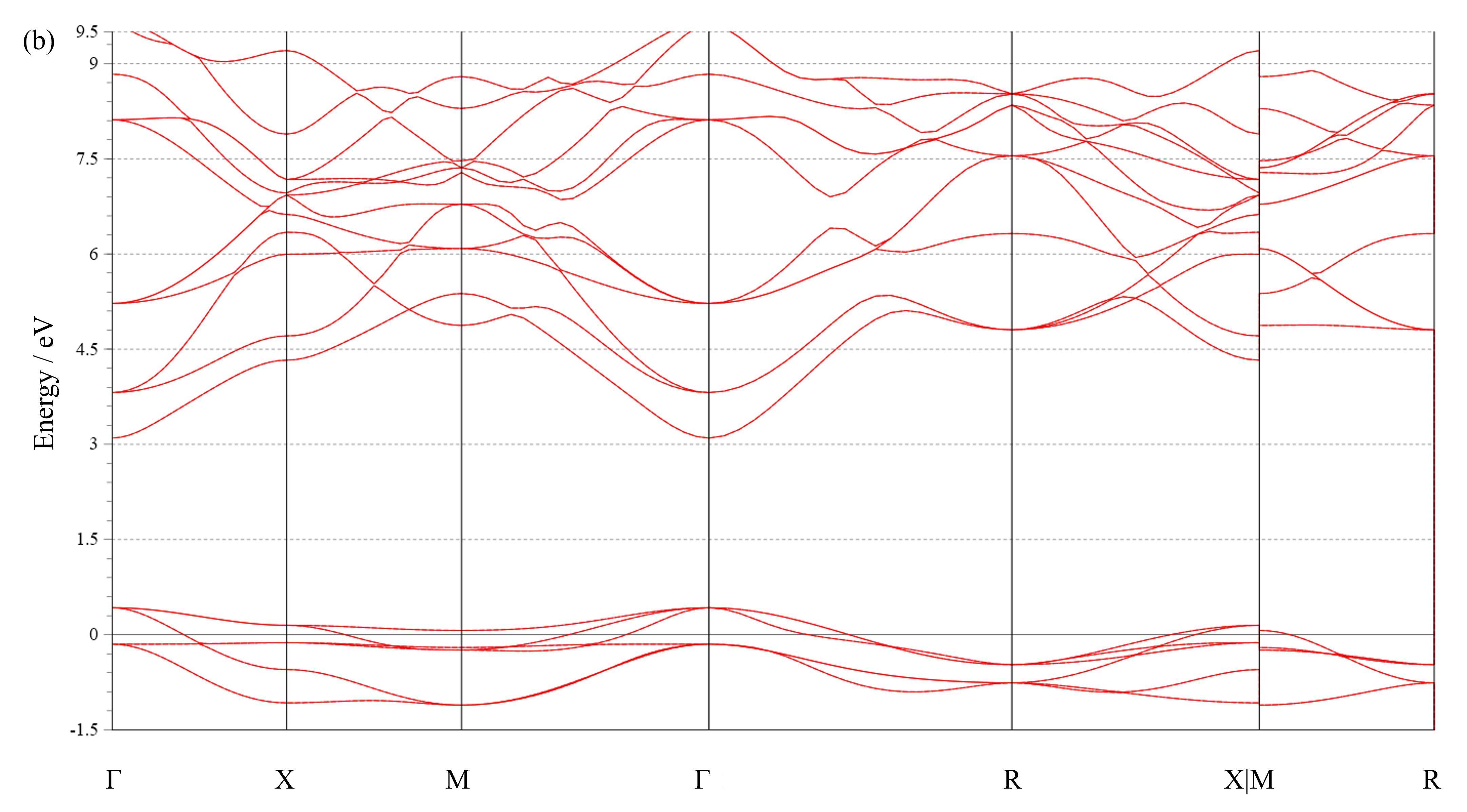Band Structure 1

Band Structure 2

Used EV-GGA to get these band structures, I could have used others, but I'm just curious as to what these particular band structures imply. As you can see, for the first one, the Fermi level has crossed into the valence band while for the second one the Fermi level is in the conduction band. Do these both imply metallic nature? These are undoped materials, can doping possibly cause the Fermi levels to move accordingly and make these materials semiconductors? Thank you everyone for your help.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
7
$\begingroup$

The terms "conduction band" and "valence band" sort of lose their usefulness if you are not talking about a standard band insulator where you have a filled valence band and the chemical potential lies in the gap.

In your two cases, both materials will be metallic because there is finite density of states at the Fermi level.

Yes with doping you can move the Fermi level. Electron doping will move the Fermi level up and hole doping will move it down. But in the actual real(physical) material it may not be possible to dope it sufficiently to put the Fermi level within the gap.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Thank you so much for your answer. The doping thing may be a good avenue for further study because I'm also curious about how the location of the Fermi level changes with doping. I'd like to ask about what you what you said about the finite density of states. Would much denser bands around the Fermi level imply some other characteristic for the material? $\endgroup$
    – tumblewush
    Commented May 17, 2022 at 7:43
  • 1
    $\begingroup$ It depends on what you mean by "denser bands". If you mean more bands, then that will affect what your Fermi surface looks like. For example, In both of your cases multiple bands cross the Fermi level, so you will have fairly complicated Fermi surfaces with multiple hole and electron pockets. This will certainly affect the material properties, such as the conductivity tensor. If you mean tuning the density of states itself, that is related to the curvature of the bands and that will also affect the material in many ways, some simple like it will change the conductivity, $\endgroup$
    – pmal
    Commented May 17, 2022 at 7:54
  • 1
    $\begingroup$ but also potentially in more complex ways because if you have a high density of states then the system will be more susceptible to collective instabilities like magnetism or superconductivity. $\endgroup$
    – pmal
    Commented May 17, 2022 at 7:54
  • $\begingroup$ Thank you so much! You've given me a good place to start with how to rationalize these band structures. Do you have any good references that I could use to read more about this? $\endgroup$
    – tumblewush
    Commented May 17, 2022 at 8:07
  • 1
    $\begingroup$ That is a bit hard to answer without knowing your background knowledge. If you are doing DFT calculations, I would think you have already read some standard solid state physics books? If not, Ashcroft and Mermin is a standard, and also Kittel. My favorite more modern condensed matter book is Girvin and Yang. $\endgroup$
    – pmal
    Commented May 17, 2022 at 8:11
5
$\begingroup$

In the book by Naeman, Semiconductor Physics, the equation $$E_F -E_{Fi}=kT\ln{\frac{n_0}{n_i}}$$ is derived and relates the change in the Fermi level due to doping. $E_F$ is the Fermi level after doping and $E_{Fi}$ is the intrinsic Fermi level, where $n_0$ and $n_i$ are the initial and final electron number densities before and after doping.

So the Fermi level clearly changes due to doping.

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ Thank you for this! I will be looking into Naeman's book as well, thanks for giving me a reference. $\endgroup$
    – tumblewush
    Commented May 17, 2022 at 7:45
  • 1
    $\begingroup$ That's ok. good luck with your studies. $\endgroup$
    – joseph h
    Commented May 17, 2022 at 7:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.