Multi-electron Bloch bundles over powers of the Brillouin torus?

Question

The quantum states of single electrons in a crystal famously form a (Hilbert) vector bundle over the Brillouin torus ${\mathbb{T}}^d$ -- the Bloch bundle.

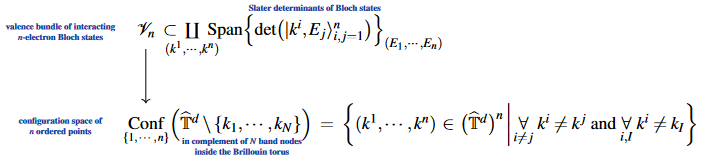
Does this theory usefully generalize to spaces of $N$-electron states for $N \geq 2$, in that these would form, under suitable conditions, a vector bundle over the $N$-fold product $\big({\mathbb{T}}^d\big)^N$ of the Brillouin torus with itself?

Or rather: a vector bundle over the complement of the "fat diagonal" in here, i.e. over the "configuration space of $N$ points" in the Brillouin torus -- something like this:

I see what might be a vague indication in this direction, towards the end of:

• Yuejin Guo, Jean-Marc Langlois, William A. Goddard: Electronic Structure and Valence-Bond Band Structure of Cuprate Superconducting Materials, Science, New Series 239 4842 (1988) 896-899 (jstor:1700316)

where the authors speak of "$N$-electron band theory". The multi-indices of the Slater determinants used in such contexts would be points in that $N$-fold product of the Brillouin torus with itself.

More explicitly, the authors of

• J. Hu, J. Gu, W. Zhang: Bloch’s band structures of a pair of interacting electrons in simple one- and two-dimensional lattices, Physics Letters A 414 (2021) 127634 (doi:10.1016/j.physleta.2021.127634)

work out explicit examples of "2-electron bands" which are functions on the configuration space of 2 points in the Brillouin torus.

But what I am wondering is whether these Slater-determinant states relative to single electron Bloch states usefully organize themselves into a Bloch-like vector bundle over products of the Brillouin torus, as indicated above -- or rather: Whether this is being discussed and developed anywhere in the literature?

Answer 1

When the many-body system ground state $|{\Psi_0}\rangle$ can be expressed as s single Slater determinant it is essentially the the exterior product of non-interacting fermions in a set of full bands
$$ |{\Psi_0}\rangle = \bigwedge_{n, {\bf k}} |\psi_{n,{\bf k}}\rangle. $$ It can then be thought of as a point in a Grassmannian manifold ---i.e. an $N$-dimensional hyperplane in the single-particle Hilbert space. This picture is no longer strictly valid when interactions are turned on as the ground state is no longer a single Slater determinant but unless the interactions alter the topology of the ground state bundle, the Chern numbers can be computed from the Grassmannian. The result is that for an insulator, the many-body Chern number ${\mathcal N}$ is the sum of the Chern numbers computed from the $u_{n,{\bf k}}$ of the occupied bands.