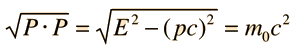Is light's momentum $0$?

Question

From Paul Dirac's extended mass-energy equivalence equation, we know that,

E² = (mc²)² + (pc)²

We also know that E = mc²

So, if we write mc instead of E in the equation and then subtract mc² from the both side, we get 0 in one side and (pc)² on the other.

Like so:

E² = (mc²)² + (pc)²

=> (mc²)² = (mc²)² + (pc)²

=> (mc²)² - (mc²)² = (mc²)² + (pc)² - (mc²)²

=> 0 = (pc)²

Since, p is the momentum, can't we state that light has 0 momentum? Am I missing something? Did I make any mistake in the equation?

Answer 1

If you start with the energy momentum relation $$ E^2 = \left(m_{0} c^2\right)^2 + \left(pc\right)^2 $$ where $m_{0}$ is the rest/invariant mass, and apply it in the rest frame (where $p = 0$), you get $$ E = m_{0}c^2 $$ You've turned the argument around, and asked "what are the conditions where $E = m_{0}c^2$?" You found that $p = 0$.

For a photon, $m_{0} = 0$, so the first equation becomes $$ E = pc \ \ \Rightarrow \ \ p = \frac{E}{c} $$ With $E = hf = hc/\lambda$, this means the momentum of a photon is $$ p = \frac{hf}{c} = \frac{h}{\lambda} $$

Answer 2

Your original equation is only correct when you mean m to be rest mass, which is actually denoted $m_{0}$. m is actually $\gamma m_{0}$ and is called relativistic mass.

You are finding conditions on the equation that make $E=m_{0}c^2$. Which ultimately give $P = 0$. Have you noticed that your reasoning has nothing to do with light? You're just proving that $P=0$ when your condition is met.

Your mistake lies in the fact that you believe $E=m_{0}c^2$ holds in general. It does not. Only when momentum is zero does this hold.

The standard equation:

$$E=mc^2 = \gamma m_{0}c^2$$

Which I believe you were trying to assert is true always, is also not true in general. (instead of the equation with $m_{0}$)

Substituting this correctly, as you were trying to do, yields that when $E=\gamma m_{0} c^2$

$$P = \gamma m_{0} v$$

Which is the equation for momentum of a massive body.

This does not apply for light, since atleast in the context of classical electrodynamics, the field momentum is denoted by $$P = \mu_{0} \epsilon_{0} \vec{S}$$

As pointed above, when $m_{0} = 0$, $E=pc$, which is the equation for the energy of light. ( which can also be independantly derived from maxwells equations)

Answer 3

For me it is simple to think in terms of classical light as the ensemble of photons, quantum mechanical particles obeying the Lorentz transformations.

This means that a photon, which has zero mass, will have a four vector

Zero mass particles do have a momentum, setting $m_0$ to zero and thus getting the momentum of the photon $pc=E$ .

The classical light is built up by a large number of photons whose momenta will add up to the momentum of a beam of light.

This answer of mine gives an intuition of the complex way single photons build up classical light. .