Distribution of Charges on a Conducting Cup

Question

A multiple-choice problem goes as follows:

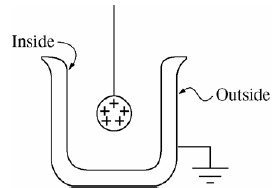
A small positively charged sphere is lowered by a nonconducting thread into a grounded metal cup without touching the inside surface of the cup, as shown above. The grounding wire attached to the outside surface is disconnected and the charged sphere is then removed from the cup. Which of the following best describes the subsequent distribution of excess charge on the surface of the cup?

and the given correct answer is:

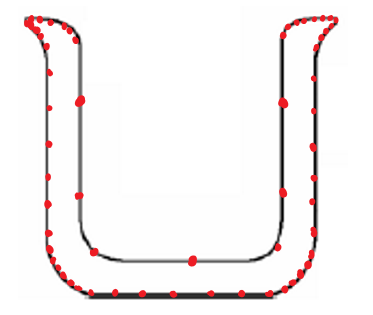
Negative charge resides on the outside surface, and no charge resides on the inside surface.

with an explanation online:

When lowered inside, the charged sphere induces a negative charge on the inner surface of the cup. The outer surface remains neutral since it is grounded. When the grounding wire is removed, the cup has a net negative charge, which when the sphere is removed, will move to the outer surface of the cup.

I agree with this right until the sphere is removed and the charges reconfigure. I can see how a much larger amount of charge would reside on the outer surface, and I can see that the charges on the inner surface will initially be repelled away from the inner surface, but is it true that exactly no charge will be on the inner surface?

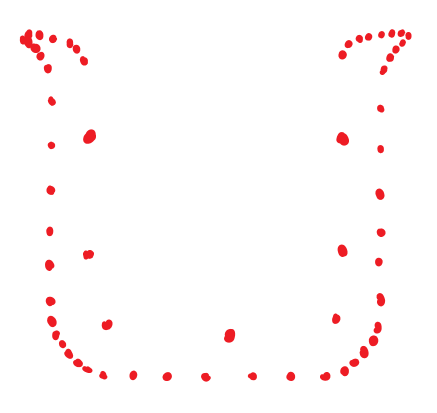
My thought process is that on the inwards bends on the inner surface, it is going to be very hard for charge for to stay there since nearby charges will push them off

So if there is a lot charge on the inner surface, a lot of it will leak off from these inwards bends, but it could still accumulate in the outwards bends on the inner surface, and if there is a small enough amount of charge on the inner surface, the charge on the outer surface could hold the charges at those inwards bends in place, making me think the charge distribution could look something like:

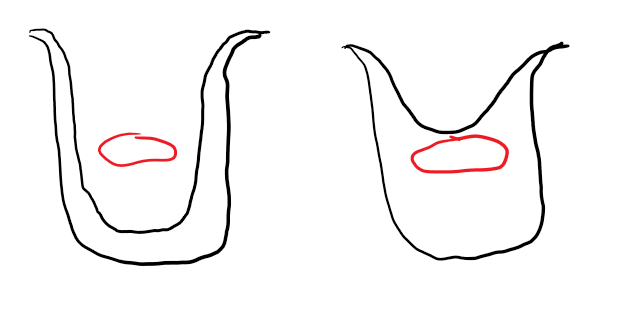
and if no charge resides on the inner surface, wouldn't that imply there is independence between outer and inner surfaces, so we could change the inner surface to not affect the outer surface distribution (as long as its still an "inner" surface):

and get that the red circled region has an E-field of 0 in both pictures since in one its inside the conductor? Not sure I believe that.

So is it true that no charge will reside on the inner surface of this cup?

Answer 1

It is not strictly true that there is no charge on the inner surface. Note that as far as the field/charge is concerned, there is no abrubt transition between the outer surface and the inner surface, so it would be odd if the charge density suddenly dropped to zero at some point on the cup, and naturally it doesn't.

The figure above shows the electrostatic simulation result for such a cup. The arrows indicate the E-field direction and magnitude (arrow length is proportional to the magnitude), and the colors correspond to the logarithm of the E-field magnitude normalized to the peak value: $$ \log_{10}\left(\frac{E}{E_{max}}\right)$$ Note that the surface charge density on the cup is proportional to the E-field magnitude at the surface. You can see that the E-field (and hence the surface charge density) gradually drops as you go downward on the inner surface of the cup. The charge density on the inner surface can be as low as ~4 orders of magnitude less than on the outer surface. So it is understandable that one might consider the charge density to be zero on much of the inner surface for practical purposes, but as you can see, this is not strictly true.