Landau and Lifshitz - collisions between particles

Question

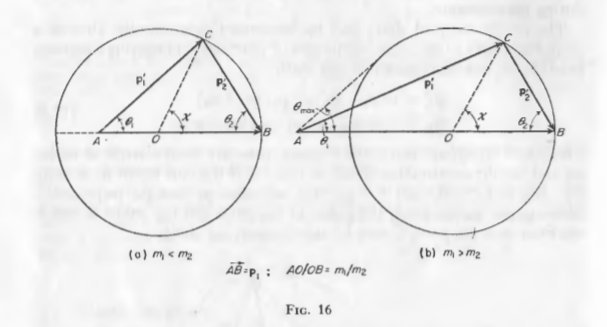
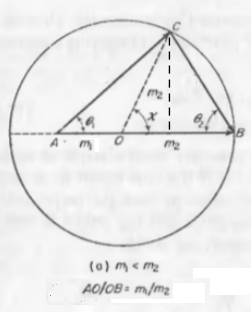
In the book mechanics from Landau & Lifshitz, section 17 collisions between particles there are those two equations in page 46: $$\tan \theta_1 = \frac{m_2 \sin \chi}{m_1+m_2\cos\chi}, \quad \quad \theta_2 = \frac{1}{2}(\pi-\chi).$$ How did they derive these equations?

The vectors in the image are: $$\textbf{p}_1' = m \mathcal{v} \textbf{n}_0 + m_1\frac{\textbf{p}_1+\textbf{p}_2}{m_1+m_2}$$ $$ \textbf{p}_2' = -m \mathcal{v} \textbf{n}_0 + m_2 \frac{\textbf{p}_1+\textbf{p}_2}{m_1+m_2},$$ and from these we get $$\vec{AO} = \frac{m_1}{m_1+m_2} (\textbf{p}_{1} + \textbf{p}_{2})$$ $$\vec{OB} = \frac{m_2}{m_1+m_2} (\textbf{p}_{1} + \textbf{p}_{2})$$ $$\vec{OC} = m \mathcal{v},$$ given that $m$ is the reduced mass $$m = \frac{m_1 m_2}{m_1+m_2}.$$

A link to the book:

https://cimec.org.ar/foswiki/pub/Main/Cimec/MecanicaRacional/84178116-Vol-1-Landau-Lifshitz-Mechanics-3Rd-Edition-197P.pdf

Answer 1

You got so close to an answer.

The authors are considering a situation when $|\vec p_1|= m_1v$ and $|\vec p_2|= 0$

This means that $|\vec{AO}| = \dfrac{m_1^2\,v}{m_1+m_2}$ and $|\vec{OB}| = \dfrac{m_2\,m_1\,v}{m_1+m_2} =|\vec OC|$

Taking the common factor, $\dfrac{m_1\,v}{m_1+m_2}$ out of each of the lengths results in the following diagram.

Noting that $OB=OC$ for the second relationship the required equation,

$\tan \theta_1 = \dfrac{m_2 \sin \chi}{m_1+m_2\cos\chi}$ and $\theta_2 = \dfrac{1}{2}(\pi-\chi),$

then follow.

---

Although they do not answer your question you might find these two articles of interest?

Diagrammatic Approach for Investigating Two Dimensional Elastic Collisions in Momentum Space I: Newtonian Mechanics

Diagrammatic Approach for Investigating Two Dimensional Elastic Collisions in Momentum Space II: Special Relativity