Integral for the sum of $y$-component of an infinite number of forces

Question

I'm new to integrals, so I'm kind of trying to understand how it works

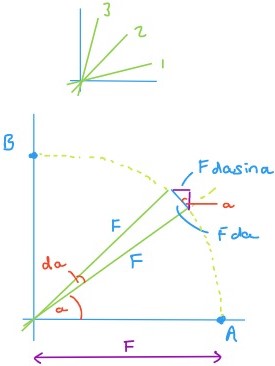
Let's say you are in the first quadrant and you have a infinite number of forces with intensity $F$ which create with the positive $x$ axis an angle $0 \leq a \leq \pi/2$

Now if I want to sum the $y$-components of this forces I should use something like

$$\int_0^{\pi / 2} F \sin(a) da$$

Which gives me F, but it doesn't make sense: if you consider $a = \pi/2$ alone, the y-component is F, so the whole sum must be greater than that...

I know it may sound stupid but I've just started studying this!

Answer 1

If you want to do a sum of all the $y$ components it's

$$\sum_{i=1}^nF_i\sin (a_i)\tag1$$

first diagram, but that'll be infinite for an infinite number of forces.

It's not really possible to replace the sum by an integral for an infinite number of forces, in the way you tried because the $da$ at the end of the integral represents a small change of angle.

So what your integral does is integrate, or sum, the lengths of the infinite number of $F\sin (a) da$ changes in the $x$ direction, (the top horizontal purple line of the second diagram).

As the angle swings from $0$ to $\pi/2$ the changes add up to the purple line by the $x$ axis, of length $F$.