0
$\begingroup$

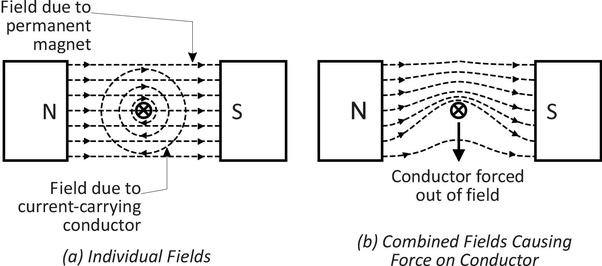
According to the most depictions of a Lorentz force I've found, Outer magnetic field of a wire reinforces the external magnetic field on the other side, and weakens it on the other, so the conductor gets pushed towards the weaker magnetic field (figure below).

Lorentz force

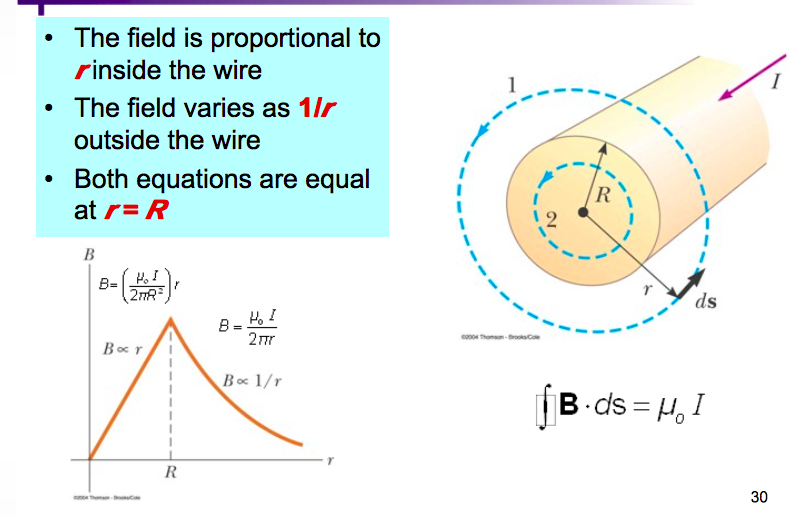
As the magnetic field also exists inside of a wire (as per figure below), does it also gets affected by the external magnetic field?

Magnetic field inside & outside of the wire radius

If so, I can't really comprehend why does a permanent magnet in a form of an axially-magnetized rod (with magnetic field direction going circle-wise, with no distinct north or south pole, just like inside of a wire) doesn't follow the same effect and experiences no force when put into an external magnetic field (what will obviously violate the Energy Conservation law, if an external magnetic field comes from a permanent magnet, for example)

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

(1) Diagram (b) (the 'catapult picture') is a possible way of remembering the direction of the force on a current-carrying wire, but I'm sceptical about its adding to our understanding. For all purposes I can think of, it's simpler to calculate the magnitude and direction of the force on a wire of directed length $\vec l$, carrying current $I$ using $$\vec F=I\ \vec l \times \vec B.$$ $\vec B$ is the external field. It is not some sort of internal/external resultant field.

(2) "As the magnetic field also exists inside of a wire (as per figure below), does it also gets affected by the external magnetic field?" You can, if you wish, calculate a resultant field, but that's not what you need for calculating the force on the wire. See (1) above.

(3) If you want to break the wire down into parallel strands, then the field due to other strands becomes part of the external field for any particular strand. But it turns out that if we sum the forces on all the strands due to the fields from the other strands, the resultant 'self-force' on the wire is zero! Only the field from sources outside the wire gives rise to a force on the wire.

(4) "permanent magnet in a form of an axially-magnetized rod" This would be a bar magnet.

(5) "(with magnetic field direction going circle-wise, with no distinct north or south pole, just like inside of a wire)" But this not the field of a bar magnet!

(6) "doesn't follow the same effect and experiences no force when put into an external magnetic field " I'm lost. The same effect as what?

Improve this answer
$\endgroup$
3
  • $\begingroup$ By the "axially magnetized rod" I didn't really mean the bar magnet, what I'm trying to visualize is a cylinder, where field lines are circular (bottom image in the linked figure), for example, it can be created by combining two half-cylinders with longitudinal poles (like on a top image). Theoretically, it can be residual magnetism in a steel rod, created by passing a current through it. So on a high level it's internal magnetism resembles such of a current-carrying wire $\endgroup$
    – eyeballpaul
    Commented Nov 28, 2021 at 22:40
  • $\begingroup$ 6 - I am trying to compare the effect of external magnetic field on the described permanent magnet (please see in the comment above) and a current carrying wire. Their internal magnetic fields are similar, but wire also has an external field. (sorry if confusing) $\endgroup$
    – eyeballpaul
    Commented Nov 28, 2021 at 22:47
  • 1
    $\begingroup$ Thank you. You've now explained nicely how the rod is magnetised. I don't know how it will respond to an external field. My guess is that it will experience no force, since, like a toroidal coil, it will have no field outside it and therefore won't exert forces on magnets, hence from Newton's third law, won't itself experience a force. But I'm not confident of this. $\endgroup$
    – Philip Wood
    Commented Nov 29, 2021 at 0:04

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.