How small would a neutron star be to see the entirety of it?

Question

How small in Schwarzschild radii would a neutron star need to be for its gravity to be strong enough to deflect light emitted from one side toward an observer on the opposite side? I know the figure is above $1.5R_s$.

Answer 1

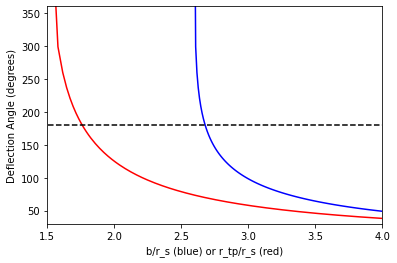
For a Schwarzschild spacetime outside the neutron star (i.e. spherically symmetric and non-rotating), the neutron star surface would need to be at a radial coordinate $\leq 1.76 r_s$ (e.g. Pechenik et al. 1983), where $r_s$ is the Schwarzschild radius. This corresponds to an apparent radius at infinity of $\leq 2.68r_s$.

The derivation (and it is a numerical problem) is to just figure out the closest approach to a Schwarzschild object at which light from infinity is bent through 90 degrees. This means that light emitted tangential to the surface from this minimum radial coordinate at the back of the neutron star will bend through 90 degrees and reach an observer at infinity on the opposite side.

There are possible equations of state being considered for neutron stars that would allow them to be as small as this for large neutron star masses. This effect - that you can see more than $2\pi r^2$ of a neutron star surface - must be taken account of when interpreting the flux of radiation from a neutron star.

EDIT: I attach a plot from a script I've written to calculate the total deflection angle either as a function of the impact parameter of light ($b$) or the closest approach ($r_{tp}$). The plot shows that a total deflection angle of 180 degrees (corresponding to a 90 degree bend for light emitted tangentially from the centre of the opposite side of the neutron star) occurs for $r_{tp}=1.76r_s$ and for an impact parameter of $2.68r_s$. In other words, the apparent radius of the neutron star at infinity would be $2.68r_s$, with the centre of the rear-side of the neutron star forming the outermost ring of the apparent image.