7
$\begingroup$

The Universe's densest objects are black holes. In the second place, there are neutron stars.

So, if a neutron star compresses to its Schwarzschild radius, would it appear as a black hole? That black hole would be one of the most dense objects in the universe?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
11
$\begingroup$

Actually, before you got to the Schwarzschild radius, the pressure previously supporting the neutron star against its own gravity would no longer be able to do so, and the entire star would violently collapse. Some matter would be thrown out, while the rest would become a black hole. The singularity at the center would be among all the rest of the singularities at the centers of all other black holes for the densest objects in the Universe.

Improve this answer
$\endgroup$
8
  • 2
    $\begingroup$ Consider a "stable" neutron star. Are there any mechanisms that allow it to slowly compress further, until it's enough to implode into a singularity? Would mere cooling do that? $\endgroup$
    – Florin Andrei
    Commented Mar 22, 2012 at 16:36
  • 3
    $\begingroup$ @FlorinAndrei Neutron stars are supported by degenerate neutron pressure. Converting neutrons into strange hadrons would reduce the neutron density, but if that mechanism is energetically favorable you get a soft equation of state and consequently a low maximum mass which increasingly looks ruled out by the data. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 22, 2012 at 18:58
  • 1
    $\begingroup$ So, that seems to indicate that, if they don't collapse into a black hole at first, chances are won't collapse later on, is that right? $\endgroup$
    – Florin Andrei
    Commented Mar 22, 2012 at 19:56
  • 5
    $\begingroup$ Unless there is an external source of mass...as is the case in some binary systems that include neutron stars. Mass loss from the companion accumulates on the neutron star until BOOM you get a type uhm...one? supernova. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 22, 2012 at 20:37
  • 1
    $\begingroup$ is it a coincidence that degeneracy pressure of neutron stars is enough to keep it outside the Schwarzschild radius? $\endgroup$
    – Jus12
    Commented Apr 5, 2012 at 14:09
10
$\begingroup$

An important point to make is that it is not possible for a neutron star to shrink "gradually" so that it disappears quietly inside its own event horizon. There will always be some sort of violent collapse because a neutron star becomes unstable at radii significantly larger than the Schwarzschild radius.

A neutron star which gains mass could shrink. This is because the equation of state is temperature independent and may have a density dependence such that the mass-radius relation results in more massive neutron stars being smaller. (This is definitely true for ideal neutron degeneracy pressure, but the equation of state in a neutron star is far more complicated than that - a neutron star supported only by neutron degeneracy pressure could never exceed $0.7M_{\odot}$, the original TOV limit!)

However, there are limits imposed by causality and General Relativity on the structure of neutron stars. In "Black Holes, White Dwarfs and Neutron Stars" by Shapiro & Teukolsky, (pp.260-261), it is shown, approximately, that even if the equation of state hardens to the point where the speed of sound equals the speed of light, that $(GM/Rc^2)<0.405$.

The Schwarzschild radius is $R_s=2GM/c^2$ and therefore $R > 1.23 R_s$ for stability. This limit is reached for a neutron star with $M \simeq 3.5 M_{\odot}$. A more accurate treatment in Lattimer (2013) suggests that a maximally compact neutron star has $R\geq 1.41R_s$.

If the equation of state is softer, then collapse will occur at smaller masses, and higher densities but at a similar multiple of $R_s$.

Thus there will always be some sort of violent collapse event associated with accretion onto a neutron star that then exceeds the TOV limit.

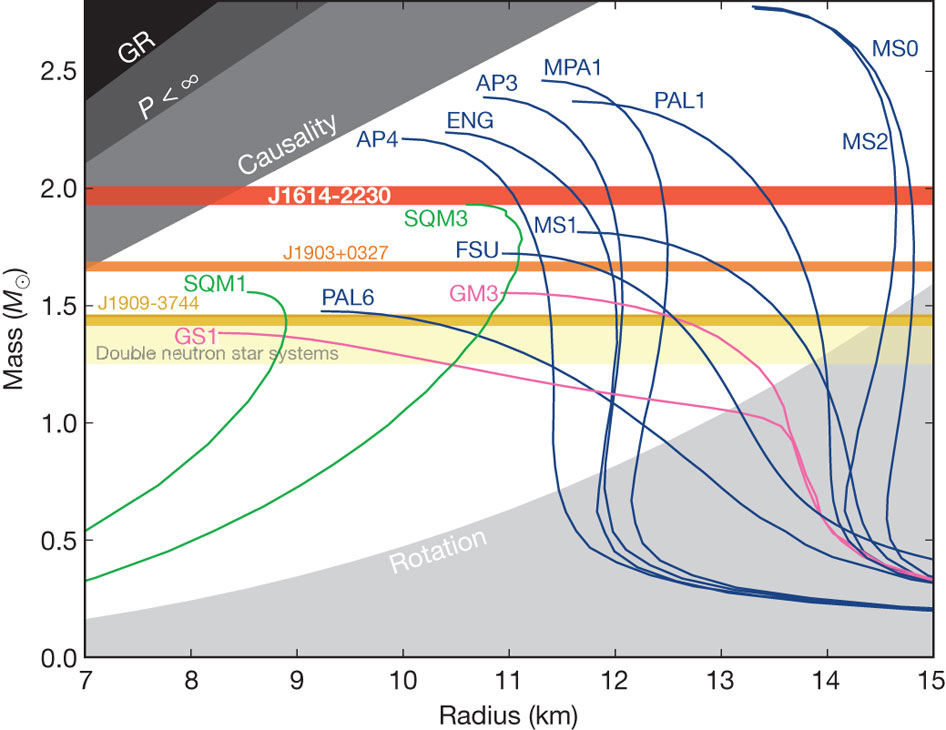
The picture below (from Demorest et al. 2010) shows the mass-radius relations for a wide variety of equations of state. The limits in the top-left of the diagram indicate the limits imposed by (most stringently) the speed of sound being the speed of light (labelled "causality" and which gives radii slightly larger than Shapiro & Teukolsky's approximate result) and then in the very top left, the border marked by "GR" coincides with the Schwarzschild radius. Real neutron stars become unstable where their mass-radius curves peak, so their radii are always significantly greater than $R_s$ at all masses.

Neutron star mass-radius relations

Improve this answer
$\endgroup$
2
  • $\begingroup$ This is Really interesting! Thanks for adding this $\endgroup$
    – DilithiumMatrix
    Commented Jun 2, 2015 at 15:41
  • $\begingroup$ Really nice answer. $\endgroup$
    – Árpád Szendrei
    Commented Apr 20, 2022 at 20:16
5
$\begingroup$

Just to add a little (especially to @dmckee's comment to @Florin_Andrei's response):

A typical, isolated neutron star stably resists gravity with neutron degeneracy pressure. If instead it is accreting mass from a binary companion, it may grow beyond the Tolman-Oppenheimer-Volkoff Limit (just like the Schwarzschild limit for white-dwarfs, except for neutron stars), at which point it will inevitably succumb to gravity and collapse.

Neutron-star collapse is believed to almost always form a black-hole remnant; the exact details are unknown, but there are numerous quite successful models. What exactly it would look like is unclear, but the emission would be much less than the supernova of a typical massive star, or white dwarf. The observational signature would be entirely unlike a type Ia, and most likely unlike a type II as-well.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Type II supernovae are from the collapse of the cores of massive stars, and have to work their way out from the heavy blanket of material above the core. So yeah, a neutron star collapse will look entirely different than that. $\endgroup$
    – Andrew
    Commented Apr 6, 2012 at 11:04
  • 1
    $\begingroup$ The TOV limit if "A typical, isolated neutron star stably resists gravity with neutron degeneracy pressure" would be 0.7 solar masses. The strong nuclear force is required to harden the ideal neutron degeneracy pressure. $\endgroup$
    – ProfRob
    Commented Nov 25, 2014 at 14:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.