I think your understanding is pretty sound.
Can you say that a single mode will be excited containing all wavelengths from 1.5 to 3 μm?
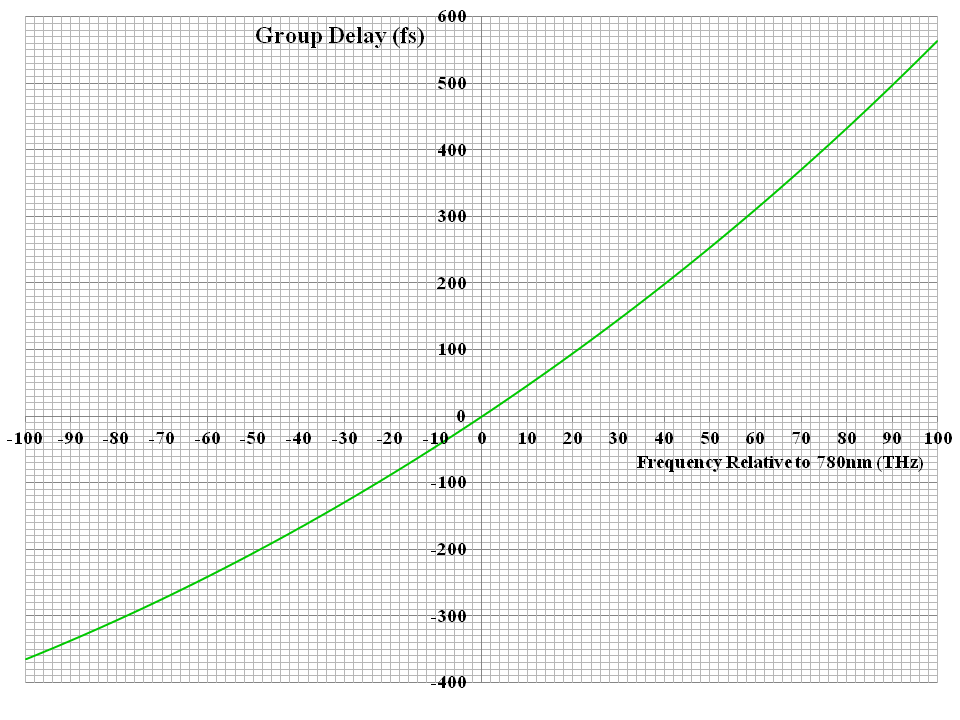
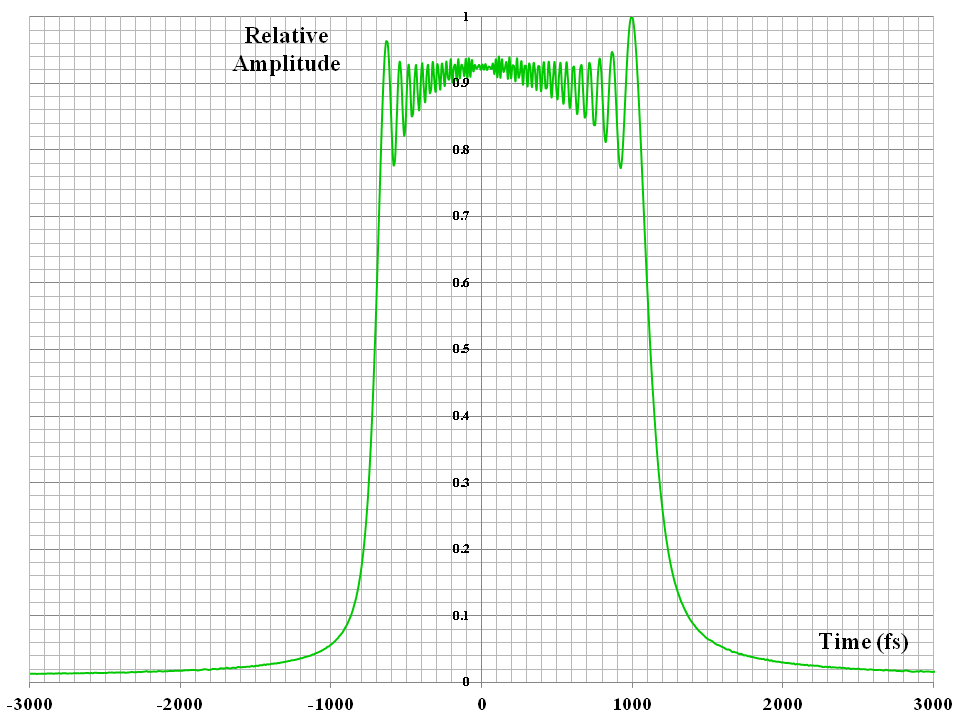
Yes, absolutely. For analysis, you would decompose the light signal by Fourier decomposition (Fourier integral). You would then impart the phase delay through the fibre appropriate to the lone mode at each different wavelength, then rebuild the signal at the other end. To do this precisely, you would need to find out the dispersion relationship for the particular refractive index profile in question. These relationships are derived and tabulated for step index and parabolic profiles in e.g. Chapter 12 of Snyder and Love, "Optical Waveguide Theory". Practically, however, the manufacturers give out group velocities as well as dispersion co-efficients, the latter typically specified in ${\rm ps\,nm^{-1}\,km^{-1}}$ and you would use these to calculate the dispersion. This gives the fibre a distinctive looking impulse response. I plot one below: this is for a length of fibre working in a different band, but it is the impulse response for the group delay with frequency dependence curve shown, and the shape of the response is very typical. It spreads over longer times for longer lengths of fibre, and the ripples in the "on-period" stay roughly the same frequency. So you end up with long pulses looking rather like a flattop haircut in profile as the typical behaviour.
If the source is incoherent then you would need to work out its power spectral density. Ideally, since the fibre only imparts phase delay over the band of interest, this means that the input and output power spectral densities are the same.
And what about if the spectrum extends from 1 to 3 μm - will there be multiple modes excited, since 1.4 μm is the cut-off for single mode behavior? Or is this picture invalid since the light is incoherent?
Here you Fourier decompose the signal as above, and for the part of the signal between 1.4μm and 3μm you would do exactly as above. However, for the signal between 1μm and 1.4μm things are a little tricky. You have here two modes propagating at once, and the amplitude of the field propagating in each one depends on:
The excitation conditions at the fibre's input. If, for example, the input laser is focussed precisely onto the fibre's centre and the fibre is axisymmetric, the first nonfundamental mode has odd-symmetry about any axis (i.e. $\psi(-\vec{r})=-\psi(\vec{r})$ in any transverse plane) and the first nonfundamental mode is not excited. In this case, you would analyse the problem exactly as though the fibre were one moded all the way down to 1μm, using the dispersion relations for the fundamental mode in the 1μm to 3μm region. If both modes are excited in the 1μm to 1.4μm region, you analyse their propagation as in the single mode case separately and then work out their summed effect on the detector at the output. Snyder and Love treat the analysis of excitation conditions thoroughly.
Modes couple into one another owing to fibre bending, inclusions in the fibre, variable stress-optic effect or any other deviation from translational invariance of the fibre's profile. For the fundamental and first non fundamental mode, the difference between the phase velocities tends to be large, so that the two are highly nonresonant and therefore only couple very weakly in most cases. Snyder and Love treats this topic of coupling thoroughly in about Chapter 18.


