0
$\begingroup$

I have a question regarding how the the stationary states (eigenstates) are arrived at in Schrodinger's wavefunction, please.

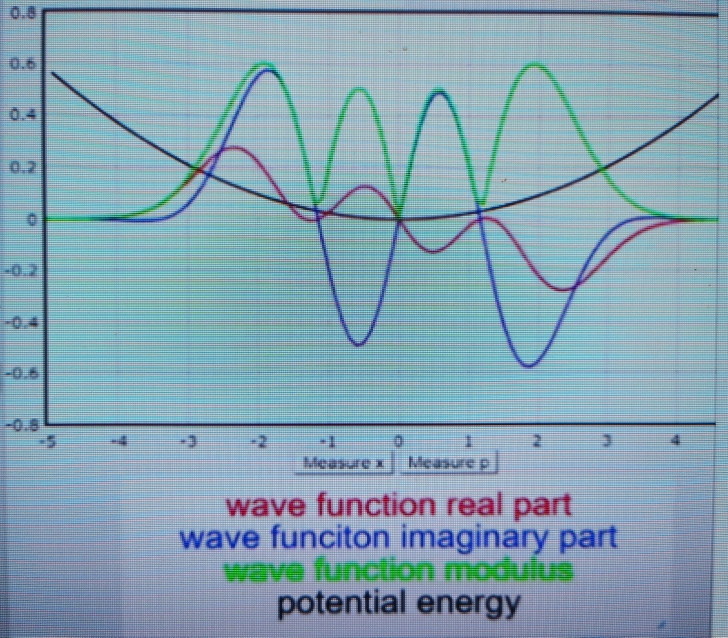
In the graph below, taken from https://www.youtube.com/watch?v=2V0Xmc0ow80&list=PLdCdV2GBGyXM0j66zrpDy2aMXr6cgrBJA&index=4:

we see the resulting position wave shape distribution over a long time period using the potential constraint V(x) = x^2 and the initial wave shape W0(x) = [(2x^3-3x)*exp(-x^2/2)/sqrt(1.25323), 0]. The array indexing refers to [Re_part, Im_part].

The resulting probability function shape (in green) given this initial probability distribution, which we deliberately chose to be W0(x), remains the same over time regardless how the first R part and the second I part is intra-changing individually.

This is referred to as the stationary (or eigen) state, if we only consider the "real" physical green function above (which is the shape of the probability distribution of finding the particle at location x), and ignore the red and blue internal components which are not individually discernable by us.

My question is, this stable state is achieved by first inputting the initial probability shape of W0(x) = [(2x^3-3x)*Math.exp(-x^2/2)/Math.sqrt(1.25323), 0], and letting the shape evolve over time.

If we start with a random "incorrect" initial shape for W0(X) to start with, the resulting evolution over time will probably not automatically evolve into the steady/stationary state by itself.

So, by what process, supposing we just throw an electron into this V(x)=x^2 constraint, for which the electron will probably not find itself in this magical Hermite state to begin with, by what process will the particle discover and settle over time into the stationary (eigen) state like the green wave distribution above?

If the Schrodinger equation claims complete description over the time evolution of the probability wave, then shouldn't the starting condition makes no difference at all, and the particle will always end up in a stationary ("stable") state no matter what the starting W0(x) looks like, just by following the rules of the Schrodinger wavefunction?

Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ There is no reason for the electron to eventually settle into a stationary state. Indeed, the Schrodinger equations predicts that if you don't start in an eigenstate, you will never be an eigenstate; your probability density will be a function of time. $\endgroup$
    – Jahan Claes
    Commented Oct 12, 2021 at 17:58
  • 1
    $\begingroup$ @ Jahan Thank you for answering. I am new to this and still working things out... So, if we throw an electron near a proton, and the Schrodinger function is not "self-stabilizing", how can it be that there are so many hydrogen atoms in existence? Shouldn't nearly all attempts to "entrap" an electron around a proton fail because the electron initial state doesn't lead to a stationary state around the proton? Thanks $\endgroup$
    – user315366
    Commented Oct 12, 2021 at 18:03
  • 3
    $\begingroup$ Isolated few body quantum systems cannot relax into a lower energy state. Rather energy is conserved. However, like in classical systems, by exchanging energy with an environment the system can relax, and when the temperature of the environment is low compared to the energy scales of the system, this relaxation is statistically favoured. How this behaviour emerges in a quantum mechanical system is central question of quantum statistical mechanics. $\endgroup$
    – ComptonScattering
    Commented Oct 12, 2021 at 18:36

1 Answer 1

Reset to default
1
$\begingroup$

How electrons come to be in the lowest energy level of a Hydrogen atom is a very good question. Whether they start off in one definite higher energy level or a superposition of two or more. The reason is that your standard $s, p, d, f$ orbitals are stationary states of the system including just the electron and proton. Not of the system that includes the electromagnetic field as well.

The Wikipedia article on the Jaynes-Cummings model gives a good description of what happens to the contributions from excited states. Initially they decay and then it talks about a "revival time". But this time is on the order of $\omega^{-1}$ where $\omega$ is the minimal frequency that the electromagnetic field can have because it is in a cavity. Atoms in the continuum have $\omega \to 0$ so that the process looks like pure decay.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.