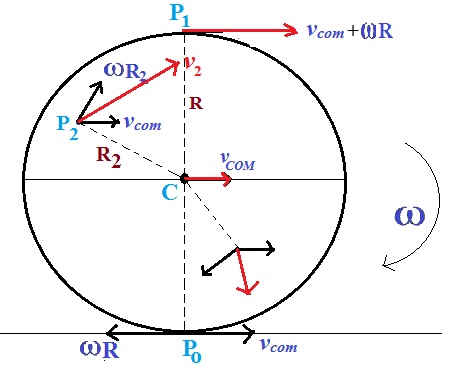
Why is the resultant velocity of a particle inside a body undergoing rolling without slipping always perpendicular to the line segment connecting it and the instantaneous axis of rotation?
$P_2V_2$ is the resultant velocity.
$P_2V_2=\omega R^2+V_{CM}$
$P_0$ is the instantaneous axis of rotation.
Rephrased question: why is $P_0P_2$ always perpendicular to $P_2V_2$ for all $P_2$ situated inside the circle?