0
$\begingroup$

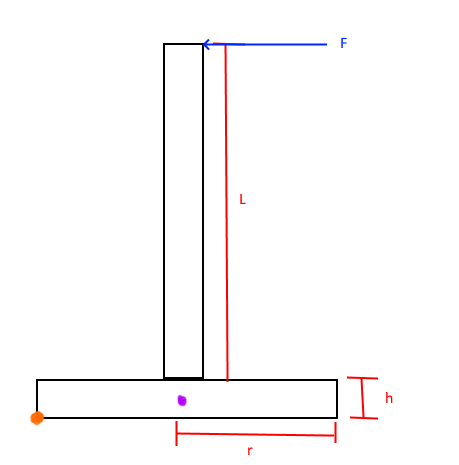
enter image description here

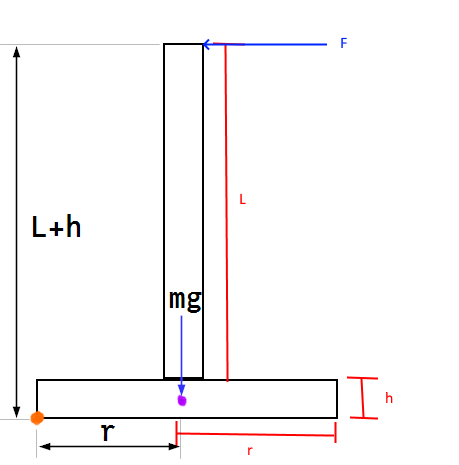
I want to calculate the minimum force $F$ required to tip the above shape about the pivot point (orange dot). Assume the mass of the vertical portion is negligible compared to the base. Assume that the center of mass is where the purple dot is.

I know the torque of due to the center of mass is $\tau_{\text{com}} = m g r$, but I'm having trouble conceptualizing the torque due to $F$, since it is off-axis. My guess is that it's either $\tau_F = F L$, or $\tau_F = F \sqrt{(L+h)^2 + r^2} \cdot \sin(180-\tan^{-1}(\frac{L+h}{r})$.

Is it one of those, or am I completely wrong?

Improve this question
$\endgroup$
7
  • $\begingroup$ Seems odd that you are drawing the moment arm for the weight to the right of the center of mass, instead of to the left. Also, what do you mean by $F$ being "off axis"? $\endgroup$
    – Bob D
    Commented Oct 1, 2021 at 18:28
  • $\begingroup$ Look at the diagram. I'm applying a force that's not along the axis of rotation about the orange point. $\endgroup$
    – explodingfilms101
    Commented Oct 1, 2021 at 18:39
  • $\begingroup$ Note that $$\sin\left[180^\circ - \tan^{-1} \left( \frac{ L+h}{r} \right) \right] = \sin\left[\tan^{-1} \left( \frac{ L+h}{r} \right) \right] = \frac{(L+h)/r}{\sqrt{ 1 + (L+h)^2/r^2}} = \frac{L+h}{\sqrt{ r^2 + (L+h)^2}}$$where I've used one of the identities in this table in the second step. $\endgroup$
    – Michael Seifert
    Commented Oct 1, 2021 at 18:48
  • $\begingroup$ @explodingfilms101 Don't you realize that the moment arm of F is the perpendicular distance between the line of action of F and the orange dot, i.e. L+h? $\endgroup$
    – Bob D
    Commented Oct 1, 2021 at 18:55
  • $\begingroup$ So the torque due to $F$ is $F \cdot (L + h)$? @BobD $\endgroup$
    – explodingfilms101
    Commented Oct 1, 2021 at 19:07

2 Answers 2

Reset to default
0
$\begingroup$

The torque due to a force equals the force magnitude times the perpendicular distance to the force axis.

In this case

$$ \tau_F = F (L + h) $$

Also, the torque due to the weight is

$$ \tau_W = m g r $$

equating the two will give you the balance point unless the application of the force moves the center of mass due to deflection.

pic

Improve this answer
$\endgroup$
0
$\begingroup$

Torque can be calculated by multiplying the force by the perpendicular distance from the axis to the line of motion of the force. Your first expression should be: $$F(L + h)$$ I'm thinking the second expression does not require the $180^\circ$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ My first expression is $\tau_{com}$, or $\tau_{F}$? $\endgroup$
    – explodingfilms101
    Commented Oct 1, 2021 at 18:39
  • 1
    $\begingroup$ @R.W. Bird You seem focused on the purple dot. I direct your attention to the orange dot (lower left corner). That's the pivot point. $\endgroup$
    – candied_orange
    Commented Oct 1, 2021 at 18:46
  • $\begingroup$ That's why I suggested (L + h). $\endgroup$
    – R.W. Bird
    Commented Oct 2, 2021 at 14:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.