A lattice of synchronized clocks

Question

Over the years I have seen this image which always confused me:

(from Wikipedia Spacetime)

"In special relativity, the observer measures events against an infinite latticework of synchronized clocks." This sounds needlessly artificial and abstract.

Let me take a stab at what they are trying to say. Say the event is a star exploding which is 1 billion light years away. The light reaches my eyeballs 1 billion years after the event happened. Therefore, one of these synchronized clocks mis-times the event. Do these clocks account for the time taken for the light from events to reach our eyeballs?

In the exact same frame, I could have an observer 1 billion light years away right next to the star when it exploded registering a "more correct" time.

So the observers disagree on the timing of the events, even though they are in the same frame? Or does the far-away observer back-calculate the time of the event, knowing the distance to the star, and then they agree?

Now that I think about it, are all these clocks actually out of synch, but appear to be in synch by the time the light from all of them has reached the eyeballs of our observer?

Answer 1

All it means is that in your stationary frame you have a plane of simultaneity, so if it is 2:37pm where you are it is 2:37pm everywhere in your frame of reference.

For example, if it is 2:37pm where you are 'now' it is also 2:37pm 'now' on Jupiter, which is about 33 light minutes away. If a light from Jupiter arrives at you now at 2:37, then in your frame of reference it must have left Jupiter 33 minutes ago at 2:04pm.

So all it really means is that in your intertial rest frame it is the same time everywhere.

To take your exploding star example, if it is 2:37pm on August 18th 2021 when you see the explosion, then it is also 'now' the same time and date a billion light years away, and the star would have exploded a billion years earlier.

Answer 2

Now that I think about it, are all these clocks actually out of synch, but appear to be in synch by the time the light from all of them has reached the eyeballs of our observer?

The opposite is true. Here's how you synchronize two clocks at positions $\mathbf x_A$ and $\mathbf x_B$.

1. At time $t_1$ (as measured on clock $A$), a light pulse is emitted from the point $\mathbf x_A$ toward the point $\mathbf x_B$.
2. At time $t_2$ (as measured on clock $B$), the pulse is received at $\mathbf x_B$ and sent back toward $\mathbf x_A$.
3. At time $t_3$ (as measured on clock $A$), the reflected pulse arrives back at $\mathbf x_A$.

If $t_2 = (t_1+t_3)/2$, then we say the clocks at $A$ and $B$ are synchronized. Keeping this procedure in mind, it should be clear that an observer looking out at the lattice of clocks would see (with her eyes) the more distant clocks to be lagging because of the finite speed of light delay; however, she could compensate for this by mentally adding $d/c$ to each clock time (where $d$ is the distance to the clock) and if she did so, all of these naive time-of-flight discrepancies would disappear.

---

"In special relativity, the observer measures events against an infinite latticework of synchronized clocks." This sounds needlessly artificial and abstract.

The crucial point is that when we specify the time at which some event occurs, then that time should be understood as being measured on a clock at the location of the event. For example, when we say "a firecracker exploded at location $\mathbf x$ at 2:30 PM," what we mean is that a clock sitting at $\mathbf x$ read 2:30 PM when the firecracker exploded.

In Newtonian physics, we implicitly label events with a location and time, but that time is not localized to any particular position - we simply say that it is the time. As a result, we mathematically equate an observer's reference frame with a lattice of measuring sticks (against which one measures position) and a single clock which we could imagine sitting on the observer's wrist. In contrast, the perspective presented in special relativity is that we should make the idea of time a local one, by placing a clock at each lattice point.

Though it may seem artificial and unnecessary, the distinction is shown to be crucial by a straightforward consequence of the postulates of special relativity (specifically the constancy of the speed of light). Imagine two observers - each with their own synchronized lattice of clocks - at the same point, but with one moving relative to the other. The clocks at their respective coordinate origins will agree, but the more distant clocks will not.

More concretely, consider an observer who measures a firecracker exploding at position $\mathbf x = (10\ \mathrm m, 0, 0)$ at time $t_\mathbf x = 0$. For clarity, I mean that the light from the firecracker arrived at the origin $\mathcal O$ at time $t_\mathcal O = (10\ \mathrm m) / c$, and the appropriate time-of-flight was compensated for. Then - by straightforward consequence of the postulates of relativity - a second observer at the position as the first, and whose clock (at the origin) agrees with that of the first, but who is moving with velocity $\mathbf v = v\hat x$ relative to the first would measures the time at which the firecracker explodes to be $t'_\mathbf x=-\gamma vx/c^2$.

As a result, it is strictly necessary to understand time as a local phenomenon as a consequence of the postulates of special relativity. The time at which a distant event occurs is entirely dependent on the reference frame of the observer and as such is not a meaningful notion in the absence of further context; that is, the notion of a single, global time is simply incompatible with the constancy of the speed of light.

Answer 3

"In special relativity, the observer measures events against an infinite latticework of synchronized clocks." This sounds needlessly artificial and abstract.

It IS artificial and abstract, but it is far from needless. It serves a very good purpose to think of it along those lines.

" Therefore, one of these synchronized clocks mis-times the event. Do these clocks account for the time taken for the light from events to reach our eyeballs? "

No. The clocks do not account for time taken for light to reach eyeballs. Because, the clocks are not concerned with when the light from the event reached your eyeball. The clocks are concerned with when the event OCCURED , IN YOUR FRAME.

" In the exact same frame, I could have an observer 1 billion light years away right next to the star when it exploded registering a "more correct" time. "

In the same frame, all the clocks in the lattice would display the exact same time, when that event occurs. For an observer , 1 billion light years away, he would have his OWN lattice of clocks, and that lattice would also display some time on those clocks when that event happens, IN HIS FRAME. The time displayed on his clocks when the event happens in his frame, would NOT necessarily be the same as the time displayed on your lattice of clocks when the event happens in your frame.

" So the observers disagree on the timing of the events, even though they are in the same frame? Or does the far-away observer back-calculate the time of the event, knowing the distance to the star, and then they agree?"

Whether the observers clocks show the same time or not, depend on the specifics of the example. Specifically, whether their clocks were synced with each other some time in the past, and what were the sequence of " their motions " from the event of that syncing of clocks till now.

" are all these clocks actually out of synch, but appear to be in synch by the time the light from all of them has reached the eyeballs of our observer? "

No, in your frame, your lattice of clocks are always in sync. That is the premise of this system of infinite lattice of clocks. They are all in sync in your frame.

Answer 4

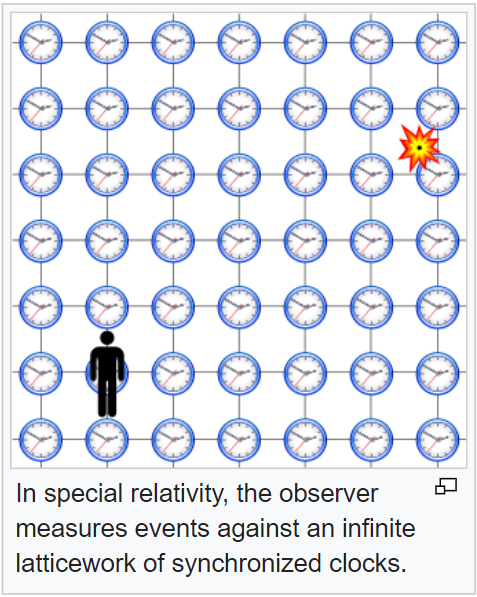
This question highlights an important point of confusion in many explanations of clock synchronization in frames of reference in special relativity: the sloppy use of the term "observer".

In actual fact, many explanations forget to explicitly highlight that an admissible special relativistic frame of reference, when imaginged as a lattice of clocks, not only has to synchronize its clocks but it also comes equipped with a little assistant at each synchronized clock, each assistant only recording the events that occur in the immediate vicinity of her clock by reading and co-recording the (ever changing) local time of her clock and the (unchanging) local coordinates of her lattice point at her home position when the event occurs.

The "observer" is a ficticious entity that has access to all the notebooks of all the assistants. In standard physics usage an "observer" in special relativity is, for all practical purposes, simply equivalent to a frame of reference and its coordinate system's encoding of events. This coordinate system in turn is best imagined as the lattice of clocks and their respective local assistants or recording devices for events. If one were to insist that the observer were indeed real and sitting at the origin then she would have to wait until the updates of all the assistants' new entries in their respective notebooks have arrived at the origin (and even when being sent by a radio wave travelling at the speed of light this may take some time...). In theoretical physics an observer in special relativity is thus not much more than a coordinate frame and its events, and in experimental physics you'd better automate all the assistants' work (local particle detectors) and then evaluate all the recorded events off-line (see e.g. experiments at CERN).

This same procedure of establishing a lattice, synchronizing clocks, and putting a recording device for local events (assistant) at the position of each lattice point and its corresponding clock is executed for all admissible frames of reference (by admissible I mean inertial systems in which a light beam may be considered to follow a straight line).

Thereby even statements about simultaneity in a single frame of reference already involve multiple (at least two) assistants at different positions recording in their respective notebooks their local time read from their respective local clock, and we mean by the assistants doing this at a single point in time that these assistants happen to write down the same numbers, e.g. 12:00 at their different locations into their different notebooks of events.

So, for example, when measuring the length of a "rigid" rod that is moving at a constant velocity in a frame of reference 1, then one has to understand that also in the frame of reference 2 that is comoving with the rod (i.e. in which the rod is not moving at all) one has two assistants: one at each end of the rod. Or to put it differently: all the assistants of the comoving frame are instructed to check if they are at the rear end or the front end when their respective clock shows 12:00. This is an instruction that does not require the assistants to travel. Only to check events in their vicinity. If they are at one of these locations when their clock reads 12:00, they should mark their coordinate lattice reading into their notebooks. Afterwards, these entries in the different notebooks must be evaluated: there will be one notebook noting "rear end at 12:00 at position P1=(x1,y1,z1)" and another notebook noting "front end at 12:00 at position P2=(x2,y2,z2)". All the other notebooks will have no entries since neither the rear end nor the front end happened to be at any other locations at 12:00. The "observer", or the master of all assistants, or simply the physicist using the coordinates of the comoving frame of reference, will then get the length of the rod by computing the Euclidean distance between the spatial locations P1 and P2 in frame 2. This gives the length of the rod at rest, i.e. the length in the co-moving frame 2. The same procedure applies of course in coordinate frame 1, in which the rod is moving. Only in this frame one uses the synchronized clocks of frame 1 and its lattice and its assistants. One imagines that such different frames of reference do not physically disturb each other. In frame 1, the assistants will perform the same task, they too will note at their pre-specified local times, e.g. 12:00, if they happen to be at the rear end or at the front end and they will record their positions Q1 and Q2 in the local notebooks in frame 1. It is most important to realise that when the synchronized clocks of frame 1 show the same time 12:00 the corresponding clocks of frame 2 at the same physical positions as their counterparts in frame 1 would not show the time of frame 1, and would not even show identical times in frame 2 either. Hence, from the point of view of the comoving frame 2, the positions of the rear end and the front end of the rod are recorded by assistants in frame 1 at different comoving times in frame 2, even though the clocks of frame 1 would all show 12:00 and the procedure in frame 1 is perfectly sound: record the positons of both ends at the same time in frame 1. This is the origin of length contraction in special relativity. It is important to understand that this length contraction result is not what anyone "sees" with their eyes but rather the result of the entries in the notebooks of the assistants in frames 1 and 2. This result is generic and applies also to the original question: a single person's visual experience of possibly remote events is not what is usually meant by observations made by an "observer" in special relativity (note that e.g. observations in astrophysics usually already involve general relativity, see also the brief remarks on clocks in general relativity below. In any case also for these observations we also need to imagine little local assistants throughout the universe and then compute from their imagined detector readings what an astrophysicist on earth should in the end actually "see" in her telescope, e.g. red-shifts, the measurement event here being the impinging of a propagating light ray on the telescope's imaging plane and the recording of this event by the local astrophysicist/assistant).

A similar argument applies to a moving clock and ticks on this clock. The clock would be moving by the lattice of clocks of frame 1. And when one tick has passed for the moving clock, the clock of frame 1 which happens to be next to the moving clock would not show the same time as the moving clock, even if the moving clock and another clock of frame 1 had shown the same time at the beginning of the experiment when these two clocks were co-located. This is the origin of time dilation. Again it is important to understand that this is the result of the entries of events in the notebooks of the assistants in frames 1 and 2.

This viewpoint of local assistants only recording their local events and an imagined "global observer" evaluating all recordings is especially useful when it comes to generalize to general relativity: all you have to imagine then is that the latticework is no longer rigid but rather turns into a freely floating cloud of tiny dust particles (or "reference-mollusc" as Einstein put it) with each dust particle carrying its local coordinates as three numbers written onto it, and a local clock with a local assistant and her local notebook to record only events occurring in the vicinity of the assistant's respective dust particle (and assuming that all these imagined infinitesimal dust particles do not modify the background metric, which is akin to assuming that a charged infinitesimal test particle would not influence the local electric field it is meant to measure). The clocks can no longer be synchronized globally in general relativity but that is not necessary to log local events in the local notebook together with a local point in time and a local spatial coordinate, i.e. the reading of the unsynchronized clock and three numbers written on the local dust particle when and where the event occurred. Again the "observer" is an imagined physicist evaluating all the logs in all the notebooks (NB1: strictly speaking the most general coordinate system in general relativity would not even have three constant spatial locations written on the dust particle but rather only three numbers that may also change over time, e.g. imagine four clocks on a dust particle, or alternatively a smartphone showing four continuously changing numbers. The only really important condition is that the four clocks are continuously changing times without ever showing equal four numbers for different events, e.g. for different dust particles, and usually the mathematical condition that the coordinates should be smoothly changing from particle to particle is also desirable. The readings of these four clocks, or, equivalently, the four changing numbers on the smartphone display of the local assistant serve to specify the coordinates of an event taking place at the dust particle and recorded by the local assistant or recording device. NB2: as an aside, the fact that a freely falling local observer "in an elevator" can also establish an extremely tiny, in both space and time localized latticework of rigid rods and synchronized clocks, just like in special relativity, allows for measuring the general relativistic metric by comparison of the local special relativistic coordinates with their corresponding dust particle coordinates)

For further reading along these lines (assistants recording events in notebooks) I would recommend:

(1) "The Geometry of Minkowski Spacetime" by Gregory L. Naber, 2012, Springer, in particular the Introduction.

(2) "The Einstein Theory of Relativity: A Trip to the Fourth Dimension", by Lillian Lieber, Paul Dry Books, 2008, for an extremely easy introduction to both special and general relativity.

(3) "General Relativity from A to B" by Robert Geroch, The University of Chicago Press, 1978, for an equally easy introduction to general relativity.

(4) "Relativity: The Special and the General Theory", Albert Einstein, annotated 100th Anniversary Edition by Princeton University Press, 2019, discussing in chapter 28 the "reference-mollusc" as a pedagogical aid invented by the master himself.

(5) https://en.wikipedia.org/wiki/Length_contraction#Visual_effects for further references explaining the difference between what a single person would "see" and what is meant by measuring a length contraction.

Answer 5

"In special relativity, the observer measures events against an infinite latticework of synchronized clocks."

A more precise statement is that in special relativity, spacetime is modeled as if there were an infinite latticework of clocks. The clocks don't actually exist, and also the term "synchronized" is problematic. Synchronicity is defined in terms of these imaginary clocks, so it's a bit circular to assert that they are synchronous.

Say the event is a star exploding which is 1 billion light years away. The light reaches my eyeballs 1 billion years after the event happened. Therefore, one of these synchronized clocks mis-times the event.

In relativity, "event" refers to a point in four-dimensional spacetime. The idea with the synchronous clocks is that the time of an event is defined by the time shown by the clock at that event. The star exploding, and the light from the star's explosion reaching your eyeballs, are two different events. The clock next to the star correctly times the explosion, and the clock next to your eyeballs correctly times the light reaching you.

Compare it a video that has an on-screen display of the time. This is a record of the time when that moment of the video was recorded. When you actually watch it, the time will be different from the current time, but that doesn't mean the clock on the video mistimed the events. It correctly recorded the time at the time of the events. Similarly, if there were a lattice of clocks, then when you see the star exploding, you would see a clock next to it, and that clock would correctly record the time of the explosion.

Or does the far-away observer back-calculate the time of the event, knowing the distance to the star, and then they agree?

If the clocks were actually there, we would not have to back-calculate the time. We could just look at the clock and see what time the event was. But since we don't have the clocks, we calculate what the clock would say if it were there.

Now that I think about it, are all these clocks actually out of synch, but appear to be in synch by the time the light from all of them has reached the eyeballs of our observer?

There is no objective basis for whether the clocks are in synch or not. The clocks are simply a mental model for the time coordinate. We assign each event a time coordinate, and then we imagine a clock there showing that time coordinate. Someone in a different reference frame would have different times on their imaginary clocks. We could have a coordinate system such that by time the light reaches us, they are showing the same time as a clock next to us. That is an anisotropic coordinate system, and it violates the Copernican principle, but it is a valid coordinate system.

Answer 6

The simple answer is this:

1. If the observers are in the SAME frame of reference, then they will see the event at the exact SAME time (even when they are a billion light years apart).
2. If the observers are in a DIFFERENT frame of reference, then they will see the event at DIFFERENT times.

Notes:

• This is a thought experiment. In reality it is nearly impossible to be a billion light years apart and still be in the same frame of reference because galaxies are constantly moving.
• The universe is expanding, which means that the same frame of reference at these large scales are not possible.

Answer 7

Rather than explaining what it means, I will try to explain how the clocks are synchronized.

Initially all clocks are not synchronized.

Let us say one of the clock produces a flash. This flash indicates t = 0. As soon as flash is produced, this clock sets its time as zero.

Let us say the next it takes t = 1 second for light to reach next clock. As soon as next clock sees the flash, it sets its time as t = 1 second. Now it is synchronized with original clock taking into account for the time taken for the light to travel.

Soon all clocks are synchronized!

When as event occurs, we measure time using clock nearest to it. This clock issues a "time tag" for the event. Everyone considers this "time tag" as the time of event.

You asked,

Do these clocks account for the time taken for the light from events to reach our eyeballs?

Yes. You are correct. That could be one way of thinking about it. All clock measure time of event by subtracting time of journey of light. But "lattice of clock" approach is more elegant way of thinking about it!