Consider the Sturm-Liouville regular problem in self-adjoint form
$$(A-\zeta)\,v=f$$
whose explicit solution is
$$v=\int_a^bG(x,s)\,f(s)\,ds$$
where the Green function
$$G(x,s)=\begin{cases}\frac{\varphi_b(x)\,\varphi_a(s)}{W(s)},&a\leq{s}\leq{x}\\[0.1in]\frac{\varphi_a(x)\,\varphi_b(s)}{W(s)},&x\leq{s}\leq{b}\end{cases}$$
is built by the solutions $\varphi_a$, $\varphi_b$ of the equation $A\,\varphi=0$ which satisfy respective boundary conditions at $x=a,b$. It's a long way to get here, but you can verify this. The resolvent associated to $A$ is given by
$$R(A,\zeta)\,f=\int_a^bG(x,s)\,f(s)\,ds$$
Now, showing that an operator $K$, whose kernel $k(x,s)$ satisfies
$$\int_a^b\int_a^b\left|k(x,s)\right|^2ds\,dx<\infty\hspace{0.5in}(*)$$
is compact (meaning the functions $k$ are of square-integrable), may be used to show that if the Green function $G$ of the resolvent operator $R$ is continuous, then $G$ satisfies equation $(*)$ on a finite interval $[a,b]$, meaning that $R$ is a compact self-adjoint operator for regular Sturm-Liouville problems. You could just do this last thing in general, and just show that with the operator
$$\hat{H}\equiv{T}=-\frac{\hbar^2}{2m}\nabla^2+V$$
(the Hamiltonian), the eigenvalue problem $\hat{H}\psi=E\psi$ (known as Schrödinger stationary -or time independent- equation) can be reduced to a regular Sturm-Liouville problem.
All of this because, complementing what you said, the eigenvalues of the problem $T\varphi_k=\lambda_k\varphi_k$ for a compact symmetric operator $T$ on a Hilbert space $H$ with interior product $\langle\cdot\mid\cdot\rangle_H$ are a bounded countably infinite set that converges to zero, $\displaystyle{\lim_{k\to\infty}\lambda_k=0}$. Other (pretty relevant) properties are that the multiplicity of each eigenvalue $\lambda_k$ is finite, and that the set of all eigenfunctions $\varphi_k$ define a complete basis of the space $H$, so that any element $f$ of $H$ can be expanded as $\displaystyle{f=\sum_{k=1}^\infty{f_k}\,\varphi_k}$.
Update
Please note the relevance of the condition $\boxed{\displaystyle{E<\lim_{|x|\rightarrow \infty}V(x)}}$. Consider this next image as an illustration (I've taken it directly from the web, so take the potential $V=U$); as the math is pretty straightforward, I'll go a little more with physical picture this time
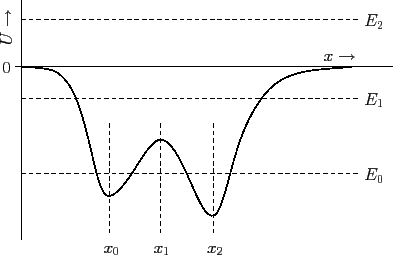
with the condition above, the results found are valid for the equation $\hat{H}\psi=E_i\psi$ with $i=0,1$. Indeed, both equations may presumably be reduced to a regular S-L problem because they would be ones with a finite or penetrable potential well, where we can know the energy spectrum just from $\psi$ inside the potential well, i.e. for a finite interval, whereas considering $i=2$ the interval for $\psi$ would inevitably be infinite and it is evident that $\hat{H}\psi=E_i\psi$ couldn't be reduced to a regular S-L problem and thus the energy spectrum presumably wouldn't be discrete. Feel free to consider any potential you like.
This corresponds to a beautiful analogy with classical mechanics when we find closed or open orbits, which here are bounded and unbounded states (hope I got the translations right) respectively. Think of a massive nucleus and an electron that interact by means of an atractive Columb potential. If the condition above is satisfied, the system may be a hydrogen-type atom that would have a discrete energy spectrum, as known. Although if someone shot the electron against the nucleus from very far away and with enough kinetic energy, the total energy would be positive and the nucleus would deflect the electron without capturing it and without changing it's energy, which evidently is free to take any value. The simplest case for a continuous energy spectrum in QM is that of the free particle, i.e. $V(\mathbf{r})=0,\,\forall\mathbf{r}\in\mathbb{R}^3$; there the normalization condition $\int\psi^*_n\psi_m\,dx=\delta_{nm}$ is not valid and may be generalized using the Dirac delta, also wavefunctions strictly belong to a Banach space.

